A uniform disc of mass M and radius R is supported vertically by a pivot at its periphery as shown. A particle of mass M is fixed to the rim and raised to the highest point above the center. The system is released from rest and it can rotate about pivot freely. The angular speed of the system when the attached object is directly beneath the pivot, is
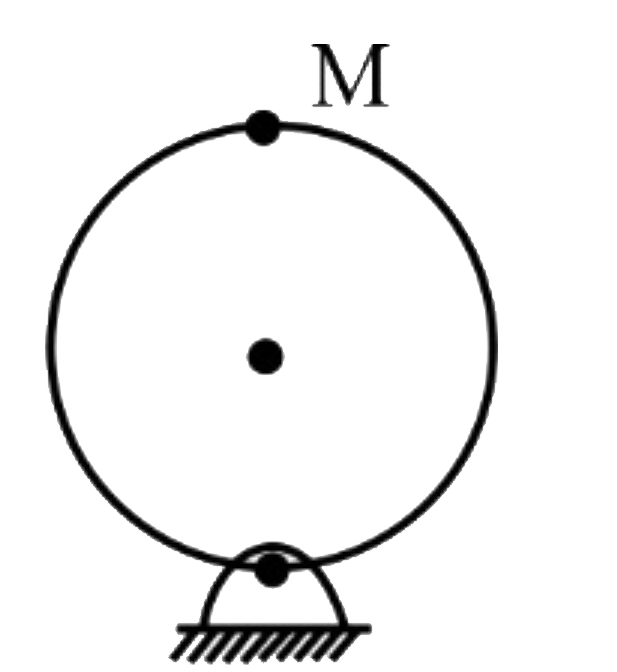
√24g11R
√8g11R
√8g3R
√14g3R
The correct Answer is:A
Step by step video, text & image solution for A uniform disc of mass M and radius R is supported vertically by a pivot at its periphery as shown. A particle of mass M is fixed to the rim and raised to the highest point above the center. The system is released from rest and it can rotate about pivot freely. The angular speed of the system when the attached object is directly beneath the pivot, is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions

A uniform disc of mass M and radius R is pivoted about the horizontal axis through its centre C A point mass m is glued to the disc at its rim, as shown in figure. If the system is released from rest, find the angular velocity of the disc when m reaches the bottom point B.View SolutionA uniform disc of radius R and mass M is free to rotate only about its axis. A string is wrapped over its rim and a body of mass m is tied to the free end of the string as shown in the figure. The body is released from rest. Then the acceleration of the body is
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A uniform disc of mass M object of mass M is attached to the rim and raised to the highest point above the centre. The unstable system is then released. The angular speed of the system when the attached object passes directly beneath the pivot is.
 A√3R8RB√3g8RC√8R3gD√8g3R
A√3R8RB√3g8RC√8R3gD√8g3R - Question 2 - Select One
A uniform rod of length l is from rest such that it rotates about a smooth pivot. The angular speed of the rod when it becomes vertical is.
 .A2√6g7lB√6g7lC2√3g7lD√3g7l
.A2√6g7lB√6g7lC2√3g7lD√3g7l - Question 3 - Select One
A particle of mass 'm' is rigidly attached at 'A' to a ring of mass 3m and radius 'r' . The system is released from rest and rolls without sliding. The angular release is acceleration of ring just after release after
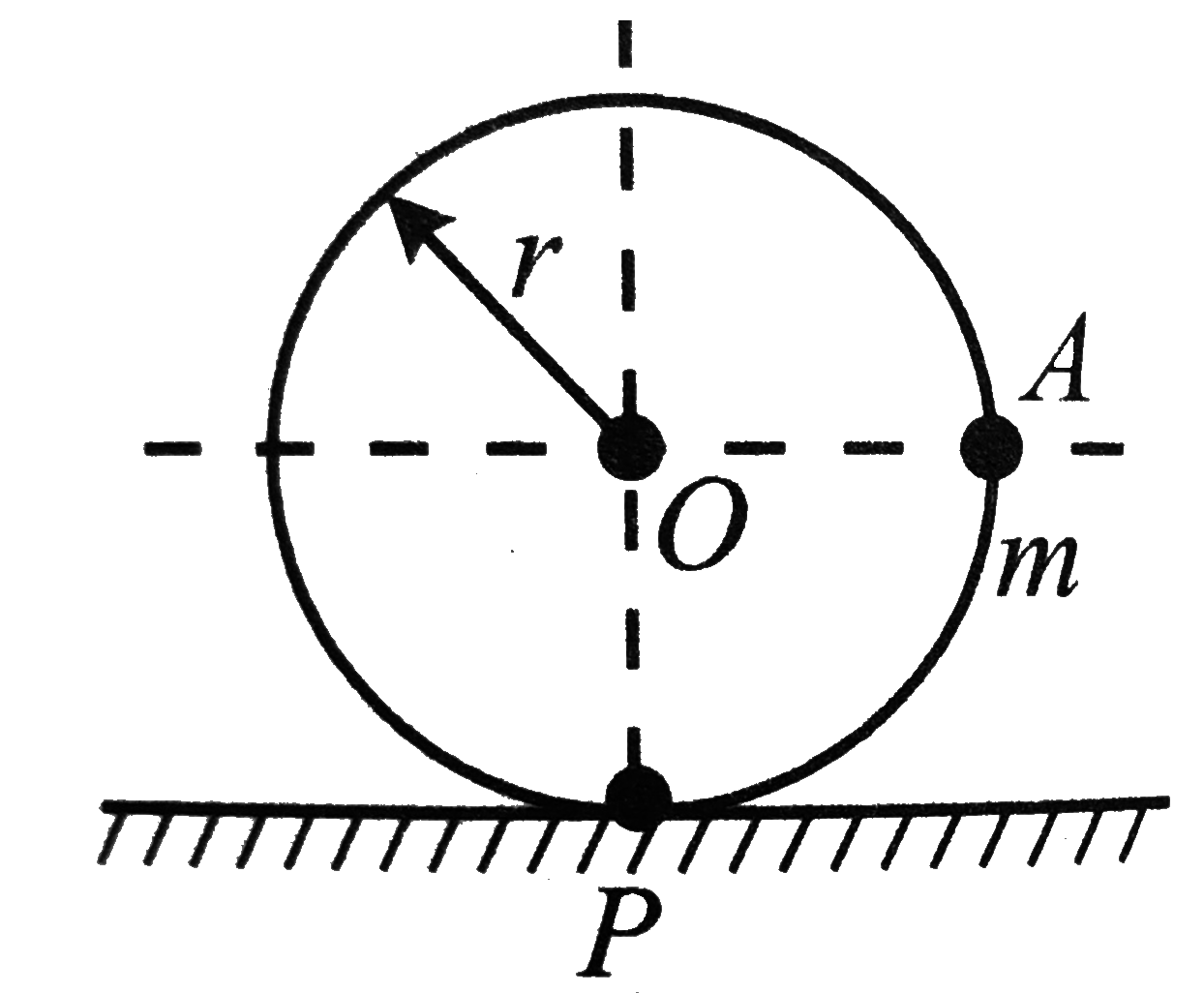 Ag4rBg6rCg8rDg2r
Ag4rBg6rCg8rDg2r
A disc of mass m and radius r is free to rotate about its centre as shown in the figure. A string is wrapped over its rim and a block of mass m is attached to the free end of the string. The system is released from rest. The speed of the block as it descends through a height h, is :-
 View Solution
View SolutionA uniform disc of mas M and radius R is smoothly pivoted at O. A light inextensible string wrapped over the disc hangs a particle of mass m. If the system is released from rest, assuming that the string does not slide on the disc, find the angular speed of the disc as the function of time using impulse momentum equation.
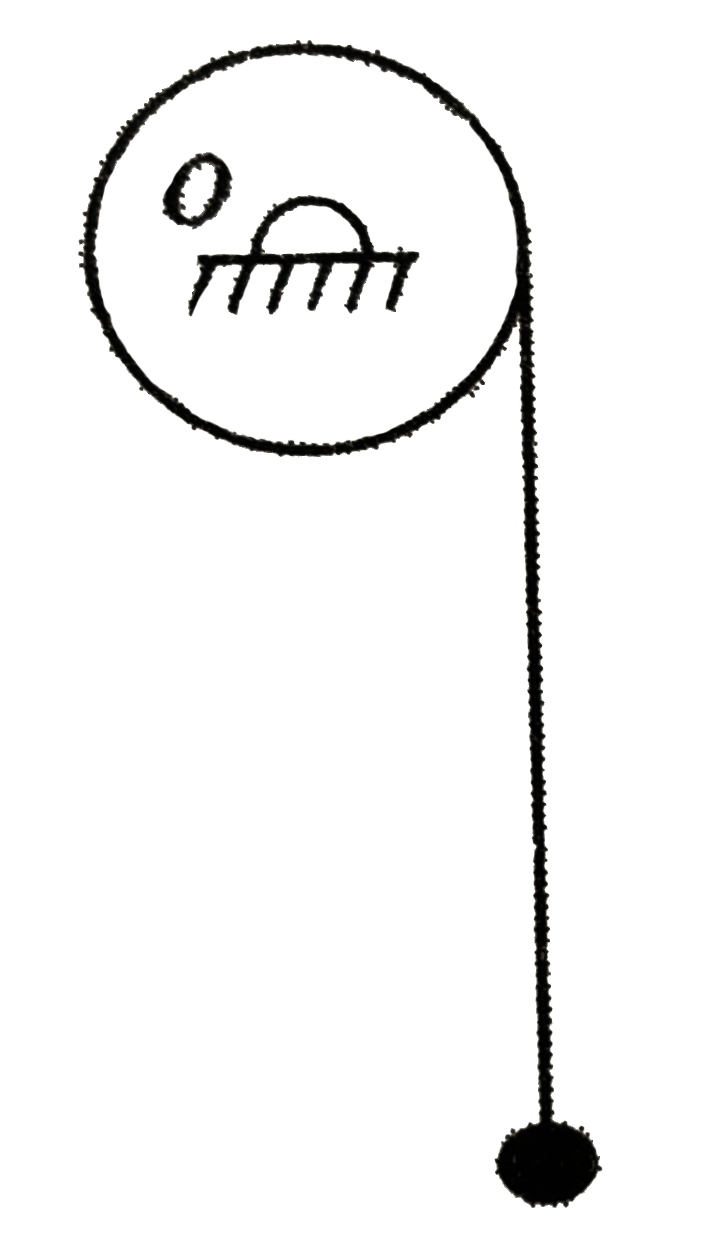 View Solution
View SolutionA uniform disc of mass M and radius R is pivoted about a horizontal axis passing through its edge. It is released from rest with its centre of mass at the same height as the pivot.
 View Solution
View SolutionThere is a ring or mass m and radius R is pivoted at a point O on its periphery. It is free to rotate about an axis perpendicular to its plane. What is the period of ring?
View SolutionA solid unifrom disk of mass m and radius R is pivoted about a horizontal axis through its centre and a small body of mass m is attached to the rim of the disk. If the disk is released from rest with the small body, initially at the same level as the centre, the angular velocity when the small body reaches the lowest point of the disk is.
View Solution

