Question

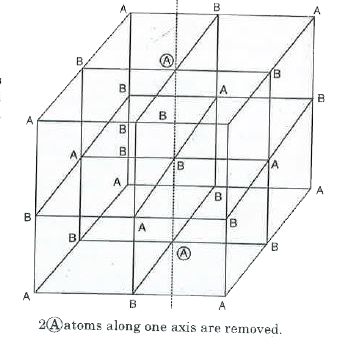
In a solid 'AB' having rock salt arrangement ($NaCl$ structure), 'A' atoms occupy the corners and face center of the cubic unit cell. 'B' atoms occupies all octahedral voids of the unit cell. If all the face - centered atoms along one of the axes are removed, then the resultant stoichiometry of the solid is

Options

A. `AB_(2)`
B. `A_(2)B`
C. `A_(4)B_(3)`
D. `A_(3)B_(4)`

Answer

D

Explanation

To solve the problem, we need to analyze the arrangement of atoms in the solid 'AB' with a rock salt (NaCl) structure and determine the resultant stoichiometry after removing certain atoms. Here’s a step-by-step breakdown of the solution:

Step 1: Determine the number of A atoms in the unit cell
In a rock salt structure:
- A atoms occupy the corners and face centers of the cubic unit cell.
- There are 8 corners in a cube, and each corner contributes \( \frac{1}{8} \) of an atom to the unit cell.
- There are 6 faces, and each face contributes \( \frac{1}{2} \) of an atom to the unit cell.

Calculation:
- Contribution from corners:
\( 8 \text{ corners} \times \frac{1}{8} = 1 \text{ A atom} \)
- Contribution from face centers:
\( 6 \text{ faces} \times \frac{1}{2} = 3 \text{ A atoms} \)
- Total A atoms:
\( 1 + 3 = 4 \text{ A atoms} \)

Step 2: Determine the number of B atoms in the unit cell
- B atoms occupy all octahedral voids in the unit cell.
- In a cubic unit cell, there are 4 octahedral voids.

Conclusion:
- Total B atoms:
\( 4 \text{ B atoms} \)

Step 3: Initial stoichiometry of the compound
From the above calculations:
- A = 4
- B = 4

Thus, the initial stoichiometry of the compound is \( AB \) (or \( A_4B_4 \)).

Step 4: Remove face-centered A atoms along one axis
According to the problem, we need to remove all face-centered A atoms along one axis.
- There are 2 face-centered A atoms along the chosen axis (one from each of the two faces).

Contribution of removed atoms:
- Each face-centered atom contributes \( \frac{1}{2} \).
- Therefore, the total contribution of the removed atoms is:
\( 2 \text{ atoms} \times \frac{1}{2} = 1 \text{ A atom} \)

Step 5: Calculate the new number of A atoms
- Initial number of A atoms = 4
- Removed A atoms = 1

New number of A atoms:
\( 4 - 1 = 3 \text{ A atoms} \)

Step 6: Final stoichiometry of the compound
- After the removal of face-centered A atoms:
- A = 3
- B = 4

Thus, the resultant stoichiometry of the solid is \( A_3B_4 \).

Final Answer:
The resultant stoichiometry of the solid is \( A_3B_4 \).

---