Find the work done by the frictional force in drawing a circle of radius r by a pencil of negligible mass with a normal pressing force N ( coefficient of friction μk).
To find the work done by the frictional force in drawing a circle of radius r by a pencil with a normal pressing force N and a coefficient of friction μk, we can follow these steps:
1. Understanding the Setup:
- We have a pencil (considered to have negligible mass) that is drawing a circle of radius r.
- The normal force acting on the pencil is N.
- The coefficient of kinetic friction between the pencil and the surface is μk.
2. Identifying the Frictional Force:
- The frictional force fk can be calculated using the formula:
fk=μk⋅N
3. Calculating the Work Done:
- The work done dW by the frictional force when the pencil moves through an infinitesimal displacement dl is given by:
dW=−fk⋅dl
- The negative sign indicates that the frictional force opposes the direction of displacement.
4. Integrating Over the Path:
- To find the total work done W as the pencil completes one full circle, we need to integrate dW over the entire length of the path, which is the circumference of the circle:
W=∫dW=∫−fk⋅dl
- The total length L of the circle is given by:
L=2πr
- Substituting fk into the integral:
W=∫2πr0−(μk⋅N)⋅dl
- Since μk and N are constants, we can take them out of the integral:
W=−μk⋅N⋅∫2πr0dl
5. Evaluating the Integral:
- The integral of dl from 0 to 2πr is simply 2πr:
W=−μk⋅N⋅(2πr)
6. Final Result:
- Therefore, the total work done by the frictional force in drawing the circle is:
W=−2πrμkN
- We have a pencil (considered to have negligible mass) that is drawing a circle of radius r.
- The normal force acting on the pencil is N.
- The coefficient of kinetic friction between the pencil and the surface is μk.
- The frictional force fk can be calculated using the formula:
fk=μk⋅N
- The work done dW by the frictional force when the pencil moves through an infinitesimal displacement dl is given by:
dW=−fk⋅dl
- The negative sign indicates that the frictional force opposes the direction of displacement.
- To find the total work done W as the pencil completes one full circle, we need to integrate dW over the entire length of the path, which is the circumference of the circle:
W=∫dW=∫−fk⋅dl
- The total length L of the circle is given by:
L=2πr
- Substituting fk into the integral:
W=∫2πr0−(μk⋅N)⋅dl
- Since μk and N are constants, we can take them out of the integral:
W=−μk⋅N⋅∫2πr0dl
- The integral of dl from 0 to 2πr is simply 2πr:
W=−μk⋅N⋅(2πr)
- Therefore, the total work done by the frictional force in drawing the circle is:
W=−2πrμkN
Topper's Solved these Questions
- BOOK - FIITJEECHAPTER - WORK, ENERGY AND POWER EXERCISE - SOLVED PROBLEMS (SUBJECTIVE )8videos
WORK, ENERGY AND POWER
- BOOK - FIITJEECHAPTER - WORK, ENERGY AND POWER EXERCISE - SOLVED PROBLEMS ( OBJECTIVE)18videos
WORK, ENERGY AND POWER
- BOOK - FIITJEECHAPTER - TEST PAPERSEXERCISE - PHYSICS747videos
TEST PAPERS
Similar Questions
Knowledge Check
- Question 1 - Select One
Work done by a frictional force is
AnegativeBpositiveCzeroDall of the above - Question 2 - Select One
Work done by frictional force
AIs always negativeBIs always positiveCIs zeroDMay be positive, negative or zero - Question 3 - Select One
Work done by the force of friction
Acan be zeroBcan be positiveCcan be negativeDany of the above
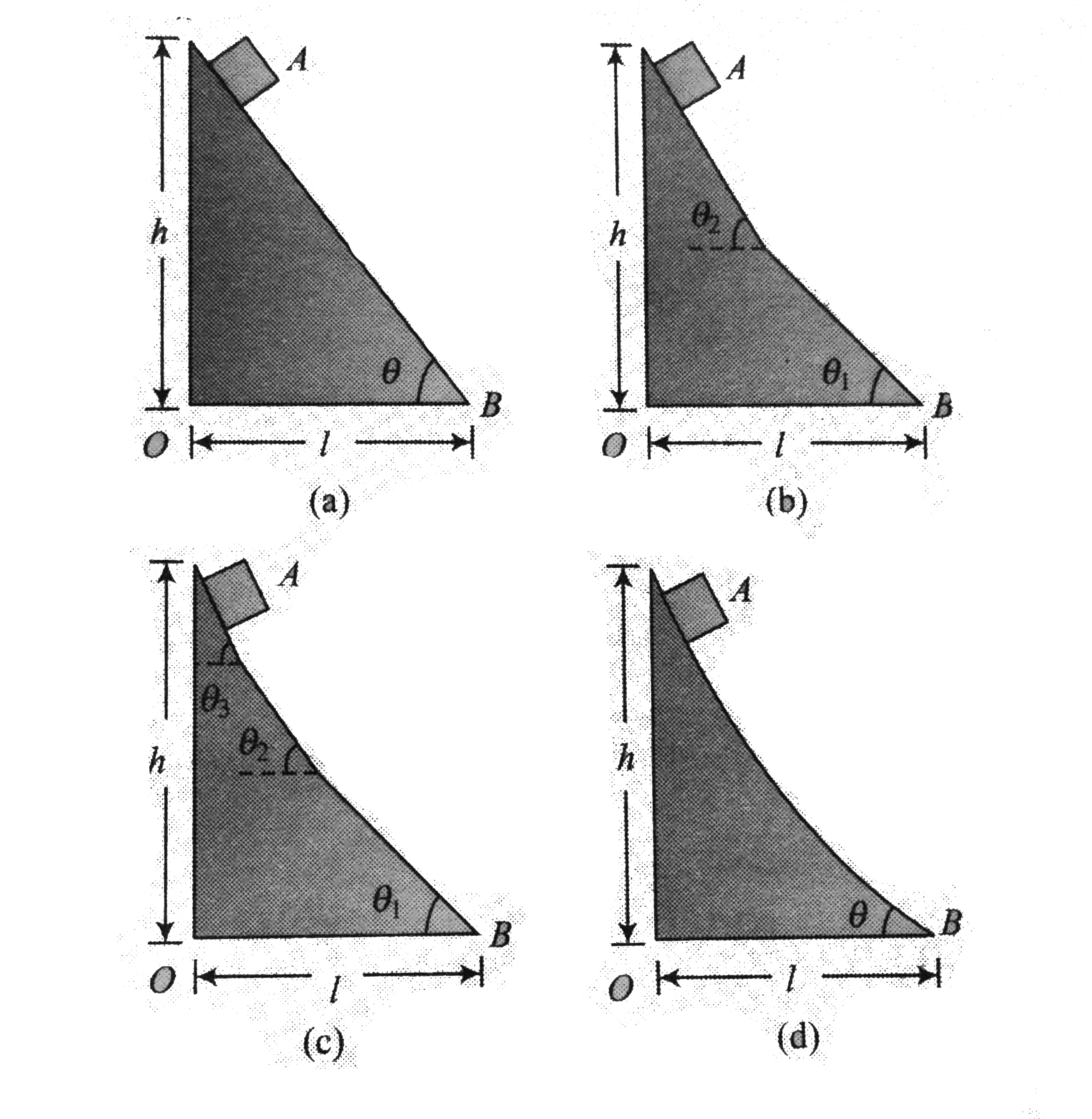
Block of mass m are released from rest and they slide down the inclined surface as shown in figure. Show that the work done by the frictional forces on the blocks are same in all the cases. Also calculate the speeds with which the blocks reach point B. The coefficient of friction for all the surface is μk.
 View Solution
View SolutionIf the normal force is double, then coefficient of friction is,
View Solutionthe work done against force of friction is
View SolutionWork done by force of static friction .
View SolutionIf normal force becomes two times, then coefficient of friction
View Solution

