Considering a body of mass m, radius R rotating with angular speed ω about the centre of the mass and with a velocity v of centre of mass, the most appropriate definition of rolling motion will be
v = R ω
the point of contact is stationary
the point of contact is stationary with respect to the surface
the body purely rotates about the point of contact
The correct Answer is:C
To solve the question regarding the definition of rolling motion for a body of mass m, radius R, rotating with angular speed ω and having a linear velocity v of the center of mass, we can follow these steps:
1. Understanding Rolling Motion:
- Rolling motion occurs when a body rotates about its axis while simultaneously translating along a surface. For pure rolling without slipping, the point of contact with the surface must have zero velocity relative to the surface.
2. Identify Key Points:
- Let’s denote:
- v: Linear velocity of the center of mass.
- R: Radius of the body.
- ω: Angular velocity of the body.
- Point P: The point of contact between the body and the surface.
3. Velocity at the Point of Contact:
- The linear velocity of the center of mass is v.
- The tangential velocity at the point of contact due to rotation is given by Rω.
4. Condition for Pure Rolling:
- For pure rolling, the point of contact must be stationary with respect to the surface. This means that the net velocity at point P must be zero:
v−Rω=0
- Rearranging this gives:
v=Rω
5. Conclusion:
- The most appropriate definition of rolling motion is that the point of contact is stationary with respect to the surface. This implies that the linear velocity v of the center of mass is equal to the tangential velocity Rω at the point of contact.
Final Answer:
The most appropriate definition of rolling motion is that the point of contact is stationary with respect to the surface.
- Rolling motion occurs when a body rotates about its axis while simultaneously translating along a surface. For pure rolling without slipping, the point of contact with the surface must have zero velocity relative to the surface.
- Let’s denote:
- v: Linear velocity of the center of mass.
- R: Radius of the body.
- ω: Angular velocity of the body.
- Point P: The point of contact between the body and the surface.
- The linear velocity of the center of mass is v.
- The tangential velocity at the point of contact due to rotation is given by Rω.
- For pure rolling, the point of contact must be stationary with respect to the surface. This means that the net velocity at point P must be zero:
v−Rω=0
- Rearranging this gives:
v=Rω
- The most appropriate definition of rolling motion is that the point of contact is stationary with respect to the surface. This implies that the linear velocity v of the center of mass is equal to the tangential velocity Rω at the point of contact.
The most appropriate definition of rolling motion is that the point of contact is stationary with respect to the surface.
Topper's Solved these Questions
- BOOK - FIITJEECHAPTER - ROTATIONAL MECHANICSEXERCISE - ASSIGNMENT PROBLEMS (OBJECTIVE ) LEVEL - II16videos
ROTATIONAL MECHANICS
- BOOK - FIITJEECHAPTER - ROTATIONAL MECHANICSEXERCISE - COMPREHENSION - III1videos
ROTATIONAL MECHANICS
- BOOK - FIITJEECHAPTER - ROTATIONAL MECHANICSEXERCISE - ASSIGNMENT PROBLEMS (SUBJECTIVE ) LEVEL - II & III15videos
ROTATIONAL MECHANICS
- BOOK - FIITJEECHAPTER - PHYSICS PART2EXERCISE - Numerical Based Question Decimal Type6videos
PHYSICS PART2
- BOOK - FIITJEECHAPTER - SEMICONDUCTOR AND DEVICEEXERCISE - SOLVED PROBLEMS Objective: Level-I20videos
SEMICONDUCTOR AND DEVICE
Similar Questions
A disc of mass M and Radius R is rolling with an angular speed ω on the horizontal plane. The magnitude of angular momentum of the disc about origin is:
 View Solution
View SolutionA disc of mass M and radius R is rolling with angular speed w on horizontal plane as shown. The magnitude of angular momentum of the disc about the origin O is :
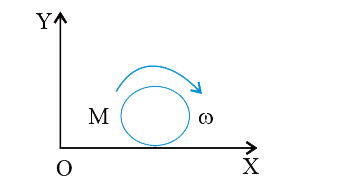 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
If a body of mass 'm' and radius of gyration K rotates with angular velocity ω, then the angular momentum of the body will be
Am2kωBmk2ωCmkω2Dmkω - Question 2 - Select One
A solid cylinder of mass M and radius R rotates about its axis with angular speed ω. Its rotational kinetic energy is
A12MR2ω2BMR2ω2C14MR2ω2D18MR2ω2 - Question 3 - Select One
A disc of mass M and radius R is rolling with angular speed ω on a horizontal plane. The magnitude of angular momentum of the disc about a point on ground along the line of motion of disc is :
A(1/2)MR2ωBMR2ωC(3/2)MR2ωD2MR2ω
A disc of mass m, radius r and carrying charge q, is rotating with angular speed ω about an axis passing through its centre and perpendicular to its plane. Calculate its magnetic moment
View SolutionIf V is velocity of centre of mass of a rolling body then velocity of lowest point of that body is
View SolutionA rigid body of mass m rotates about an axis passing through its centre. If the angular velocity of the body is unity, the moment of inertia of the body will be equal to
View SolutionA rigid body of mass m rotates about an axis passing through its centre. If the angular velocity of the body is unity, the moment of inertia of the body will be equal to
View SolutionA body of mass m and radius r is rotated with angular velocity ω as shown in the figure & kept on a surface that has sufficient friction then the body will move
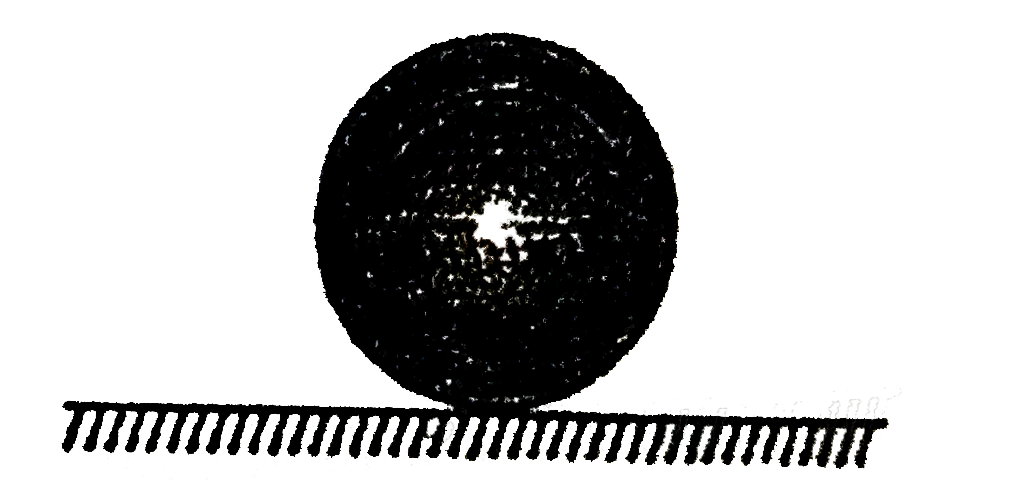 View Solution
View Solution

