Question

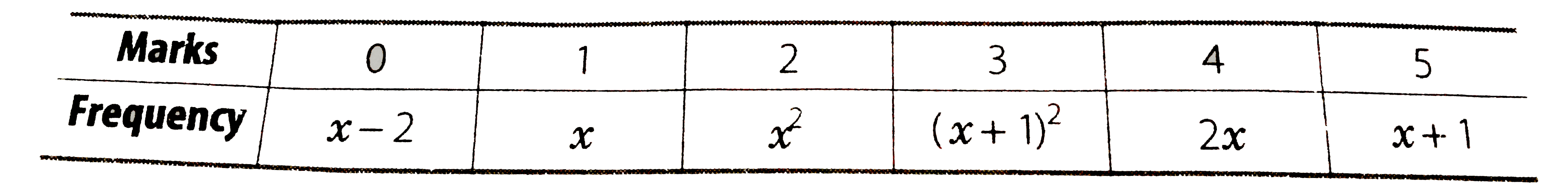
For a frequency distribution lower quartile is computed by

Options

A. `Q_(1)=l+(N/4-C)/(f) xxi`
B. `Q_(1)=l+((N/2)-C)/(f)xx i`
C. `Q_(1)=l+((3N)/(4)-C))/(f) xx i`
D. `Q_(1)=l+((N-C))/(f) xx i`

Answer

A

Explanation

To compute the lower quartile (Q1) for a frequency distribution, we follow these steps:

1. Identify the Frequency Distribution:
- A frequency distribution consists of values (or class intervals) and their corresponding frequencies. For example, if we have a set of data, we can group it into intervals and count how many data points fall into each interval.

2. Determine the Total Frequency (N):
- Calculate the total number of observations (N) by summing up all the frequencies.

3. Calculate N/4:
- To find the lower quartile, compute \( N/4 \). This value indicates the position of the lower quartile in the ordered data.

4. Find the Cumulative Frequency:
- Create a cumulative frequency table by adding the frequencies cumulatively. This helps in identifying which class interval contains the lower quartile.

5. Locate the Class Interval for Q1:
- Identify the class interval where the cumulative frequency is greater than or equal to \( N/4 \). This is the class interval that contains the lower quartile.

6. Determine the Lower Bound (LB) of the Q1 Class:
- The lower bound of the class interval identified in the previous step is denoted as LB.

7. Find the Frequency (F) of the Q1 Class:
- Note the frequency (F) of the class interval that contains Q1.

8. Calculate the Cumulative Frequency (C) of the Class Before Q1:
- Find the cumulative frequency (C) of the class interval that is immediately before the Q1 class.

9. Determine the Width of the Class Interval (I):
- Calculate the width of the class interval (I). This is the difference between the upper and lower limits of the class interval.

10. Use the Formula for Q1:
- Finally, apply the formula for the lower quartile:
\( Q1 = LB + \left(\frac{N}{4} - C\right) \times \frac{I}{F} \)
- Here, LB is the lower bound of the Q1 class, N is the total frequency, C is the cumulative frequency of the class before Q1, F is the frequency of the Q1 class, and I is the width of the class interval.