The rate expression for the reaction A(g) + B(g) → C(g) is rate = KC2AC½B. What changes in the initial concentration of A and B will cause the rate of reaction increase by a factor of eight?
CA×2,CB×2
CA×2,CB×4
CA×1,CB×4
CA×4,CB×1
The correct Answer is:B
To determine the changes in the initial concentrations of A and B that will cause the rate of the reaction A(g)+B(g)→C(g) to increase by a factor of eight, we start with the given rate expression:
Rate=k(CA)2(CB)1/2
We want to find new concentrations (C′A) and (C′B) such that:
New Rate=8×Rate
This means:
k(C′A)2(C′B)1/2=8×k(CA)2(CB)1/2
We can simplify this by canceling k from both sides:
(C′A)2(C′B)1/2=8(CA)2(CB)1/2
Next, we can express (C′A) and (C′B) in terms of the original concentrations (CA) and (CB):
Let:
(C′A)=x(CA)
(C′B)=y(CB)
Substituting these into the equation gives:
(x(CA))2(y(CB))1/2=8(CA)2(CB)1/2
Expanding this, we get:
x2(CA)2y(CB)1/2=8(CA)2(CB)1/2
Now, we can cancel (CA)2 and (CB)1/2 from both sides (assuming they are not zero):
x2y=8
Now, we need to find suitable values for x and y that satisfy this equation.
Possible Combinations:
1. Option 1: Let x=2 and y=2:
(2)2(2)=4×2=8(Valid)
2. Option 2: Let x=2 and y=4:
(2)2(4)=4×4=16(Not Valid)
3. Option 3: Let x=1 and y=8:
(1)2(8)=1×8=8(Valid)
4. Option 4: Let x=4 and y=1:
(4)2(1)=16×1=16(Not Valid)
Conclusion:
The valid combinations that will increase the rate by a factor of eight are:
- Doubling the concentration of A and doubling the concentration of B: (C′A)=2(CA) and (C′B)=2(CB).
- Keeping the concentration of A the same and increasing the concentration of B by a factor of 8: (C′A)=(CA) and (C′B)=8(CB).
(C′A)=x(CA)
(C′B)=y(CB)
(2)2(2)=4×2=8(Valid)
(2)2(4)=4×4=16(Not Valid)
(1)2(8)=1×8=8(Valid)
(4)2(1)=16×1=16(Not Valid)
- Doubling the concentration of A and doubling the concentration of B: (C′A)=2(CA) and (C′B)=2(CB).
- Keeping the concentration of A the same and increasing the concentration of B by a factor of 8: (C′A)=(CA) and (C′B)=8(CB).
Similar Questions
The experimental rate law for a reaction
2A+2B→ Product, is
V∝CAC1/2B. If the concentration of both A and B are doubled the rate of reaction increases by a factor ofView SolutionFor a reaction A+B→C , the rate law is written as r=k[A]2[B] . Doubling the concentrations of both of 'A' and 'B' increases the rate of reaction by
View Solution
Knowledge Check
- Question 1 - Select One
The rate expression for the reaction, A(g)+B(g)→C(g), is rate = k[A]2[B]1/2.
What changes in the initial concentrations of A and B will cause the rate of reaction to increase by a factor of eight?A[A]×2,[B]×2B[A]×2,[B]×4C[A]×1,[B]×4D[A]×4,[B]×1 - Question 2 - Select One
The rate expression for the reaction A(g)+B(g)→C(g) is rate=k[A]2[B]1/2. What changes in the initial concentrations of A and B will cause the rate of reaction to increase by a factor of eight?
A[A]=[A]:[B] = 2[B]B[A]=2[A]:[B] = 4[B]C[A]=[A]:[B] = 4[B]D[A]=4[A]:[B] = [B] - Question 3 - Select One
The rate expression for reaction A(g)+B(g)→C(g) is rate =k[A]1/2[B]2. What change in rate if initial concentration of A and B increases by factor 4 and 2 respectively ?
A4B6C8DNone of these
In a reaction A →B, the rate of reaction increases eight times on increasing the concentration of the reactant four times, then order of reaction is:
View SolutionFor a hypothetical reaction, A+B→C+D, the rate =k[A]−1/2[B]3/2. On doubling the concentration of A and B the rate will be
View SolutionIn a reaction between A and B, the initial rate of reaction was measured for different initial concentration A and B as:
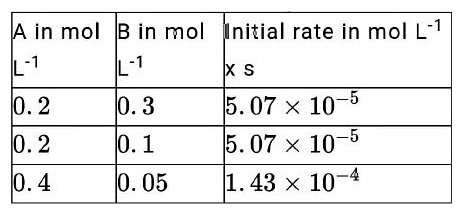
Find the order of reaction with respect to A and B.View SolutionFor a reaction A+B→C, the rate law is written as r=k[A]2[B] . Doubling the concentration of 'A' without changing concentration of 'B' increases the rate of reaction by
View SolutionFor the reaction A+B products, it is observed that:
(1) on doubling the initial concentration of A only, the rate of reaction is also doubled and
(2) on doubling te initial concentration of both A and B, there is a charge by a factor of 8 in the rate of the reaction.
The rate of this reaction is given byView Solution

