A typical PIN (personal identification number) is a sequence of any four symbols chosen from the 26 letters in the alphabet and the ten digits. If all PINs are equally likely, what is the probability that as randomly chosen PIN contains a repeated symbol?
The correct Answer is:2658961679616
Step by step video, text & image solution for A typical PIN (personal identification number) is a sequence of any four symbols chosen from the 26 letters in the alphabet and the ten digits. If all PINs are equally likely, what is the probability that as randomly chosen PIN contains a repeated symbol? by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PROBABILITYBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - PROBABILITYEXERCISE - SHORT ANSWER TYPE QUESTIONS
20 Videos
- PRINCIPAL OF MATHEMATICAL INDUCTION BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - PRINCIPAL OF MATHEMATICAL INDUCTION EXERCISE - Short Answer Type Questions
24 Videos
- REALATION AND FUNCTIONS BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - REALATION AND FUNCTIONS EXERCISE - SHORT ANSWER TYPE QUESTIONS (4 MARKS)
29 Videos
Similar Questions
Four digit numbers are formed by using the digits 1,2,3,4 and 5 without repeating any digit. Find the probability that a number, chosen at random, is an odd number.
View SolutionOne urn contains two black balls (labelled B1 and B2) and one white ball. A second urn contains one black ball and two white balls (labelled W1 and W2). Suppose the following experiment is performed. One of the two urns is chosen at random. Next a ball is randomly chosen from the urn. Then a second ball is chosen at random from the same urn without replacing the first ball. Write the sample space showing all possible outcomes. What is the probability that two black balls are chosen? What is the probability that two balls of opposite colour are chosen?
View Solution
Knowledge Check
Three digits are chosen at random from 1,2,3,4,5,6,7,8 and 9 without repeating any digit. What is the probability that the product is odd?
A23B748C542D5108Three digits are chosen at random from 1, 2, 3, 4, 5, 6, 7, 8 and 9 without repeating any digit. What is the probability that the product is odd?
A23B748C542D5108Three distinct vertices are chosen at random from the vertices of a gien regular polygon of (2n+1) sides. Let all such choices are equalty likely and the probability that the centre of the given polygon lies in the interior of the triangle determined by these three chosen random points is 514.
The number of diagonals of the polygon is equal toA14B18C20D27
On a normal standard dic one of the 21 dots from any one of the six faccs is removéd at random with each dot equally likely to be chosen. The die is then rolled. The probability that the top face has an odd number of dots is
View SolutionOn a normal standard die one of the 21 dots from any one of the six faces is removed at random with each dot equally likely to the chosen. If the die is then rolled, then find the probability that the odd number appears.
View SolutionA bag contains a total of 20 books on physics and mathematics, Any possible combination of books is equally likely. Ten books are chosen from the bag and it is found that it contains 6 books of mathematics. Find out the probability that the remaining books in the bag contains 3 books on mathematics.
View SolutionThree distinct vertices are chosen at random from the vertices of a gien regular polygon of (2n+1) sides. Let all such choices are equalty likely and the probability that the centre of the given polygon lies in the interior of the triangle determined by these three chosen random points is 514.
The number of points of intersection of the diagonals lying exactly inside the polygon is equal to
View SolutionAfter a day filled with adventures in Tehran, you now reach the bank of Hari river. There you find a box that contains the key to the boat which can help you cross the river quickly. The box can be opened only if the correct password is entered. On the top part of the box there is a number carved.
“101111830140311”
The box you have got can be opened by deciphering the code number given on the top of the box which would provide you the password. The password consists only English alphabets (capital). Each alphabet is coded by a three digit number in which the ones-place is given by the number of curved lines in that alphabet and the tens- place is given by the number of straight lines in the alphabet. To find the hun- dredths-place digit first place all the alphabets with the same last two digits together in their alphabetical order and interchange the first half with the second half. Now number them 1 onwards and thus the corresponding number will take the hundredths-place. Example: Let these letters (of some unknown alphabet) be the ones having one curved line and one straight line in ascending order then they will be coded as-
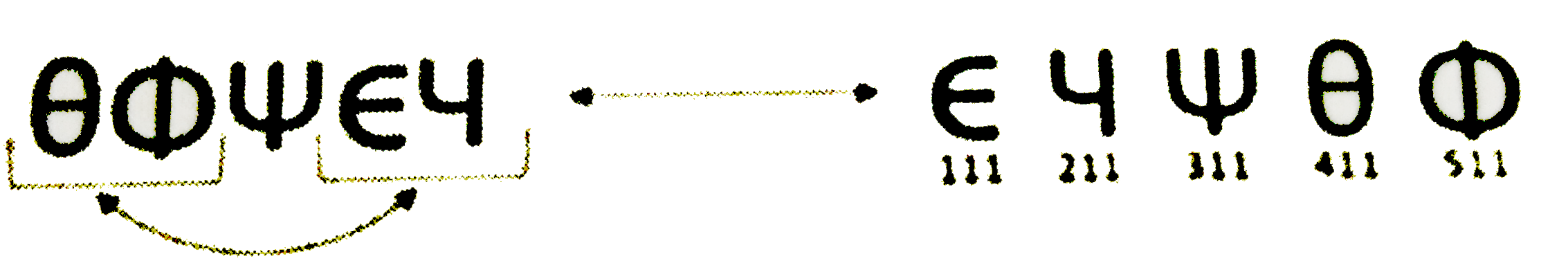
(Consider “B” to have 2 curves and 1 straight line whereas “U” to have only a curved line. Also consider these letter as shown here: GIJQRY)
What is the correct password ?
View Solution