When a particle is mass $m$ moves on the $x -$ axis in a potential of the from $V (x) = k x^{2}$ , it performs simple harmonic motion. The corresponding thime periond is proportional to $\sqrt{\frac{m}{k}}$ , as can be seen easily asing dimensional analysis. However, the motion of a pariticle can be periodic even when its potential enem increases on both sides $x = 0$ in a way different from $k x^{2}$ and its total energy is such that the particel does not escape to infinity. consider a particle of mass $m$ moving onthe $x -$ axis . Its potential energy is $V (x) = α \cdot x^{4} (α > 0$ ) for $| x |$ near the origin and becomes a constant equal to $V_{0}$ for $| x | \geq X_{0}$ (see figure)

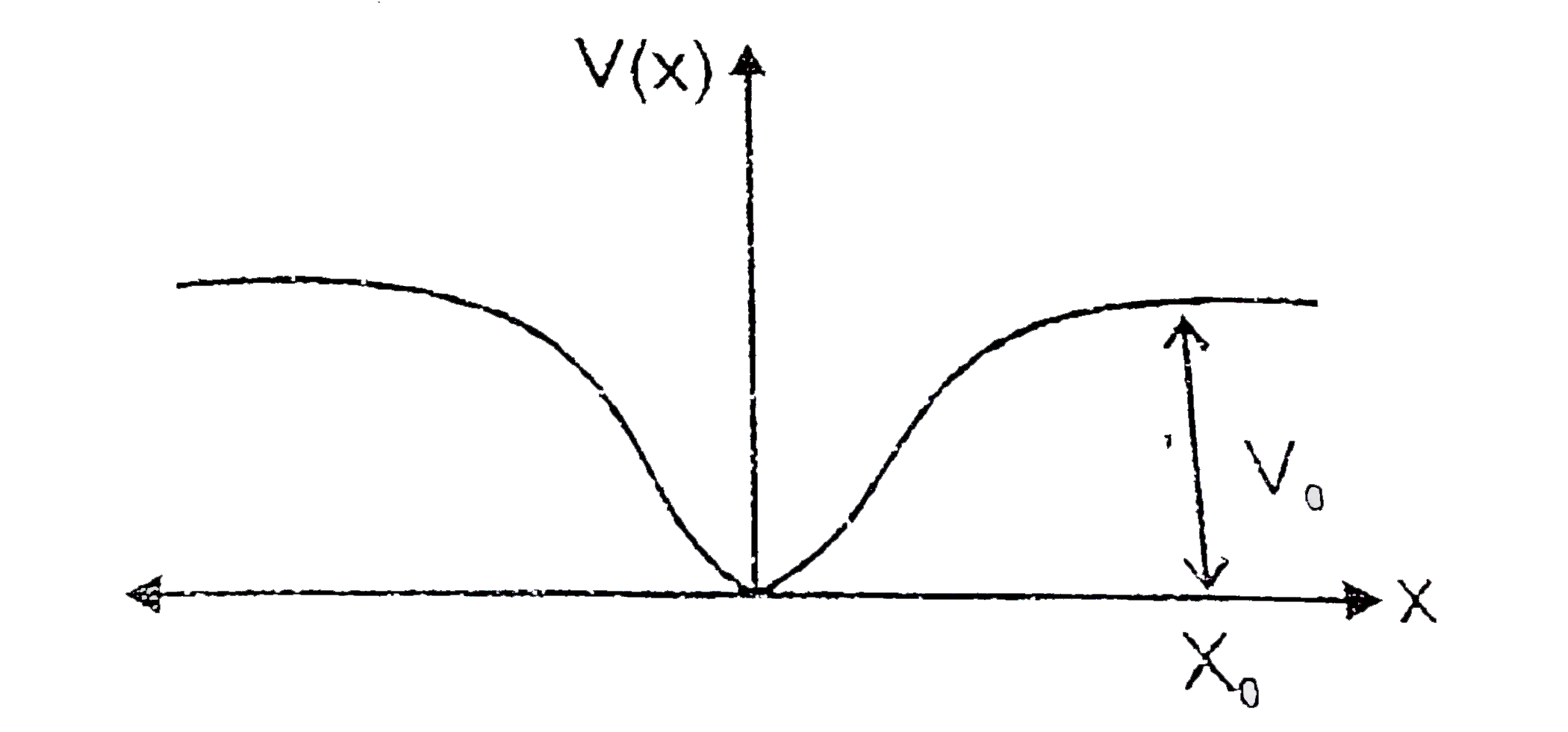
If the total energy of the particle is $E$ , it will perform is periodic motion why if :

$E < 0$

$E > 0$

$V_{0} > E > 0$

$E > V_{0}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

When $0 < E < V_{0}$ there will be acting a restoring force to perform oscillation because in this case paticle will be in the region $| x | \leq x_{0}$ .

Updated on:21/07/2023

Class 12 PHYSICS SIMPLE HARMONIC MOTION

Topper's Solved these Questions

Knowledge Check

Question 1 - Select One
When a particle of mass m moves on the X-axis in a potential of the form $V (x) = k x^{2}$ , it performs simple harmonic motion. The corresponding time period is proportional to
$\sqrt{\frac{m}{k}}$ , as can be seen easily using dimensional analysis.
However, the motion of a particle can be periodic even when its potential energy increases on both sides of x = 0 in a way different from $k x^{2}$ and its total energy is such that the particle does not escape to infinity. Consider a particle of mass m moving on the X-axis. Its potential energy is V(x) $= a x^{2} (a > 0)$ for |x| near the origin and becomes a constant equal to $V_{0}$ for $| x | \geq X_{0}$

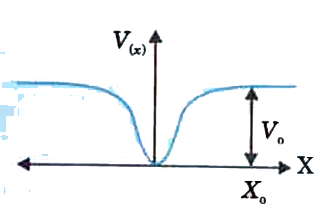
The acceleration of this particle for $| X | > X_{0}$ is
Aproportional to $(\frac{v_{0}}{m X_{0}})$
Bpropotinal to $\frac{\sqrt{V_{0}}}{m X_{0}}$
Cproportional to $V_{0}$
DZero
Question 2 - Select One
When a particle of mass m moves on the X-axis in a potential of the form $V (x) = k x^{2}$ , it performs simple harmonic motion. The corresponding time period is proportional to
$\sqrt{\frac{m}{k}}$ , as can be seen easily using dimensional analysis.
However, the motion of a particle can be periodic even when its potential energy increases on both sides of x = 0 in a way different from $k x^{2}$ and its total energy is such that the particle does not escape to infinity. Consider a particle of mass m moving on the X-axis. Its potential energy is V(x) $= a x^{2} (a > 0)$ for |x| near the origin and becomes a constant equal to $V_{0}$ for $| x | \geq X_{0}$

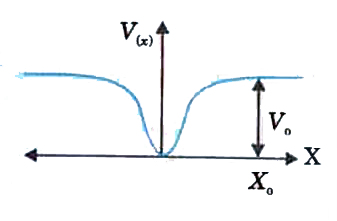
For periodic motion os small amplitude A . The time periodic T of this particle is proportional to
A $A \frac{\sqrt{m}}{α}$
B $\frac{1}{A} \frac{\sqrt{m}}{α}$
C $A \frac{\sqrt{α}}{m}$
D $\frac{1}{A} \frac{\sqrt{α}}{m}$
Question 3 - Select One
A body is performing simple harmonic motion. Then its
Aaverage total energy per cycle is equal to its maximum kinetic energy.
Baverage kinetic energy per cycle is equal to half of its maximum kinetic energy.
Caverage total energy per cycle is equal to half of its malximum kinetic energy.
DNone of these.