माना C1 और C2 क्रमशः फलन y=x2 औरy=2x,0≤x≤1 के ग्राफ हैं। माना C3 फलन, y=f(x),0≤x≤1,f(0)=0 का ग्राफ है। C1 के एक बिंदु P के लिए माना कि बिन्दु P से होकर जाने वाली तथा अक्षों के समान्तर रेखायें C2 व C3 को क्रमशः Q और R पर मिलती है।
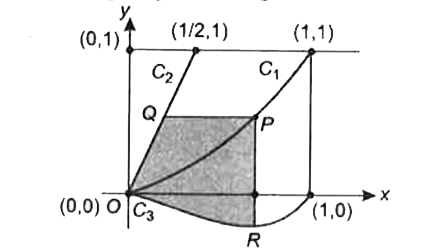 यदिP की प्रत्येक स्थिति ( C1 पर ) के लिए छायांकित क्षेत्र OPQ और ORP का क्षेत्रफल समान हो,तो फलन f(x) को ज्ञात कीजिए।
यदिP की प्रत्येक स्थिति ( C1 पर ) के लिए छायांकित क्षेत्र OPQ और ORP का क्षेत्रफल समान हो,तो फलन f(x) को ज्ञात कीजिए।
The correct Answer is:f(x)=x3−x2,0≤x≤1
Topper's Solved these Questions
- क्षेत्रफल Book:JEE Main & Advanced (Hindi Medium)Chapter:क्षेत्रफल Exercise:रिक्त स्थानों की पूर्ति कीजिए
Explore 2 Videos
- क्रमचय एवं संचय Book:JEE Main & Advanced (Hindi Medium)Chapter:क्रमचय एवं संचय Exercise:एकल पूर्णांक प्रश्न
Explore 5 Videos
- गणितीय आगमन का सिद्धांत Book:JEE Main & Advanced (Hindi Medium)Chapter:गणितीय आगमन का सिद्धांत Exercise:विश्लेषणात्मक प्रश्न
Explore 9 Videos
Similar Questions
वक्र |x|+|y|=1 से घिरा क्षेत्रफल ............ है।
View Solutionआठ सदिशों का समुच्चय V={aˆi+bˆj+cˆk,a,b,c∈{−1,1}} लीजिए। V से तीन असमतलीय सदिश 2P प्रकार से चुने जा सकते है। तब p का मान है।
View Solutionमाना u1=1,u2=1 और un+1=un+1+un'n≥1गणितीय आगमन के प्रयोग से दिखाइए कि un=1√5[(1+√52)n−(1−√52)n],∀n>1
View SolutionIf (1+x+x^(2))^(n)=(C_(0)+C_(1)x+C_(2)x^(2)+.........) then the value of C_(0)C_(1)-C_(1)C_(2)+C_(2)C_(3)...
View SolutionIf (1+x)n=C0+C1x+C2x2+….+Cnxn then prove that (ΣΣ)0≤i<j≤nCiC2j=(n−1)2nCn+22n
View SolutionIf (1+x)n=C0+C1x+C2x2+…+Cnxn, find the value of
∑∑0≤i<j≤n(i+j)(Ci+CiCj).
View SolutionLet n be a positive integer and
(1+x)n+C0+C1x+C2x2+C3x3+...+Crxr+...+Cn−1xn−1+Cnxn
Where Cr stands for .nCr, then
Q. The value of ∑∑0≤r<s≤n(Cr±CS)2 is
View Solutionx=1 पर फलन f के सांतत्य का परिक्षण कीजिए
f(x)={2x+3,0≤x<1के लिए 3x+2,1≤x≤2के लिए
View SolutionIf (1+x)n=C0+C1x+C2x2+..........+Cnxn then prove that ∑ ∑(Ci+cj)2=(n−1).2nCn+22n0≤i<j≤n
View Solutionनिम्न असमिकाओं के ग्राफ खीचिंए :
x+y−4≤0.
View SolutionIf f(x) is a differentiable function wherever it is continuous and f'(c1)=f'(c2)=0,f''(c1).f''(c2)<0,f(c1)=5,f(c2)=0and(c1<c2)
If f(x) is continuous in [c1,c2]andf''(c1)−f''(c2)>0, then minimum number of roots of f'(x)=0 in [c1−1,c2+1] is
View SolutionIf f(x) is a differentiable function wherever it is continuous and f'(c1)=f'(c2)=0,f''(c1).f''(c2)<0,f(c1)=5,f(c2)=0and(c1<c2)
If f(x) is continuous in [c1,c2]andf''(c1)−f''(c2)<0, then minimum number of roots of f'(x)=0 in c1−1,c2+1] is
View SolutionIf f(x) is a differentiable function wherever it is continuous and f'(c1)=f'(c2)=0,f''(c1).f''(c2)<0,f(c1)=5,f(c2)=0and(c1<c2)
If f(x) is continuous in [c1,c2] and f''(c1)−f''(c2)>0, then minimum number of roots of f(x)=0 in [c2−1,c2+1] is
View Solutionफलन के लिए रोल प्रमेय की सत्यता की जाँच कीजिए
f(x)=⎧⎪⎨⎪⎩x2+10≤x≤1यदि3−x1<x≤2
View Solutionमाना C1 तथा C2 क्रमश: वृत्तों x2+y2−2x−2y−2=0 तथा x2+y2−6x−6y+14=0 के केन्द्र है । यदि P तथा Q इन वृत्तों के प्रतिच्छेद बिन्दु है , तो चतुर्भुज PC1QC2 का क्षेत्रफल (वर्ग इकाइयो में) है
View Solution
