The minimum mass of mixture of A2 and B2 required to produce at least 1 kg of each product is : (Given At. Mass of 'A' = 10 : At. Mass of 'B' = 120 )
2120 gm
1060 gm
560 gm
1660 gm
The correct Answer is:A
Topper's Solved these Questions
- BOOK - ALLENCHAPTER - MOLE CONCEPTEXERCISE - O-II14videos
MOLE CONCEPT
- BOOK - ALLENCHAPTER - MOLE CONCEPTEXERCISE - O-II (Assertion Reason:)2videos
MOLE CONCEPT
- BOOK - ALLENCHAPTER - MOLE CONCEPTEXERCISE - S-II18videos
MOLE CONCEPT
- BOOK - ALLENCHAPTER - METALLURGYEXERCISE - EXERCISE-05 [B]26videos
METALLURGY
- BOOK - ALLENCHAPTER - p-Block Element EXERCISE - All Questions20videos
p-Block Element
Similar Questions
Knowledge Check
- Question 1 - Select One
The minimum mass of mixture of A2 and B4 required to produce at least 1 kg of each product is :
(Given At. mass of ‘A’ = 10, At mass of ‘B’ = 120)
5A2+2B4→2AB2+4A2BA2120 gmB1060 gmC560 gmD1660 gm - Question 2 - Select One
The mass of oxygen that would be required to produce enough CO which completely reduces 1⋅6 kg Fe2O3 (at. mass of Fe = 56)
A240 gB480 gC720 gD960 g - Question 3 - Select One
Eq. mass of AxBy is
Ax×Eq. mass of A+y×Eq. mass of BBy×Eq. mass of A+x×Eq. mass of BCEq. mass of A + Eq. mass of BDNone of these
A mixture of AB3(g) and A2B4(g) is placed in a sealed container. (At. Mass of A = 14 and At. ,mass of B =1 )
The temperature initially maintained is 300K. The total pressure is 0.5 atm. The container is heated to 1200k, when both AB3 and A2B4 decomposed to A2 and B2 when the decomposition was complete the pressure observed was 4.5 atm.
What is the percentage of AB3(g) in the original mixture ?View SolutionIf the mass of the block A = 10 kg and the coefficient of static and kinetic friction is 0.2, then the mass of block B to start the motion is
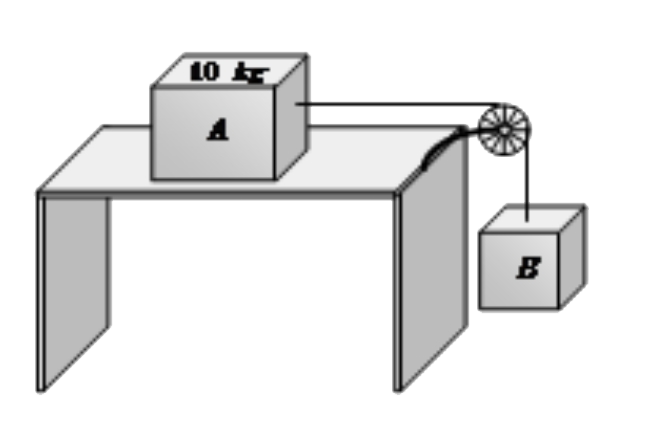 View Solution
View SolutionA and B are two objects with masses 10 kg and 40 kg respectively. Then
View SolutionThree masses A, B and C are arranged as shown in the figure, the mass of 'A' is 2 times the mass of 'B' and the mass of B is 10 kg. If the force of attraction between A and B is equal to the force of attraction between B and C, then the mass of C is _________ kg.
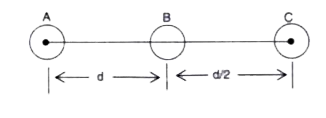 View Solution
View SolutionThree masses A, B and C are arranged as shown in the figure, the mass of 'A' is 2 times the mass of 'B' and the mass of B is 10 kg. If the force of attraction between A and B is equal to the force of attraction between B and C, then the mass of C is _________ kg.
 View Solution
View Solution

