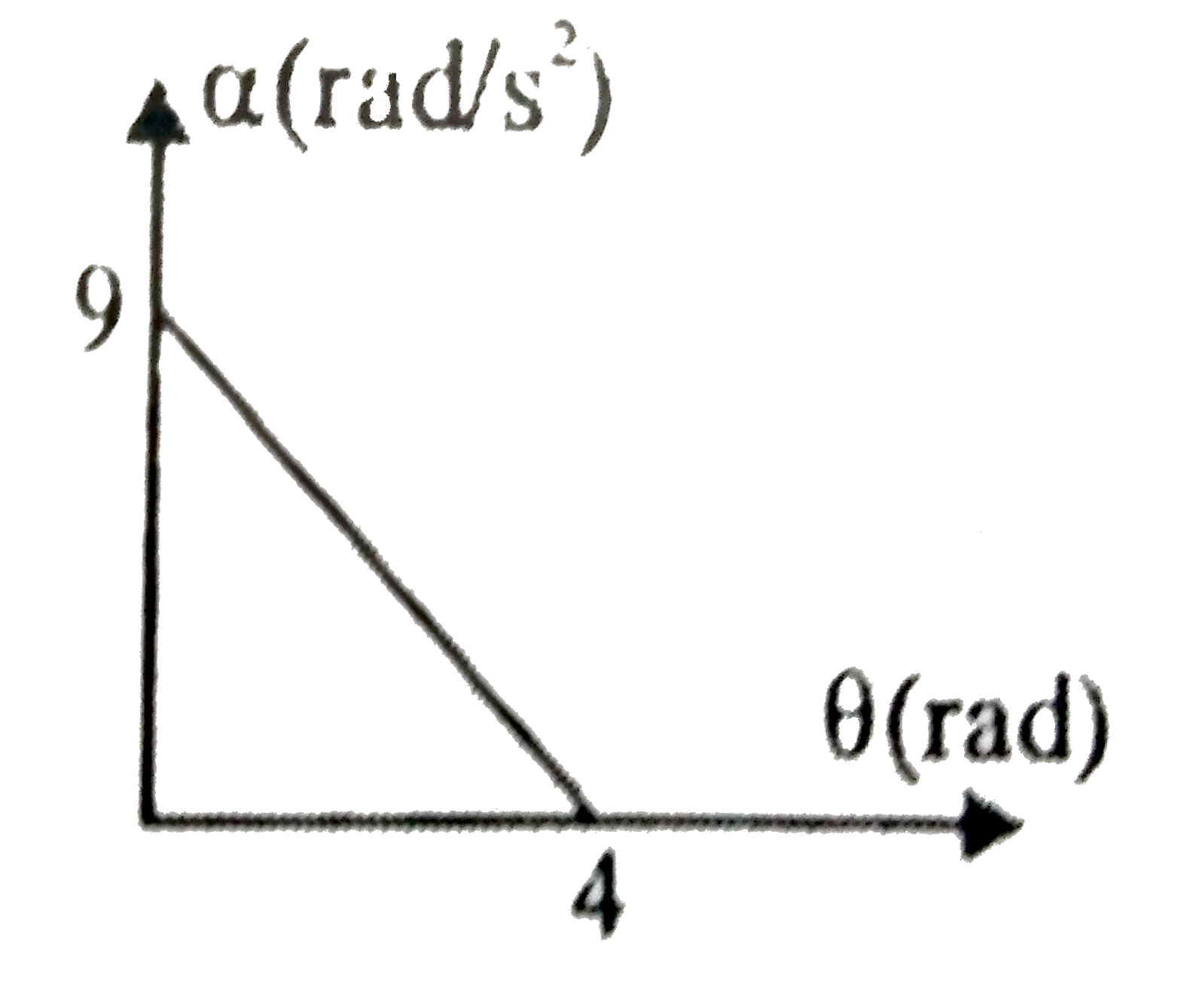
A particle starts moving in a non-uniform circular motion, lies angular acceleration as shown in figure. The angular velocity at the end of 4 radian is given by $ω$ rad/s then find the value of $ω$ .

Video Solution

Text Solution

Verified by Experts

The correct Answer is:6

|

Answer

Step by step video, text & image solution for A particle starts moving in a non-uniform circular motion, lies angular acceleration as shown in figure. The angular velocity at the end of 4 radian is given by omega rad/s then find the value of omega. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 PHYSICS CIRCULAR MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
In nonuniform circular motion, the linear acceleration $\to a$ , the angular acceleration, $\to α$ , the angular velocity $\to v$ the radius vector $\to r$ and the angular velocity $\to ω$ at any instant are related by the vector equation,
A $\to a = \to α \times \to r + \to ω \times \to v$
B $\to a = \to r \times \to α + \to v \times \to ω$
C $\to a = \to α \times r + \to v \times \to ω$
D $\to a = \to α \times r - \to ω \times \to v$
Question 2 - Select One
A particle is in circular motion in a horizotantal plane. It has angular velocity of 10 $π r a d / s$ at the end of 2 s and angular velocity 15 $π r a d / s$ at the end of 4s. The angular acceleration of particle is
A5 $π$ $r a d / s^{2}$
B2.5 $π$ $r a d / s^{2}$
C6 $π$ $r a d / s^{2}$
D16 $π$ $r a d / s^{2}$
Question 3 - Select One
A body rotating with uniform angular acceleration covers $100 π$ (radian) in the first 5 s after the start. Its angular speed at the end of 5 s (in rad/s) is
A $40 π$
B $30 π$
C $20 π$
D $10 π$

ALLEN-CIRCULAR MOTION-EXERCISE (S-1)

Home
Ask Doubt
Profile