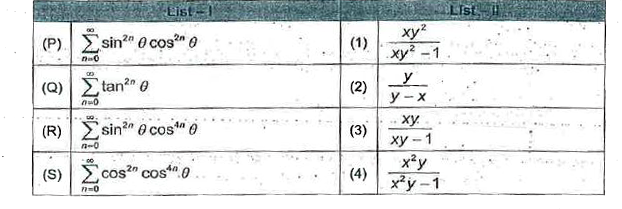
For 0≤θ<π4, let x=∞∑n=0(sinθ)2n,y=∞∑n=0(cosθ)2n then sum of the series
PQRS(A)2413(B)3241(C)1324(D)4123
Text Solution
The correct Answer is:B
|
Topper's Solved these Questions
- PROGRESSION & SERIES BOOK - FIITJEECHAPTER - PROGRESSION & SERIES EXERCISE - EXERCISE 1
5 Videos
- PROGRESSION & SERIES BOOK - FIITJEECHAPTER - PROGRESSION & SERIES EXERCISE - EXERCISE 2
4 Videos
- PROGRESSION & SERIES BOOK - FIITJEECHAPTER - PROGRESSION & SERIES EXERCISE - SOLVED PROBLEMS (SUBJECTIVE)
11 Videos
- PROBABILITYBOOK - FIITJEECHAPTER - PROBABILITYEXERCISE - Exercise 7
2 Videos
- QUADRATIC EQUATION & EXPRESSION BOOK - FIITJEECHAPTER - QUADRATIC EQUATION & EXPRESSION EXERCISE - NUMERICAL BASED
3 Videos
Similar Questions
Knowledge Check
Let x=∞∑n=0(−1)n(tanθ)2n and y=∞∑n=0(cosθ)2n qhere θ∈(0,π4), then
Ax(y+1)=1By(1-x) = 1Cy(x-1)=1Dy(1+x) = 1If 0<θ,ϕ<π2,x=∞∑n=0cos2nθ,y=∞∑n=0sin2nϕ and z=∞∑n=0cos2nθ⋅sin2nϕ then :
Axy−z=(x+y)zBxy+yz+zx=zCxyz=4Dxy+z=(x+y)zIf θ∈(π/4,π/2) and ∞∑n=11tannθ=sinθ+cosθ, then the value of tan θ is
A√3B√2+1C2+√3D√2
For 0<ϕ≤π2, if x=∞∑n=0cos2nϕ,y=∞∑n=0sin2nϕ,z=∞∑n=0cos2nϕsin2nϕ, then
View SolutionFor 0<θ<π4, let x=∞∑n=0sin2nθ,y=∞∑n=0cos2nθ, then match the entries of column-I with column-II
Column-IColumn-II(a)∑∞0sin2nθcos2nθ(p)xy2xy2−1(b)∑∞0tan2nθ(q)yy−x(c)∑∞0sin2nθcos4nθ(r)xyxy−1(d)∑∞0cos2nθsin4nθ(s)x2yx2y−1
View Solution∞∑01(3n+1)(3n+2)
View SolutionIf 0<θ<π2,x=∞∑n=0cos2nθ,y=∞∑n=0sin2nθ and z=∞∑n=0cos2nθ⋅Sin2nθ, then show xyz=xy+z.
(a) xyz = xz + y
(b) xyz = xy + z
(c) xyz = x + y + z
(d) xyz = yz + x
View SolutionIf θ∈(π/4,π/2) and ∞∑n=11tannθ=sinθ+cosθ, then the value of tan θ is
View Solution
