If the electric flux entering and leaving an enclosed surface respectively is ϕ1 and ϕ2, the electric charge inside the surface will be
A
ϕ2−ϕ1ε0
B
ϕ2+ϕ1ε0
C
ϕ1−ϕ2ε0
D
ε0(ϕ2−ϕ1)
Video Solution
Text Solution
The correct Answer is:D
According to gauss theorem, the net electric flux through any closed surface is equal to the net charge inside the surface divided by ε0
Therefore ϕ=qε0
Let q1 be the charge due to which flux f1 id entering the surface
ϕ1=−q1ε0 or −q1=ε0ϕ1
Let +q2 be the charge due to which flux f2 is leaving the surface
ϕ2=q2ε0 or q2=ε0ϕ2
So electric charge inside the surface
=q2−q10=ε0ϕ2+ε0ϕ1=ε0(ϕ2−ϕ1)
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - EXERCISE -443videos
ELECTRIC CHARGES AND FIELDS
- BOOK - NARAYNACHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - EXERCISE -2 (H.W)36videos
ELECTRIC CHARGES AND FIELDS
- BOOK - NARAYNACHAPTER - DUAL NATUREEXERCISE - LEVEL-II (H.W)17videos
DUAL NATURE
- BOOK - NARAYNACHAPTER - ELECTRO MAGNETIC INDUCTIONEXERCISE - Level-II (H.W)23videos
ELECTRO MAGNETIC INDUCTION
Similar Questions
Knowledge Check
- Question 1 - Select One
If the electric flux entering and leaving an enclosed surface respectively is ϕ1 and ϕ2 then the electric charge inside the surface will he
A(ϕ+ϕ2)ε0B(ϕ2−ϕ1)ε0C(ϕ1−ϕ2)ε0D(−ϕ1−ϕ2)ε0 - Question 2 - Select One
If the electric flux entering and leaving an enclosed surface respectively is ϕ1 and ϕ2, the electric charge inside the surface will be
Aϕ3−ϕ1εnBϕ2+ϕ1εnC(ϕ1−ϕ3)εnDεn(ϕ2−ϕ1) - Question 3 - Select One
If the electric flux entering and leaving an enclosed surface are ϕ1andϕ2 respectively, then the electric charge present within the surface is
A1∈0(ϕ1+ϕ2)B1∈0(ϕ2−ϕ1)C∈0(ϕ1+ϕ2)D∈0(ϕ2−ϕ1)
Electric flux through a closed surface in free space enclosing a charge q is
View SolutionThe electric flux through the surface
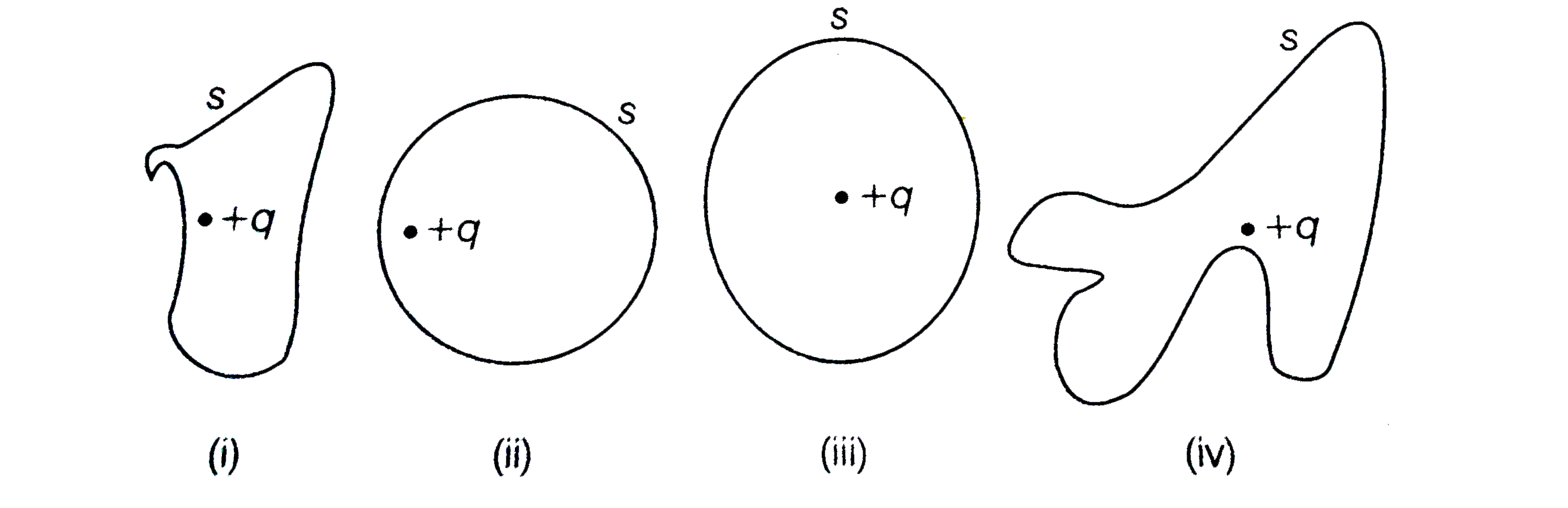 View Solution
View SolutionThe electric flux through the surface
 View Solution
View SolutionIf the electric flux entering and leaving a closed surface are 6×106 and 9×106 SI units respectively, then the net charge inside the surface of permittivity of free space ε0 is
View SolutionIf ϕ1andϕ2 are normal of flux entering and leaving an enclosed surface. Electric flux inside the surface will be
View Solution