Two identical capacitors 1 and 2 are connected in series to a battery as shown in figure. Capacitor 2 contains a dielectric slab of dielectric constant K as shown. Q1andQ2 are the charges stored in the capacitors. Now the dielectric slab is removed and the corresponding charges are Q1andQ2. Then

Q1Q2=K+1K
Q2Q2=K+12
Q2Q2=K+12K
Q2Q2=K2
The correct Answer is:C
Q1=Q2=CE2, Before the slab is removed
C1=CandC2=kC,Cnet=(kk+1)C
Q2Q2=k+12k
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCEBOOK - NARAYNACHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCEEXERCISE - EXERCISE -2 (H.W)
50 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCEBOOK - NARAYNACHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCEEXERCISE - EXERCISE - 3 (PREVIOUS AIPMT QUESTIONS)
23 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCEBOOK - NARAYNACHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCEEXERCISE - Exercise -1 (H.W)
43 Videos
- ELECTROMAGNETIC WAVES BOOK - NARAYNACHAPTER - ELECTROMAGNETIC WAVES EXERCISE - EXERCISE -4
15 Videos
- ELECTROSTATICS AND GAUSS LAWBOOK - NARAYNACHAPTER - ELECTROSTATICS AND GAUSS LAWEXERCISE - Intergers type question
11 Videos
Similar Questions
Two identical parallel plate capacitors are connected in series to a battery of 100V. A dielectric slab of dielectric constant 4.0 is inserted between the plates of second capacitor. The potential difference across the capacitors will now be respectively
View SolutionTwo identical capacitor C1 and C2 are connected in series with a battery. They are fully charged. Now a dielectric slab is inserted between the plates of C2. The potential across C1 will :
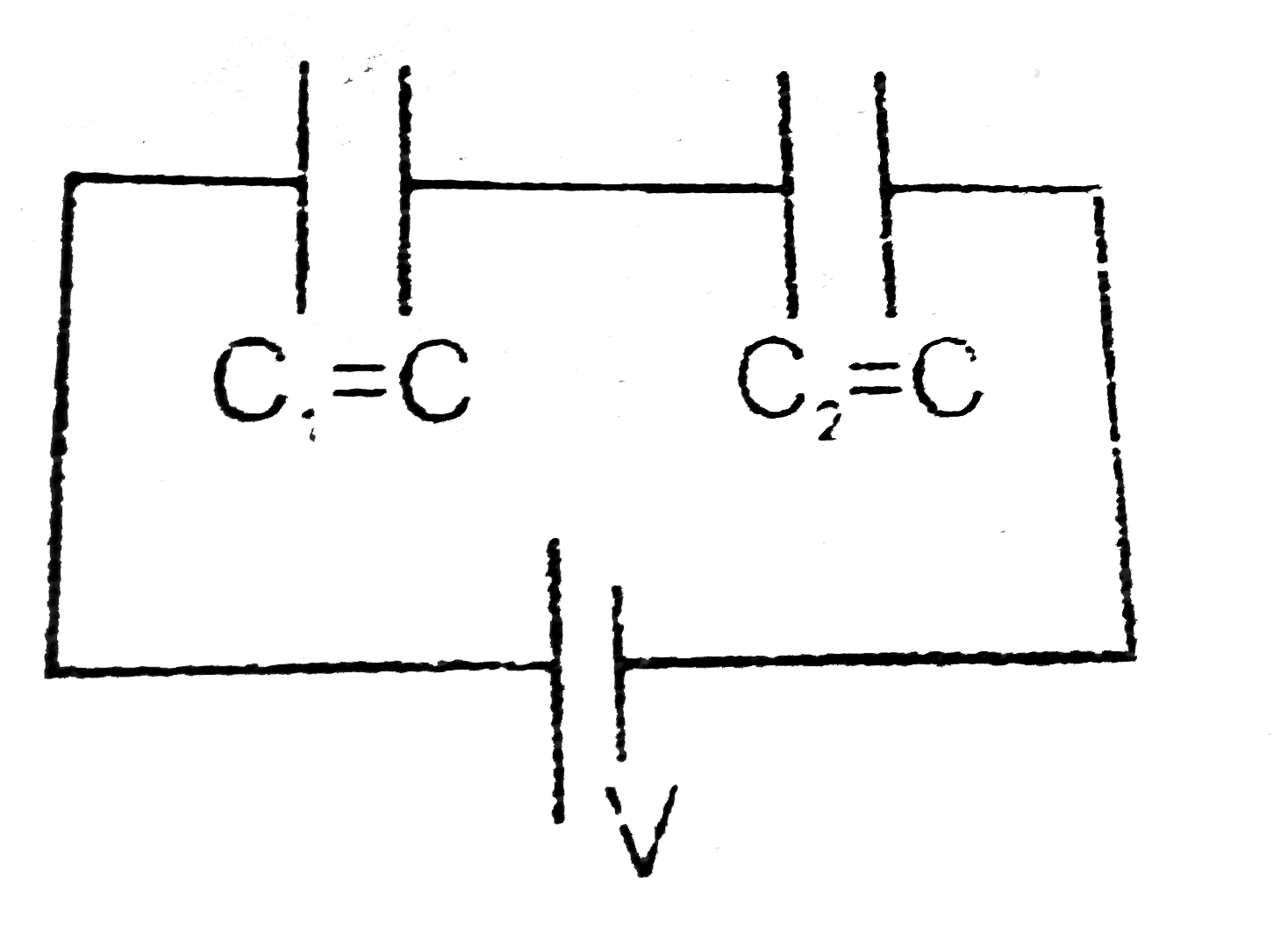
View Solution
Knowledge Check
Two identical capacitors 1 and 2 are connected in series to a batery as shown in figure. Capacitor 2 contains a dielectric slab of dieletric constant k as shown. Q1 and Q2 are the charges stored in the capacitors. Now the dielectirc slab us removed and the corresponding charges are Q'1 and Q'2. Then
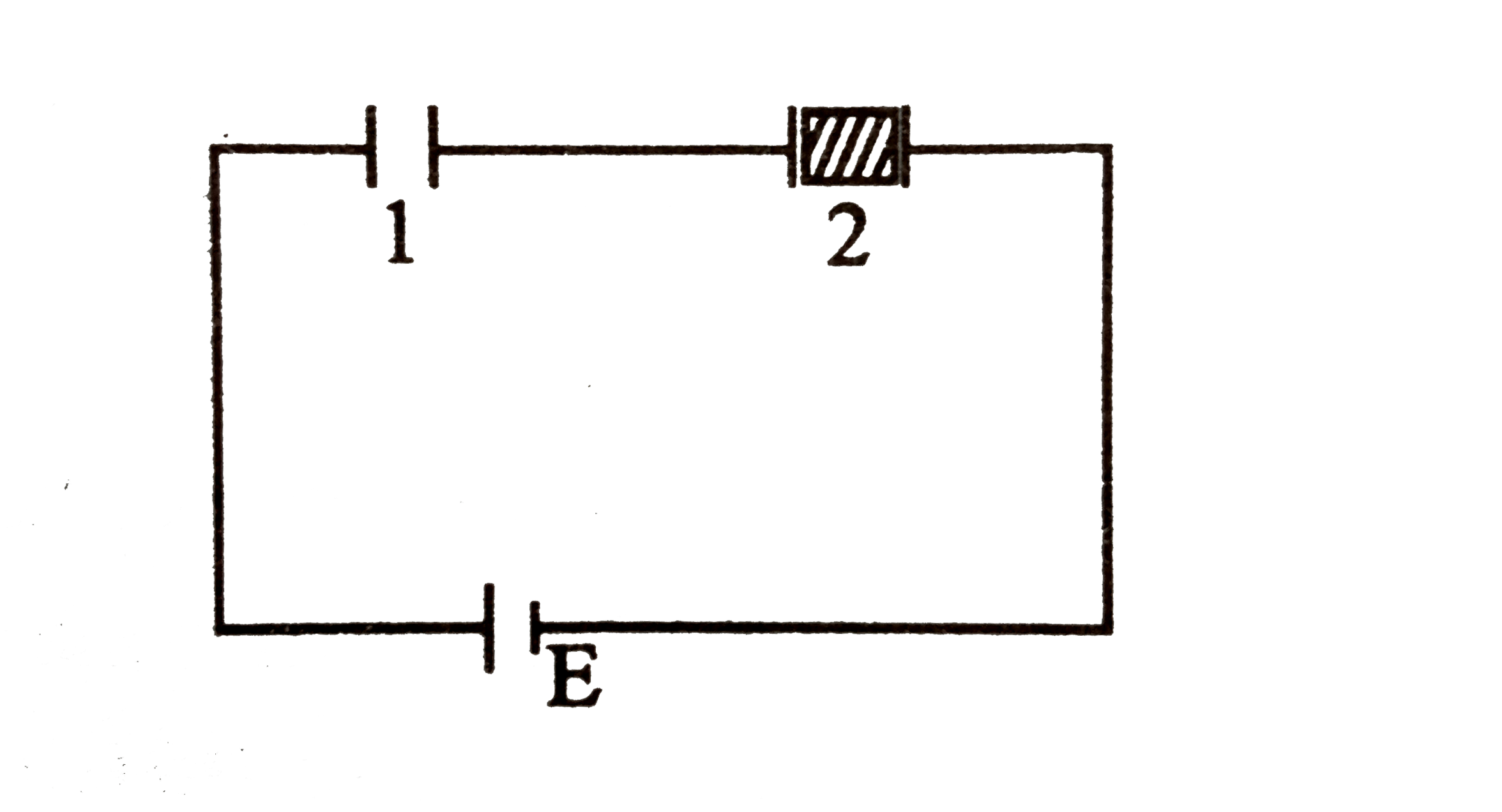 AQ'1Q1k+1kBQ'2Q2=k+12CQ'2Q2=k+12kDQ'1Q1=k2
AQ'1Q1k+1kBQ'2Q2=k+12CQ'2Q2=k+12kDQ'1Q1=k2Two identical capacitors 1 and 2 are connected in series to a batery as shown in figure. Capacitor 2 contains a dielectric slab of dieletric constant k as shown. Q1 and Q2 are the charges stored in the capacitors. Now the dielectirc slab us removed and the corresponding charges are Q'1 and Q'2. Then
 AQ'1Q1=K+1KBQ'2Q2=K+12CQ'2Q2=K+12KDQ'1Q1=K2
AQ'1Q1=K+1KBQ'2Q2=K+12CQ'2Q2=K+12KDQ'1Q1=K2Two identical parallel plate capacitors are connected in series to a battery of 100V. A dielectric slab of dielectric constant 4.0 is inserted between the plates of second capacitor. The potential difference across the capacitors will now be respectively
A50V,50VB80V,20VC20V,80VD75V,25V
Two identical capacitors A and B shown in the given circuit are joined in series with a battery. If a dielectric slab of dielectric constant K is slipped between the plates of capacitor B and battery remains connected, then the energy of capacitor A will-
View SolutionTwo identical capacitor C1 and C2 are connected in series with a battery. They are fully charged. Now a dielectric slab is inserted between the plates of C2. The potential across C1 will :
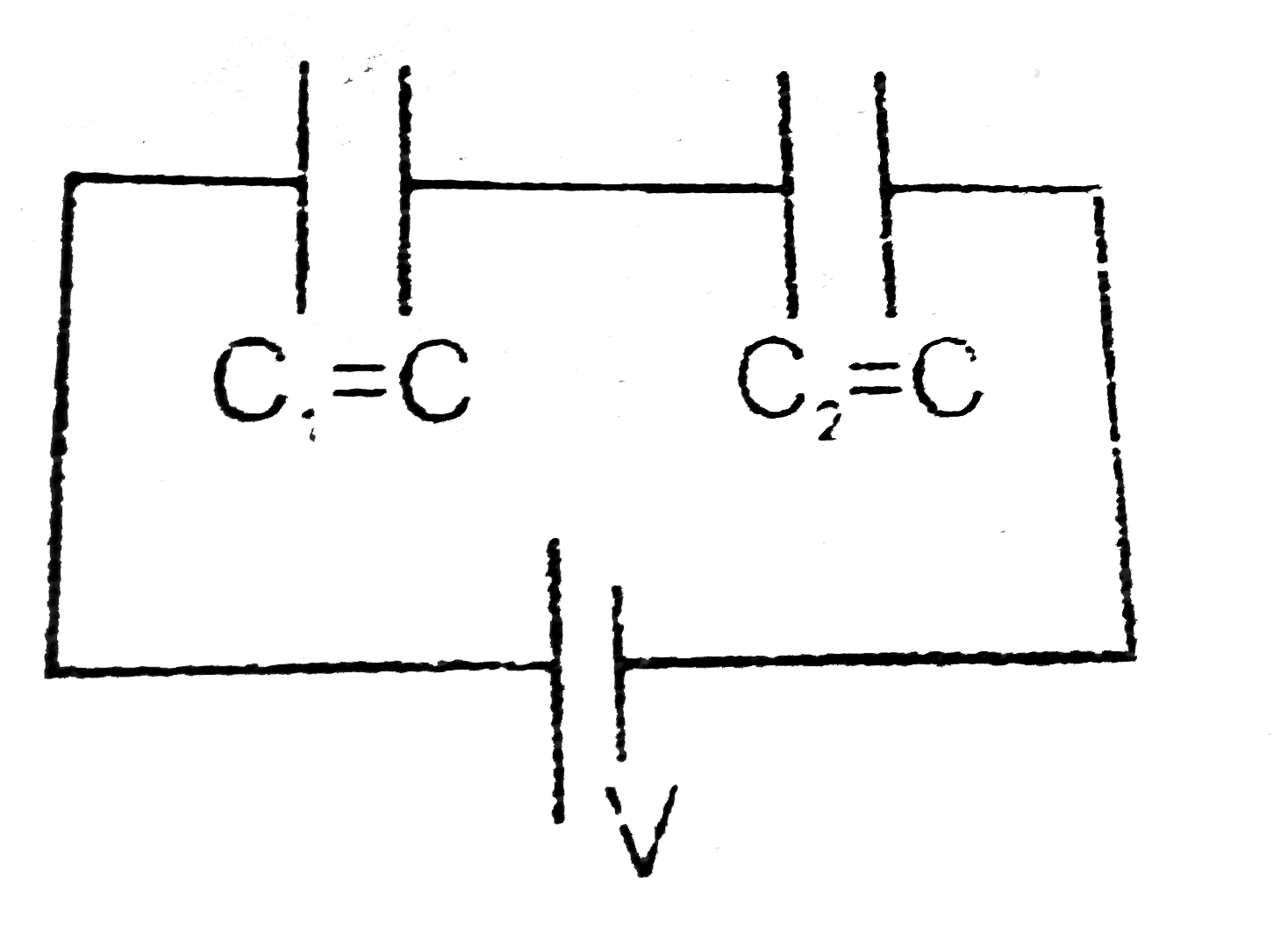
View SolutionTwo identical capacitors are connected as shown in the following figure. If a dielectric slab is inserted in B, choose correct statement
View SolutionTwo identical capacitors A and B shown in the given circuit are joined in series with a battery. If a dielectric slab of dielectric constant K is slipped between the plates of capacitor B and battery remains connected, then the energy of capacitor A will-

View SolutionA capacitor is connected to a battery of voltage V. Now a di-electric slab of di-electric constant k is completely inserted between the plates, then the final charge on the capacitor will be : (If initial charge is q0)
View Solution
