Using three wires of resistances 1 ohm, 2 ohm and 3 ohm, then no.of different values of resistances that possible are
6
4
10
8
The correct Answer is:D
To solve the problem of finding the number of different resistance values possible using three wires with resistances of 1 ohm, 2 ohm, and 3 ohm, we can explore various combinations of these resistances in series and parallel configurations.
1. Understanding Series and Parallel Combinations:
- In a series combination, the total resistance Rs is the sum of the individual resistances:
Rs=R1+R2+R3
- In a parallel combination, the total resistance Rp is given by:
1Rp=1R1+1R2+1R3
2. Calculating All Possible Combinations:
- We can use the three resistors in different combinations:
- All three in series
- All three in parallel
- Any two in series and one in parallel
- Any two in parallel and one in series
3. Calculating Individual Combinations:
- All in Series:
Rseries=1+2+3=6 ohms
- All in Parallel:
1Rparallel=11+12+13=1+0.5+0.333=1.833⟹Rparallel≈0.545 ohms
4. Two in Series, One in Parallel:
- (1 ohm and 2 ohm in series, 3 ohm in parallel):
Rs1=1+2=3 ohms
1Rp1=13+13⟹Rp1=32=1.5 ohms
- (1 ohm and 3 ohm in series, 2 ohm in parallel):
Rs2=1+3=4 ohms
1Rp2=14+12⟹Rp2=43≈1.333 ohms
- (2 ohm and 3 ohm in series, 1 ohm in parallel):
Rs3=2+3=5 ohms
1Rp3=15+12⟹Rp3=107≈1.428 ohms
5. Two in Parallel, One in Series:
- (1 ohm and 2 ohm in parallel, 3 ohm in series):
1Rp4=11+12⟹Rp4=23≈0.667 ohms
Rtotal=Rp4+3=3.667 ohms
- (1 ohm and 3 ohm in parallel, 2 ohm in series):
1Rp5=11+13⟹Rp5=34=0.75 ohms
Rtotal=Rp5+2=2.75 ohms
- (2 ohm and 3 ohm in parallel, 1 ohm in series):
1Rp6=12+13⟹Rp6=65=1.2 ohms
Rtotal=Rp6+1=2.2 ohms
6. Listing All Unique Resistance Values:
- From the calculations, we have the following unique resistance values:
- 6 ohms (all in series)
- 0.545 ohms (all in parallel)
- 1.5 ohms (1 & 2 in series, 3 in parallel)
- 1.333 ohms (1 & 3 in series, 2 in parallel)
- 1.428 ohms (2 & 3 in series, 1 in parallel)
- 3.667 ohms (1 & 2 in parallel, 3 in series)
- 2.75 ohms (1 & 3 in parallel, 2 in series)
- 2.2 ohms (2 & 3 in parallel, 1 in series)
7. Counting Unique Values:
- The unique resistance values are: 0.545, 1.2, 1.333, 1.428, 1.5, 2.2, 2.75, 3.667, and 6.
- Total unique values = 9.
Final Answer:
The total number of different resistance values possible using the three wires is 9.
- In a series combination, the total resistance Rs is the sum of the individual resistances:
Rs=R1+R2+R3
- In a parallel combination, the total resistance Rp is given by:
1Rp=1R1+1R2+1R3
- We can use the three resistors in different combinations:
- All three in series
- All three in parallel
- Any two in series and one in parallel
- Any two in parallel and one in series
- All in Series:
Rseries=1+2+3=6 ohms
- All in Parallel:
1Rparallel=11+12+13=1+0.5+0.333=1.833⟹Rparallel≈0.545 ohms
- (1 ohm and 2 ohm in series, 3 ohm in parallel):
Rs1=1+2=3 ohms
1Rp1=13+13⟹Rp1=32=1.5 ohms
- (1 ohm and 3 ohm in series, 2 ohm in parallel):
Rs2=1+3=4 ohms
1Rp2=14+12⟹Rp2=43≈1.333 ohms
- (2 ohm and 3 ohm in series, 1 ohm in parallel):
Rs3=2+3=5 ohms
1Rp3=15+12⟹Rp3=107≈1.428 ohms
- (1 ohm and 2 ohm in parallel, 3 ohm in series):
1Rp4=11+12⟹Rp4=23≈0.667 ohms
Rtotal=Rp4+3=3.667 ohms
- (1 ohm and 3 ohm in parallel, 2 ohm in series):
1Rp5=11+13⟹Rp5=34=0.75 ohms
Rtotal=Rp5+2=2.75 ohms
- (2 ohm and 3 ohm in parallel, 1 ohm in series):
1Rp6=12+13⟹Rp6=65=1.2 ohms
Rtotal=Rp6+1=2.2 ohms
- From the calculations, we have the following unique resistance values:
- 6 ohms (all in series)
- 0.545 ohms (all in parallel)
- 1.5 ohms (1 & 2 in series, 3 in parallel)
- 1.333 ohms (1 & 3 in series, 2 in parallel)
- 1.428 ohms (2 & 3 in series, 1 in parallel)
- 3.667 ohms (1 & 2 in parallel, 3 in series)
- 2.75 ohms (1 & 3 in parallel, 2 in series)
- 2.2 ohms (2 & 3 in parallel, 1 in series)
- The unique resistance values are: 0.545, 1.2, 1.333, 1.428, 1.5, 2.2, 2.75, 3.667, and 6.
- Total unique values = 9.
The total number of different resistance values possible using the three wires is 9.
Similar Questions
How can three resistances of 2, 3 and 6 ohms be connected so as to give a total resistance of 1 ohm? Justify your answer.
View SolutionTwo batteries one of the emf 3V, internal resistance 1 ohm and the other of emf 15 V , internal resistance 2 ohm are connected in series with a resistance R as shown . If the potential difference between a and b is zero the resistance of R in ohm is
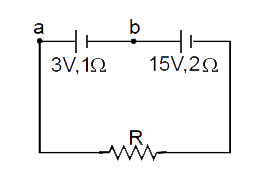 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Using three wires of resistances 1 ohm, 2ohm and 3 ohm then no. of different values of resistances that possible are
A6B4C10D8 - Question 2 - Select One
Two resistance wires on joining in parallel the resultant resistance is ohms 65 ohms. One of the wire breaks, the effective resistance is 2 ohms . The resistance of the broken wire is
A35 ohmB2 ohmC65 ohmD3 ohm - Question 3 - Select One
A 10 m long wire of resistance 15 ohm is connectd in series with a battery of emf 2V (no internal resistance) and a resistance of 5 ohm. The potential gradient along the wire is
A0.15Vm−1B0.45Vm−1C1.5Vm−1D4.5Vm−1
If a current of 3 A flows through a wire of resistance 8 ohms, calculate the potential difference applied across its ends.
View SolutionA student has been given 3 resistances of 3 ohms each.
(a) How should he connect these resistances so as to obtain a resultant resistance of 1 ohm ?
(b) Draw a labelled diagram to show the combination of these resistances.View SolutionHow should the two resistances of 2 ohms each be connected so as to produce an equivalent resistance of 1 ohm?
View SolutionA silver voltameter of resistance 2 ohm and a 3 ohm resistor are connected in series across a cell. If a resistance of 2 ohm is connected in parallel with the voltameter, then the rate of deposition of silver
View SolutionThe resistance of the four arms P,Q,R and S in a Wheatstone's bridge are 10ohm30ohm and 90ohm rerspectively. The e.m.f. and internal resistance of the cell are 7vo< and 5ohm respectively. If the galvanometer resistance is 50ohm, the current drawn for the cell will be
View Solution

