In a series resonant circuit, the ac voltage across resistance R, inductance L and capacitance C are VR=4V,VL=10V and VC=7V respectively. Find the voltage applied to the circuit.
To find the voltage applied to the series resonant circuit, we will use the given voltages across the resistor (R), inductor (L), and capacitor (C). The voltages are as follows:
- Voltage across the resistor, VR=4V
- Voltage across the inductor, VL=10V
- Voltage across the capacitor, VC=7V
1. Understand the Phase Relationships:
In a series resonant circuit:
- The voltage across the resistor VR is in phase with the current.
- The voltage across the inductor VL leads the current by 90∘ (or π2 radians).
- The voltage across the capacitor VC lags the current by 90∘ (or π2 radians).
2. Calculate the Effective Voltage Across Inductor and Capacitor:
Since VL and VC are 90∘ out of phase, we can find the effective voltage across them:
VLC=VL−VC=10V−7V=3V
3. Use the Pythagorean Theorem to Find the Total Voltage:
The total voltage V applied to the circuit can be calculated using the Pythagorean theorem, since VR is in phase with the current and VLC is perpendicular to it:
V=√V2R+V2LC
Substituting the known values:
V=√(4V)2+(3V)2=√16+9=√25=5V
4. Conclusion:
The voltage applied to the circuit is V=5V.
- Voltage across the inductor, VL=10V
- Voltage across the capacitor, VC=7V
In a series resonant circuit:
- The voltage across the resistor VR is in phase with the current.
- The voltage across the inductor VL leads the current by 90∘ (or π2 radians).
- The voltage across the capacitor VC lags the current by 90∘ (or π2 radians).
Since VL and VC are 90∘ out of phase, we can find the effective voltage across them:
VLC=VL−VC=10V−7V=3V
The total voltage V applied to the circuit can be calculated using the Pythagorean theorem, since VR is in phase with the current and VLC is perpendicular to it:
V=√V2R+V2LC
Substituting the known values:
V=√(4V)2+(3V)2=√16+9=√25=5V
The voltage applied to the circuit is V=5V.
Similar Questions
In an a.c. circuit the voltage across an inductance and resistance, joined in series, are 120 V and 160 V respectively. The total voltage applied across the combined circuit is …………..
View SolutionIn LCR circuit, the voltage across the terminals of a resistance, inductance & capacitance are 40V, 30V & 60V, then the voltage across the main source will be
View Solution
Knowledge Check
In a series resonant circuit, the ac, voltage across resistance R, inductance L and capacitance Care 5V, 10V and 10V, respectively. The ac. voltage applied to the circuit will be
A20 VB10 VC5 VD25 VIn a series resonant circuit, the AC voltage across resistance R, inductance L, and capacitance C are 5V,10V and 10V respectively. The AC voltage applied to the circuit will be
A20VB10VC5VD25VIn the given circuit the R.M.S values of voltages across the capacitor C, inductor L and resistor R1 are 12V, 10V and 5 V respectively. Then the peak voltage across R2 is
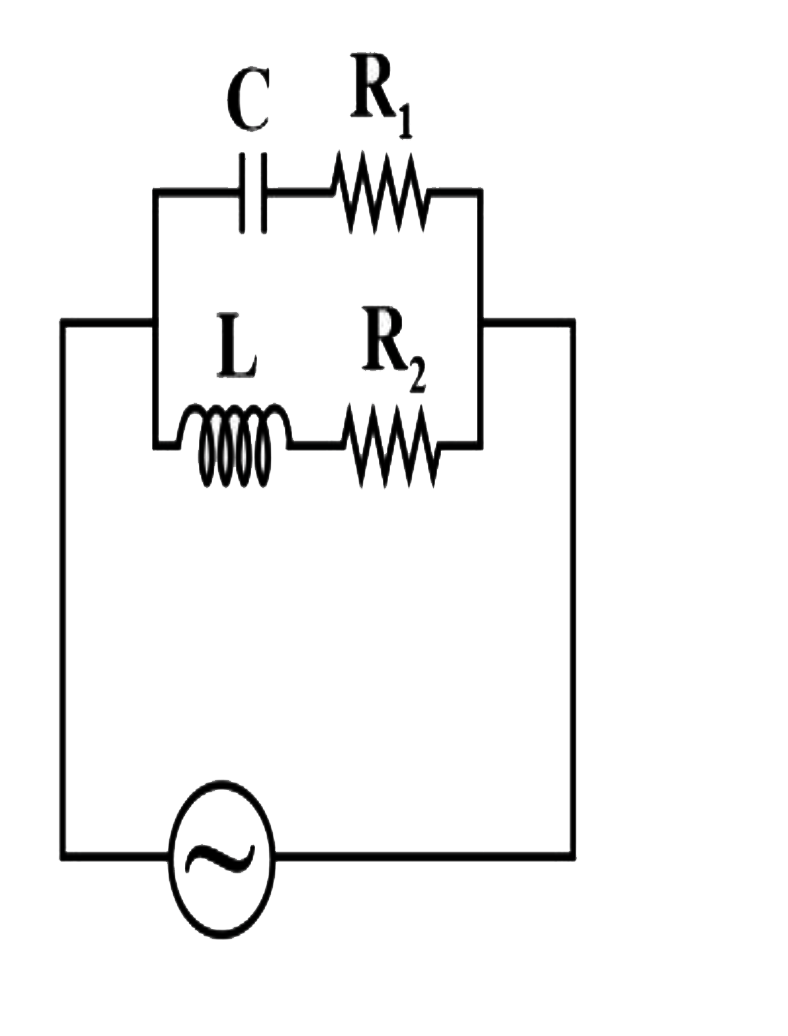 A7√2VB√69VC√138VDnone of these
A7√2VB√69VC√138VDnone of these
In series L−C−R circuit, voltage drop across resistance is 8V, across inductor is 6V and across capacitor is 12V. Then,
View SolutionThe potential differences across the resistance, capacitance and inductance are 80V, 40V and 100V respectively in an L-C-R circuit. The power factor of this circuit is
View SolutionThe potential differences across the resistance, capacitance and inductance are 80V,40V and 100V respectively in an L−C−R circuit. The power factor of this circuit is
View SolutionThe potential defferences across the resistance capacitance and indutance are 80 V, 40 V and 100 V respectively in an L-C-R circuit. The power factor of this circuit is
View SolutionThe adjoining figure shows an AC circuit with resistance R, inductance L and source voltage VS. Then
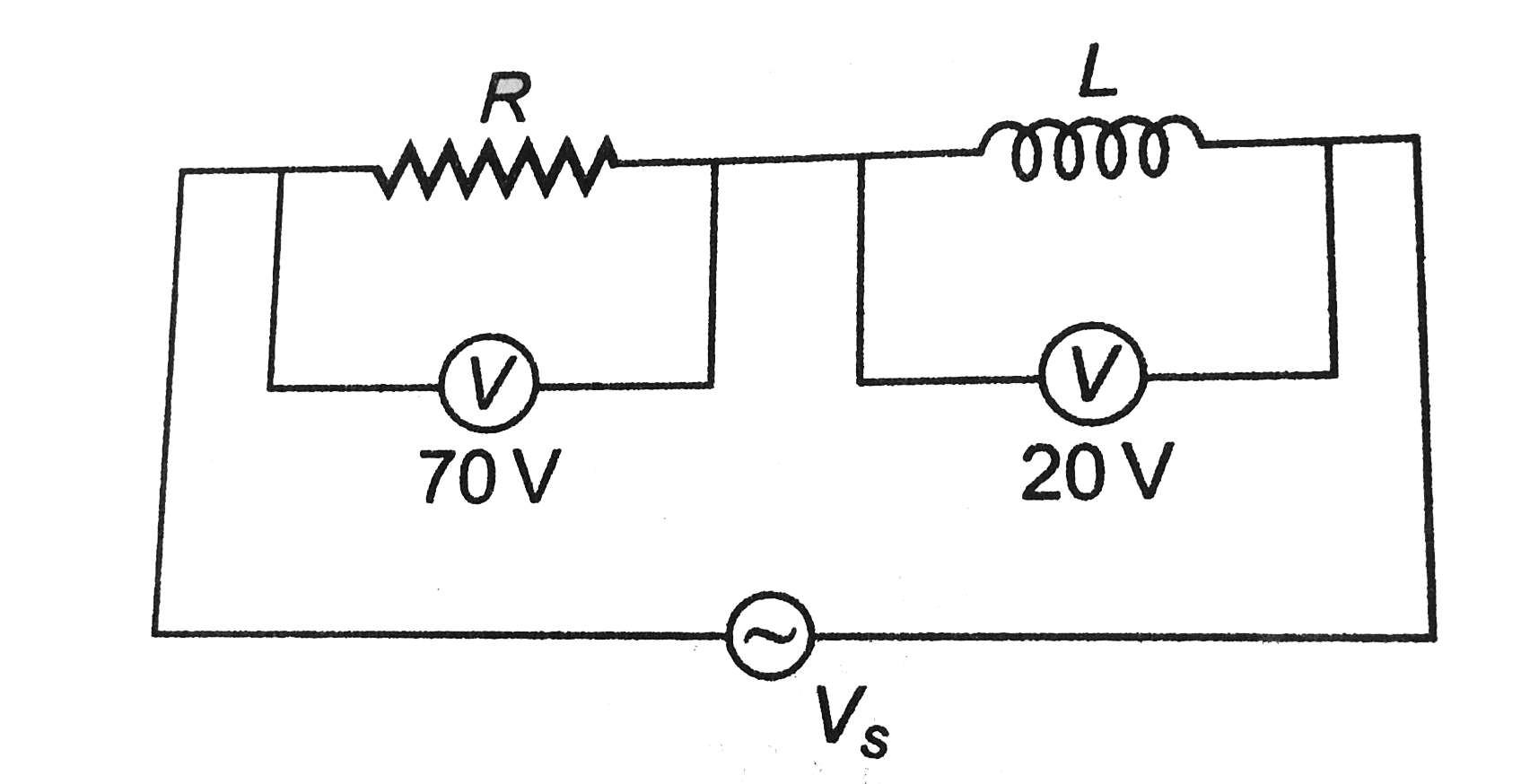
View Solution
