A beam of light converges at a point P. Now a convex lens of focal length 30 cm placed in the path of the convergent beam 12 cm from P. The point at which the beam converges now is
The correct Answer is:A
To solve the problem, we need to find the new point of convergence of the light beam after it passes through a convex lens placed 12 cm from point P. The focal length of the lens is given as 30 cm.
1. Identify the Given Values:
- Focal length of the lens, F=+30 cm (positive because it is a convex lens).
- Distance from the lens to point P, d=12 cm (this distance is measured towards the lens).
2. Determine the Object Distance (U):
- Since the lens is placed 12 cm from point P and the light converges at point P, we can consider the object distance U as negative (since the object is on the same side as the incoming light):
U=−12 cm
3. Use the Lens Formula:
The lens formula is given by:
1f=1v−1u
Rearranging gives:
1v=1f+1u
4. Substitute the Values into the Lens Formula:
Substituting f=30 cm and u=−12 cm:
1v=130+1−12
5. Calculate the Right-Hand Side:
To add the fractions, find a common denominator:
130=260,1−12=−560
Therefore:
1v=260−560=−360=−120
6. Solve for V:
Taking the reciprocal gives:
v=−20 cm
7. Interpret the Result:
The negative value of v indicates that the image is formed on the same side as the object (to the left of the lens). The distance from point P is:
Distance from P=12 cm−20 cm=−8 cm
This means the image is 8 cm to the left of point P.
Final Answer:
The new point at which the beam converges is 8 cm to the left of point P.
- Focal length of the lens, F=+30 cm (positive because it is a convex lens).
- Distance from the lens to point P, d=12 cm (this distance is measured towards the lens).
- Since the lens is placed 12 cm from point P and the light converges at point P, we can consider the object distance U as negative (since the object is on the same side as the incoming light):
U=−12 cm
The lens formula is given by:
1f=1v−1u
Rearranging gives:
1v=1f+1u
Substituting f=30 cm and u=−12 cm:
1v=130+1−12
To add the fractions, find a common denominator:
130=260,1−12=−560
Therefore:
1v=260−560=−360=−120
Taking the reciprocal gives:
v=−20 cm
The negative value of v indicates that the image is formed on the same side as the object (to the left of the lens). The distance from point P is:
Distance from P=12 cm−20 cm=−8 cm
This means the image is 8 cm to the left of point P.
The new point at which the beam converges is 8 cm to the left of point P.
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRAUMENTS Book:NARAYNAChapter:RAY OPTICS AND OPTICAL INSTRAUMENTS Exercise:EXERCISE - 1 (C.W)(REFRACTION THROUGH PRISM)
Explore 6 Videos
- RAY OPTICS AND OPTICAL INSTRAUMENTS Book:NARAYNAChapter:RAY OPTICS AND OPTICAL INSTRAUMENTS Exercise:EXERCISE - 1 (C.W)(DISPERSION BY A PRISM)
Explore 4 Videos
- RAY OPTICS AND OPTICAL INSTRAUMENTS Book:NARAYNAChapter:RAY OPTICS AND OPTICAL INSTRAUMENTS Exercise:EXERCISE - 1 (C.W)(REFRACTION THROUGH SPHERICAL SURFACES)
Explore 1 Video
- NUCLEI Book:NARAYNAChapter:NUCLEI Exercise:ASSERTION & REASON
Explore 5 Videos
- SEMI CONDUCTOR DEVICESBook:NARAYNAChapter:SEMI CONDUCTOR DEVICESExercise:Level-II (H.W)
Explore 36 Videos
Similar Questions
A beam of light converges at a point P. Now a convex lens is placed in the part of the converngent beam at 15 cm from P. At what point does a beam converge if the convex lens has a focad length 10 cm ?
View SolutionA beam of light converges at aoint P. Now a convex lens is placed in the path of the convergent beam at 15 cm from P. At what point does a beam converge if the convex lens has a focal length 10 cm?
View Solution
Knowledge Check
A beam of light converges at a point P. Now a concave lens of focal length -16 cm is placed in the path of the convergent beam 12 cm from P The point at which the beam converges now is
A6.86cm right side of the lensB6.86cm left side of the lensC48cm right side of the lensD48cm left side of the lensFigure given below shows a beam of light converging at point P. When a convex lens of focal length 16cm is introduced in the path of the beam at a place O shown by dotted line such that OP becomes the axis of the lens, the beam converges at a distance x from the lens. The value x will be equal to
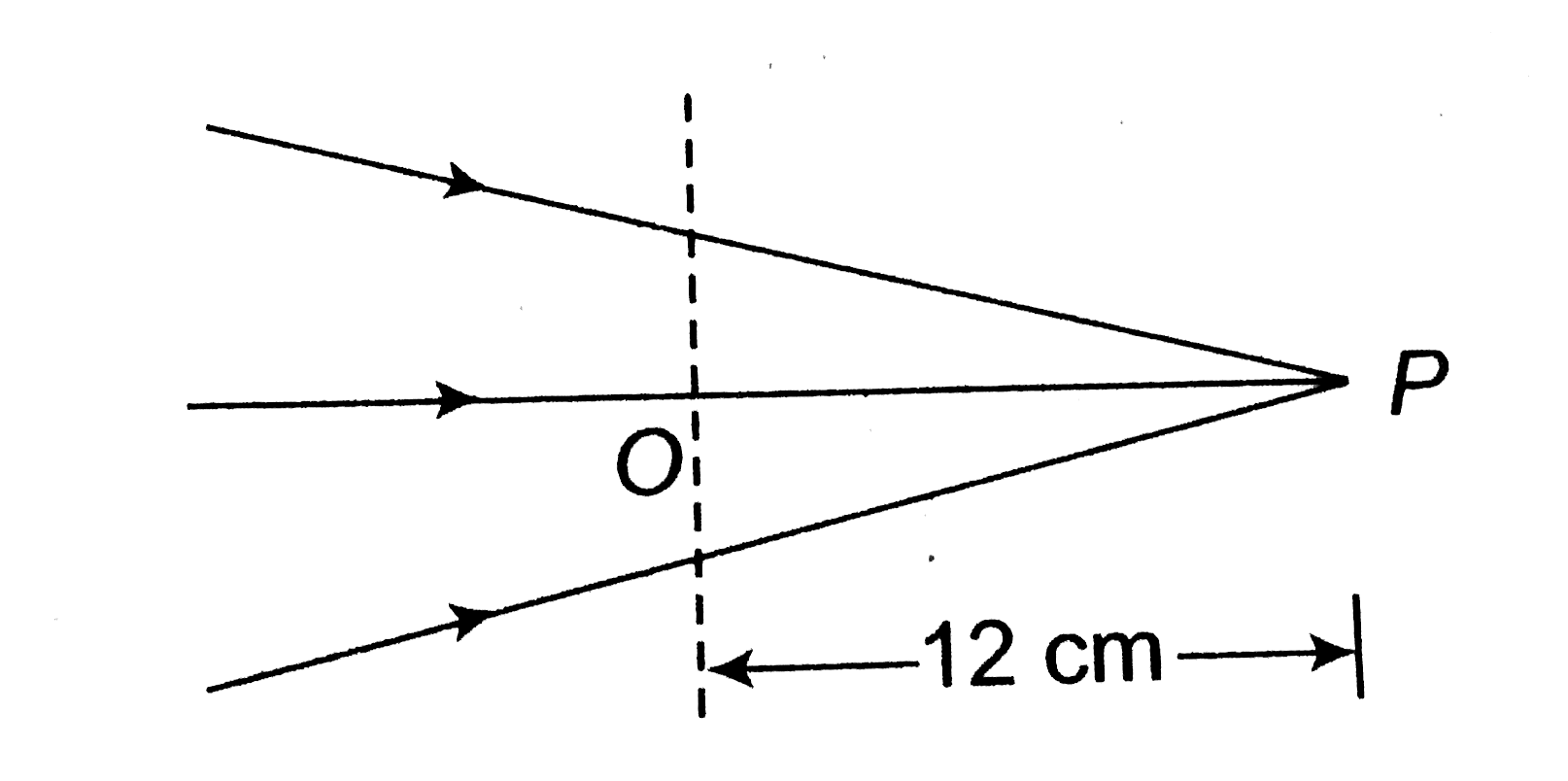 A12cmB24cmC36cmD48cm
A12cmB24cmC36cmD48cm
A beam of light converges of at a point P. Now a lens is placed in the path of the convergent beam 12 cm from P. At what point does the beam converge, if the lens is (a) a convex lens of focal length 20 cm, and (b) a concave lens of focal length 16 cm?
View SolutionA beam of light converges to a point P. A lens is placed in the path of the convergent beam 12 cm from P. At what point does the beam converge if the lens is (a) a convex lens of focal length 20 cm. (b) a concave lens of focal length 16 cm.
View SolutionA beam of light converges at a point P. A concave lens of focal length 16 cm is placed in the path of this beam 12 cm from P. Draw a ray diagram and find the location of the point at which the beam would now converge.
View SolutionA beam of light converges to a point P.A lens is placed in the path of the convergent beam 12cm from P. At what point does the beam converge if the lens is
(a) a convex lens of focal length 20cm. (b) a concave lens of focal length 16cm ?
View SolutionA beam of light converges to a point P. A lens is placed in the path of the covergent beam 12cm from P. At what point does the beam converge if the lens is
(a) a convex lens of focal length 20cm
(b) a concave lens of focal length 16cm ?
View SolutionA beam of light converges to a point on a screen S. A mirror is placed in front of the screen at a distance of 10cm form the screen. It is found that the beam now converges at a point 20cm in front of the mirror. Find the focal length of the mirror.
View Solution