Given M is the mass suspended from a spring of force constant. k.The dimensional formula for [M/k]1/2 is same as that for
frequency
time period
velocity
wavelength
The correct Answer is:B
Here [K] = force /length = ML0T−2
Hence [Mk]1/2=M0L0T−1
Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - UNITS AND MEASUREMENTSEXERCISE - EXERCISE -I (H.W)39videos
UNITS AND MEASUREMENTS
- BOOK - NARAYNACHAPTER - UNITS AND MEASUREMENTSEXERCISE - EXERCISE -II (C.W)28videos
UNITS AND MEASUREMENTS
- BOOK - NARAYNACHAPTER - UNITS AND MEASUREMENTSEXERCISE - C.U.Q -ADDITIONAL QUESTIONS27videos
UNITS AND MEASUREMENTS
- BOOK - NARAYNACHAPTER - TRANSMISSION OF HEATEXERCISE - LEVEL-II(C.W)27videos
TRANSMISSION OF HEAT
- BOOK - NARAYNACHAPTER - VECTORSEXERCISE - LEVEL-II (H.W)14videos
VECTORS
Similar Questions
A block of mass m suspended from a spring of spring constant k. Find the amplitude of S.H.M.
View SolutionTwo mass m1 and m2 are suspended from a massless spring of force constant k. When the masses are in equilibrium, m1 is removed without disturbing the system. Find the angular frequency and amplitude of oscillations.
View Solution
Knowledge Check
- Question 1 - Select One
A block of mass m is suspended by different springs of force constant shown in figure.
 AT1=T2=T4BT1=T2andT3=T4CT1=T2=T3DT1=T3 and T2=T4
AT1=T2=T4BT1=T2andT3=T4CT1=T2=T3DT1=T3 and T2=T4 - Question 2 - Select One
The frequency f of vibrations of a mass m suspended from a spring of spring constant k is given by f=Cmxky , where C is a dimensionnless constant. The values of xandy are, respectively,
A12,12B−12,−12C12,−12D−12,12 - Question 3 - Select One
Two masses M and m are suspended together by massless spring of force constant -k. When the masses are in equilibrium, M is removed without disturbing the system. The amplitude of oscillations.
AMgkBmgkC(M+m)gkD(M−m)gk

The period of oscillation of a mass M suspended from a spring of spring constant K is T. the time period of oscillation of combined blocks isView SolutionThe periodic time of a mass suspended by a spring (force constant k) is T. if the spring is cut in three equal pieces, what will be the force constant of each part? If the same mass be suspended from one piece, what will be the periodic time?
View SolutionThe period of oscillation of a mass M, hanging from a spring of force constant k is T. When additional mass m is attached to the spring, the period of oscillation becomes 5T/4. m/M =
View Solution
A mass m is suspended from a spring of force constant k and just touches another identical spring fixed to the floor as shown in the fig. The time period of small oscillations isView SolutionA mass m is suspended from a spring of force constant k and just touches another idential spring fixed to the floras shown in the figure. The time period of small oscillation is
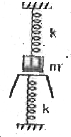 View Solution
View Solution

