A body falls from a height 'h'. In absence of air resistance time of descent of body is
√2hg
√2hg−a
√2hg±a
hg
The correct Answer is:A
To find the time of descent of a body falling from a height h in the absence of air resistance, we can use the equations of motion. Here's a step-by-step solution:
Step 1: Identify the known values
- Initial velocity u=0 (the body is initially at rest)
- Acceleration due to gravity g (approximately 9.81m/s2)
- Distance fallen s=h
Step 2: Use the equation of motion
We can use the second equation of motion:
s=ut+12at2
In this case, since the body is falling, we can substitute:
- s=h
- u=0
- a=g
So the equation becomes:
h=0⋅t+12gt2
This simplifies to:
h=12gt2
Step 3: Rearrange the equation to solve for t
To isolate t2, we multiply both sides by 2:
2h=gt2
Now, divide both sides by g:
t2=2hg
Step 4: Take the square root to find t
Now, taking the square root of both sides gives us the time of descent td:
td=√2hg
Final Result
Thus, the time of descent of the body falling from a height h in the absence of air resistance is:
td=√2hg
---
- Initial velocity u=0 (the body is initially at rest)
- Acceleration due to gravity g (approximately 9.81m/s2)
- Distance fallen s=h
We can use the second equation of motion:
s=ut+12at2
In this case, since the body is falling, we can substitute:
- s=h
- u=0
- a=g
h=0⋅t+12gt2
This simplifies to:
h=12gt2
To isolate t2, we multiply both sides by 2:
2h=gt2
Now, divide both sides by g:
t2=2hg
Now, taking the square root of both sides gives us the time of descent td:
td=√2hg
Thus, the time of descent of the body falling from a height h in the absence of air resistance is:
td=√2hg
Similar Questions
Two bodies of masses M and m are allowed to fall from the same height . If air resistance for each body be same , will the two bodies reach the ground simultaneously ?
View SolutionTwo bodies of masses M and m are allowed to fall from the same height. If air resistance for each body be same, will the two bodies reach the ground simultaneously ?
View Solution
Knowledge Check
- Question 1 - Select One
A body falls from a height H. Its velocity (v)-distance (x) graph is
A B
B C
C D
D
- Question 2 - Select One
A body falls freely from a height 'h' after two seconds if acceleration due to gravity is reversed the body
AContinues to fall downBfalls down retardation & goes up again with accelerationCfalls down with uniform velocityDraises up with acceleration - Question 3 - Select One
A body of mass m falls from a height h onto the pan of a spring balance. The masses of the pan and spring are negligible. The force constant of the spring is k. The body sticks to the pan and oscillates simple harmonically. The amplitude of oscillation is
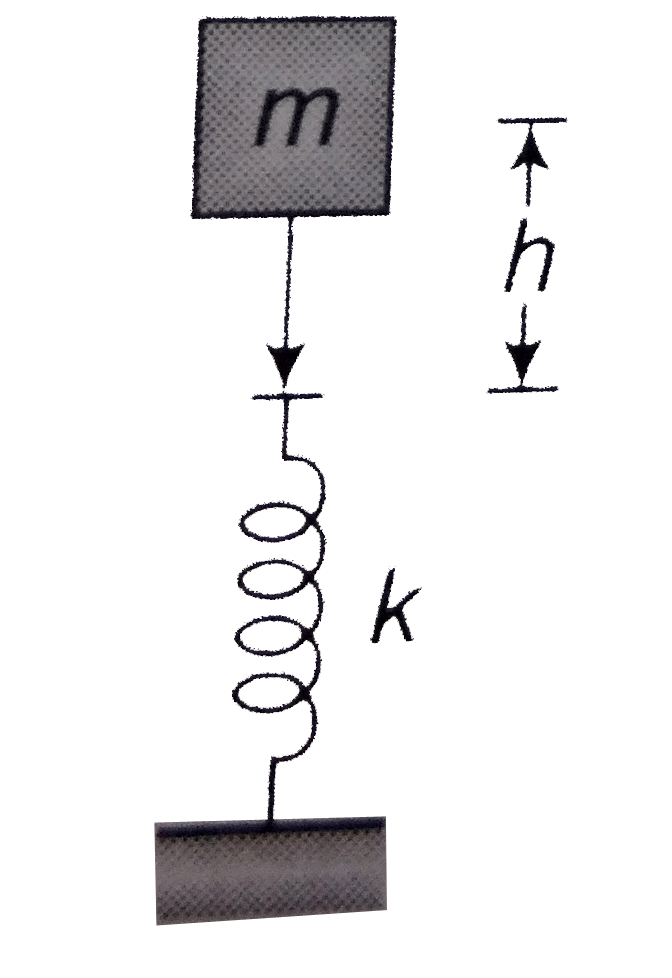 AmgkBmgk√1+2hkmgCmgk(1+√1+2hkmg)Dmgk√1+(2hkmg)−1
AmgkBmgk√1+2hkmgCmgk(1+√1+2hkmg)Dmgk√1+(2hkmg)−1
i) If the net force acting on a body is zero, will it remain necessarily at rest?
(ii) Two bodies of mass M and m are allowed to fall from the same height. If air resistance for each body be same, will the two bodies reach the ground simultaneously?View Solution" A body falls freely from a height 'h' its average velocity when it reaches the earth is "
View SolutionAssertion: Two bodies of masses M and m(M>m) are allowed to fall from the same height if the air resistance for each be the same then both the bodies will reach the earth simultaneously.
Reason: For same air resistance, acceleration of both the bodies will be same.View SolutionAssertion: Two bodies of masses M and m(M>m) are allowed to fall from the same height if the air resistance for each be the same then both the bodies will reach the earth simultaneously.
Reason: For same air resistance, acceleration of both the bodies will be same.View SolutionA body of mass m falls from height h0 on the ground. If e is the coefficient of restitution between body and the ground, then the height upto which it rises after ten collisions is
View Solution

