particle is executing the motion x=Acos(ωt−θ) The maximum velocity of the particle is
Aωcosθ
Aω
Aωsinθ
None
The correct Answer is:B
x=Acos(ωt−θ)⇒v=−Aωsin(ωt−θ)
vmax=Aω
Topper's Solved these Questions
- OSCILLATIONSBOOK - NARAYNACHAPTER - OSCILLATIONSEXERCISE - EXERCISE - II (C.W)
27 Videos
- OSCILLATIONSBOOK - NARAYNACHAPTER - OSCILLATIONSEXERCISE - EXERCISE - II (H.W)
19 Videos
- OSCILLATIONSBOOK - NARAYNACHAPTER - OSCILLATIONSEXERCISE - EXERCISE - I (C.W)
63 Videos
- NEWTONS LAWS OF MOTIONBOOK - NARAYNACHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - PASSAGE TYPE QUESTION
6 Videos
- PHYSICAL WORLDBOOK - NARAYNACHAPTER - PHYSICAL WORLDEXERCISE - C.U.Q
10 Videos
Similar Questions
The displacement - time graph of a particle executing SHM is as shown in the figure. The maximum velocity of the particle is
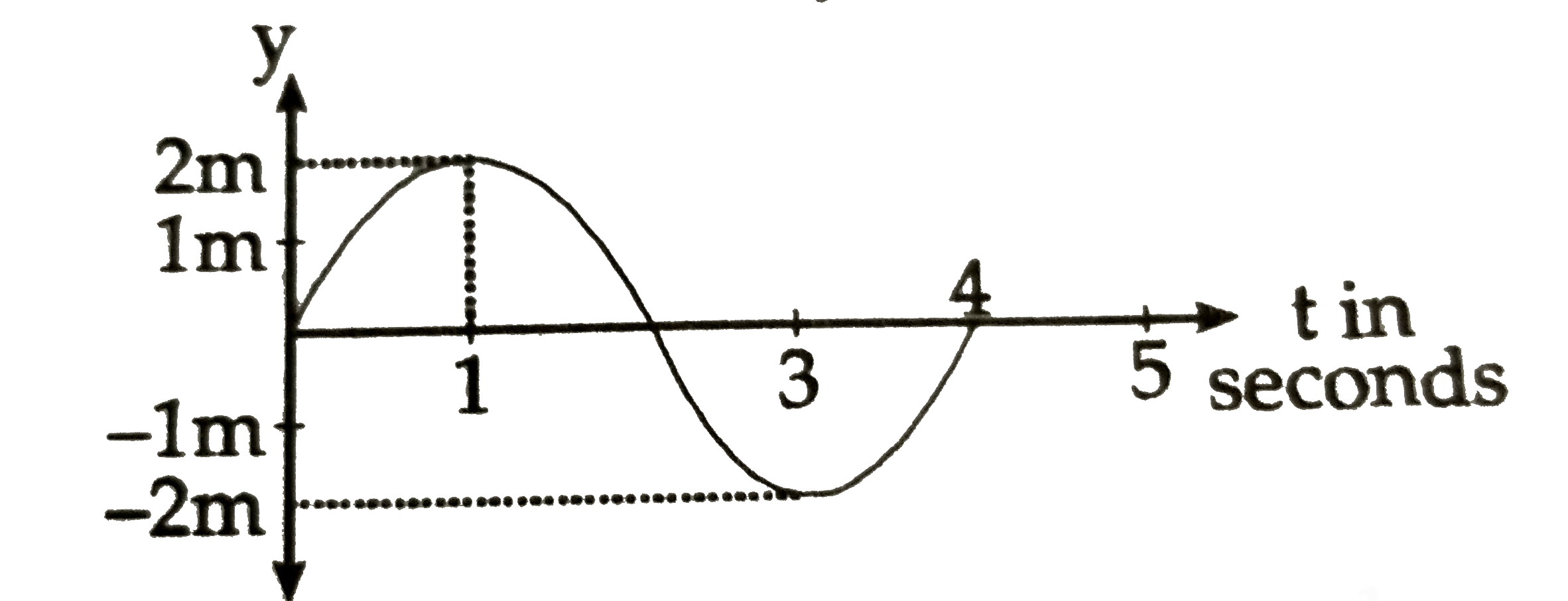
View SolutionA particle is executing simple harmonic motion with maximum velocity of 20 cm/s. Calculate the average velocity during its motion from one extreme to another.
View Solution
Knowledge Check
The displacement of a particle is represented by the equation y=3cos(π4−2ωt).
The motion of the particle isAsimple harmonic with period 2π/ωBsimple harmonic with period π/ωCperiodic but not simple harmonicDnon-periodThe displacemtn of a particle is represented by the equation y=3cos(π4−2ωt).
The motion of the particle isAsimple harmonic with period 2π/ωBsimple harmonic with periodπ/ωCperiodic but not simple harmonicDnon-periodicA particle is executing SHM acccording to the equation x=A cos ωt. Average speed of theparticle during the interval 0≤t≤π6ω
A√3Aω2B√3Aω4C3AωπD3Aωπ(2−√3)
A particle is executing SHM given by x=Asin(πt+ϕ). The initial displacement of particle is 1cm and its initial velocity is πcm/sec. Find the amplitude of motion and initial phase of the particle.
View SolutionA particle is executing SHM, whose equation is given below
x=Acos(ωt+ϕ). If t=0 , particle is mean position going towards positive x-direction then ϕ is equal to
View Solutionparticle is executing S.H.M. If its amplitude is 2 m and periodic time 2 seconds , then the maximum velocity of the particle will be
View SolutionAssertion: Total energy of a particle executing S.H.M. · is maximum at mean position .
Reason: The velocity of the particle executing S.H.M. is maximum at mean position.
View SolutionA particle executing simple harmonic motion with an amplitude A and angularr frequency ω. The ratio of maximum acceleration to the maximum velocity of the particle is
View Solution
