The following data were obtained at a certain temperature for the decomposition of in contact with catalyst.
The order of reaction is
2
3
1
4
The correct Answer is:A
To determine the order of the reaction for the decomposition of ammonia (NH₃) based on the given data, we can follow these steps:
Step 1: Understand the relationship between half-life and pressure
The half-life (t₁/₂) of a reaction is related to the initial concentration (or pressure in this case) of the reactants. For a reaction of order n, the relationship can be expressed as:
t1/2∝1Pn−10
Where:
- t1/2 is the half-life,
- P0 is the initial pressure,
- n is the order of the reaction.
Step 2: Analyze the given data
From the problem, we have the following data:
- For P0=25 mm, t1/2=1.82 minutes.
- For P0=50 mm, t1/2=0.91 minutes.
Step 3: Compare the half-lives
Notice that when the pressure doubles from 25 mm to 50 mm, the half-life decreases from 1.82 minutes to 0.91 minutes. This indicates an inverse relationship between half-life and pressure.
Step 4: Establish the relationship
Using the relationship established in Step 1, we can set up the following equations based on the two sets of data:
1. For P0=25 mm:
t1/2∝125n−1(1)
2. For P0=50 mm:
t1/2∝150n−1(2)
Step 5: Set up the ratio
Since the half-lives are inversely proportional to the pressures raised to the power of n−1, we can set up the ratio of the two half-lives:
t1/2(25)t1/2(50)=50n−125n−1
Substituting the half-life values:
1.820.91=50n−125n−1
Step 6: Simplify the equation
Calculating the left side:
2=(5025)n−1=2n−1
Step 7: Solve for n
Taking logarithm or equating the powers:
2=2n−1
This implies:
n−1=1⇒n=2
Conclusion
The order of the reaction is 2.
---
The half-life (t₁/₂) of a reaction is related to the initial concentration (or pressure in this case) of the reactants. For a reaction of order n, the relationship can be expressed as:
- t1/2 is the half-life,
- P0 is the initial pressure,
- n is the order of the reaction.
From the problem, we have the following data:
- For P0=50 mm, t1/2=0.91 minutes.
Notice that when the pressure doubles from 25 mm to 50 mm, the half-life decreases from 1.82 minutes to 0.91 minutes. This indicates an inverse relationship between half-life and pressure.
Using the relationship established in Step 1, we can set up the following equations based on the two sets of data:
t1/2∝125n−1(1)
t1/2∝150n−1(2)
Since the half-lives are inversely proportional to the pressures raised to the power of n−1, we can set up the ratio of the two half-lives:
Calculating the left side:
Taking logarithm or equating the powers:
The order of the reaction is 2.
Topper's Solved these Questions
Similar Questions
The following data were obtained at a certain temperature for the decomposition of ammonia in contact with tungsten:
p (m m Hg) 50 100 200
Relative t1/2 3.64 1.82 0.91
Find the order of the reaction.
View SolutionThe following data were obtained at a certain temperature for the decomposition of ammonia in contact with tungsten:
p (m m Hg) 50 100 200
Relative t1/2 3.64 1.82 0.91
Find the order of the reaction.
View Solution
Knowledge Check
For the decompoistion of HI at 1000K(2HI→H2+I2), following data were obtained:
∣∣ ∣ ∣ ∣ ∣∣[HI](M)Rate of decomposition of HI(molL−1s−1)0.12.75×10−80.211×10−80.324.75×10−8∣∣ ∣ ∣ ∣ ∣∣
The order of reaction isA1B2C0D1.5The following data were obtained during the first order thermal
decomposition of SO2Cl2 at a constant volume SO2Cl2(g)→SO2(g)+Cl2(g)
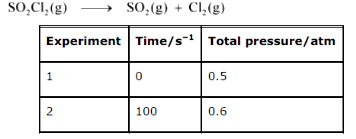
Calculate the rate of the reaction when total pressure is 0.65 atmA0.35atmS−1B2.235×10−3atmS−1C7.8×10−4atms−1D1.55×10−4atmS−1The following data were obtained for the thermal decomposition of nitrous oxide on finely divided gold :
t (minutes)020406080Concentration of N2O(molL−1)0.100.080.060.040.02
The order of reaction is :AzeroB1C2D3
The following data were obtained during the first order thermal decomposition of SO2Cl2 at a constant volume.
SO2Cl2(g)→SO2(g)+Cl2(g)

What is the rate of reaction when total pressure of 0.65 atm?
View SolutionThe following data were obtained during the first order thermal
decomposition of SO2Cl2 at a constant volume SO2Cl2(g)→SO2(g)+Cl2(g)

Calculate the rate of the reaction when total pressure is 0.65 atm
View SolutionThe following data were obtained during first order thermal decomposition of SO2(Cl2) at a constant volume.
SO2Cl2(g)→SO2(g)+Cl2(g)

Calculate the rate of the reaction when the total pressure is 0.65 atm.
View SolutionThe following data were obtained during the first order decomposition of SO2Cl2 at a constant volume:
SO2Cl2(g)→SO2(g)+Cl2(g)

Calculate the rate constant.
View SolutionAt certain temperature, the half life period in the thermal decomposition of a gaseous substance as follows :
P (mm Hg) 500 250
t1/2 (in min) 235 950
Find the order of reaction [Given log (23.5)=1.37, log (95)=1.97]
View Solution
