At time t = 0 second, voltage of an A.C. Generator starts from 0V and becomes 2V at time t=1100π second. The voltage keeps on increasing up 100 V, after wihich it starts to decrease. Find the frequency of the Generator.
100 Hz
1 Hz
2 Hz
5 Hz
The correct Answer is:B
To find the frequency of the A.C. generator based on the information provided, we can follow these steps:
Step 1: Understand the Voltage Function
The voltage V(t) of an A.C. generator can be expressed as:
V(t)=V0sin(ωt)
where V0 is the maximum voltage (amplitude) and ω is the angular frequency.
Step 2: Identify Maximum Voltage
From the problem, we know that the voltage increases to a maximum of 100V. Therefore, we have:
V0=100 V
Step 3: Determine the Time at a Given Voltage
We are given that at time t=1100π seconds, the voltage is 2V. We can substitute this into our voltage equation:
2=100sin(ω⋅1100π)
Step 4: Solve for sin(ωt)
Rearranging the equation gives:
sin(ω⋅1100π)=2100=150
Step 5: Use the Small Angle Approximation
Since sin(θ) is small, we can use the approximation sin(θ)≈θ for small angles. Thus, we have:
ω⋅1100π≈150
Step 6: Solve for Angular Frequency ω
Now, we can solve for ω:
ω≈150⋅100π=2π1=2π rad/s
Step 7: Calculate Frequency f
The frequency f is related to angular frequency by the formula:
ω=2πf
Substituting our value of ω:
2πf=2π⇒f=1 Hz
Conclusion
The frequency of the A.C. generator is:
1 Hz
The voltage V(t) of an A.C. generator can be expressed as:
V(t)=V0sin(ωt)
where V0 is the maximum voltage (amplitude) and ω is the angular frequency.
From the problem, we know that the voltage increases to a maximum of 100V. Therefore, we have:
V0=100 V
We are given that at time t=1100π seconds, the voltage is 2V. We can substitute this into our voltage equation:
2=100sin(ω⋅1100π)
Rearranging the equation gives:
sin(ω⋅1100π)=2100=150
Since sin(θ) is small, we can use the approximation sin(θ)≈θ for small angles. Thus, we have:
ω⋅1100π≈150
Now, we can solve for ω:
ω≈150⋅100π=2π1=2π rad/s
The frequency f is related to angular frequency by the formula:
ω=2πf
Substituting our value of ω:
2πf=2π⇒f=1 Hz
The frequency of the A.C. generator is:
1 Hz
Topper's Solved these Questions
Similar Questions
Knowledge Check
A generator produces a time varying voltage given by V=240sin120t, where t is in second. The rms voltage and frequency are
A170V and 19HzB240V and 60HzC170V and 60HzD120V and 19HzA alternating voltage varies with time (t) as V=100sin(50πt). The rms voltage and the frequency respectively, are
A100√2V,50HzB100V,25HzC100√2V,50HzD100√2V,25HzThe voltage of an a.c. source varies with time according to relation
E=120 sin 100πtcos100πt thenAthe peak voltage of source is 120vBthe peak voltage = 60 vCthe peak voltage = (120/√2)vDfrequency of source voltage is 100 Hz
A periodic voltage V varies with time t as shown in figure. T is the time period. Find the rms value of the voltage.
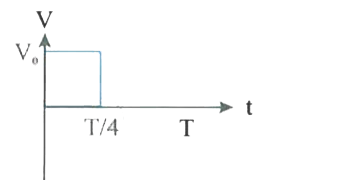
View SolutionA periodic voltage V varies with time t as shown in figure. T is the time period. Find the runs value of the voltage.

View SolutionEquation of emf of a generator is V=282sin100πt volt. Internal resistance of generator is 2000Ω. It is connected as shown in Fig. Find the frequency of generator and impedance of circuit.
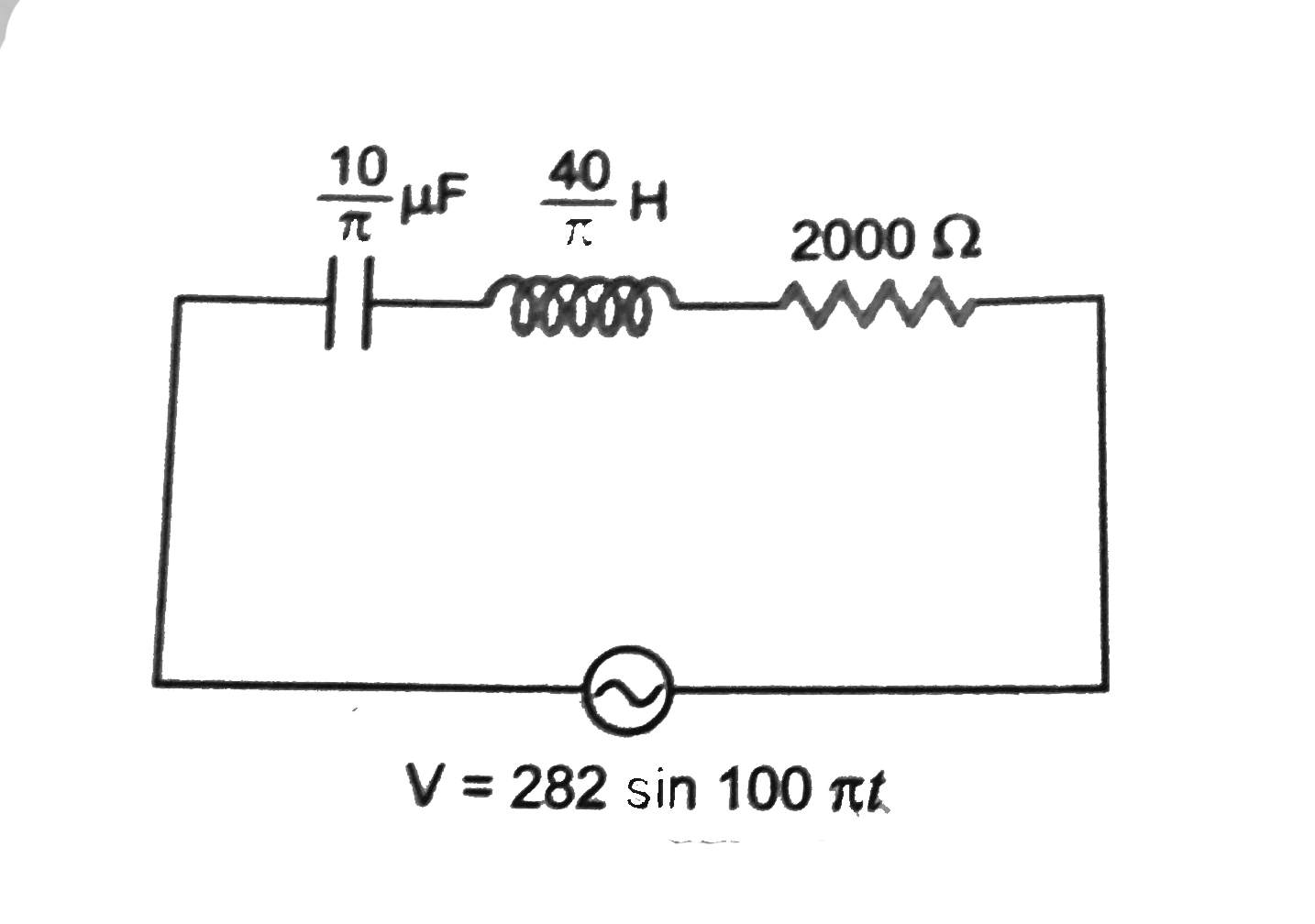
View SolutionA generator produces a voltage that is given by V=240 sin 120t, where t is in seconds. The frequency and rms voltage are (1)
View SolutionThe voltage of A.C source varies with tiem according equation. V=120sin100πtcos100πt.Then the frequency of source is
View Solution
