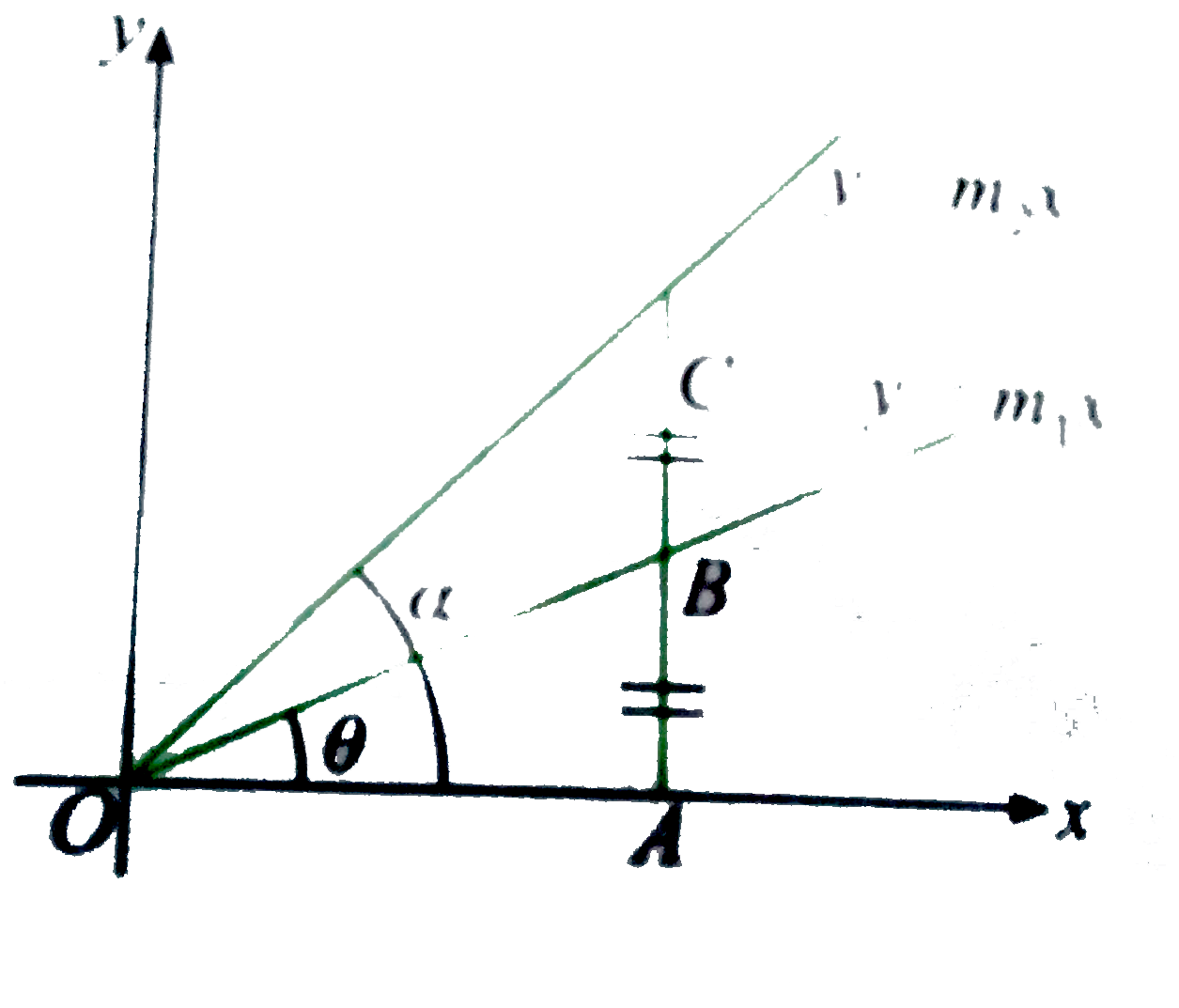
Through a point A on the x-axis, a straight line is drawn parallel to the y-axis so as to meet the pair of straight lines ax2+2hxy+by2=0 at B and C. If AB=BC, then h2=4ab (b) 8h2=9ab 9h2=8ab (d) 4h2=ab
h2=4ab
8h2=9ab
9h2=8ab
4h2=ab
The correct Answer is:2
Given AB=BC. Also , m1+m2=2hbandm1m2=ab
tanθ=ABOA=m1
and m2=tanα=2ABOA=2m1
∴m2m1=2
or m2+m1m2−m1=2+12−1=3
or 2h/b√(4h2/b2)−(4a/b)=3
or 4h2b2⋅b24(h2−ab)=9
or 9h2−9ab=h2
or 8h2=9ab
Topper's Solved these Questions
- PAIR OF STRAIGHT LINESBOOK - CENGAGECHAPTER - PAIR OF STRAIGHT LINESEXERCISE - Exercise (Multiple)
9 Videos
- PAIR OF STRAIGHT LINESBOOK - CENGAGECHAPTER - PAIR OF STRAIGHT LINESEXERCISE - Exercise (Comprehension)
6 Videos
- PAIR OF STRAIGHT LINESBOOK - CENGAGECHAPTER - PAIR OF STRAIGHT LINESEXERCISE - Exercise 3.2
7 Videos
- MONOTONOCITY AND NAXINA-MINIMA OF FUNCTIONSBOOK - CENGAGECHAPTER - MONOTONOCITY AND NAXINA-MINIMA OF FUNCTIONSEXERCISE - Comprehension Type
6 Videos
- PARABOLABOOK - CENGAGECHAPTER - PARABOLAEXERCISE - Question Bank
21 Videos
Similar Questions
Through a point A on the x-axis, a straight line is drawn parallel to the y-axis so as to meet the pair of straight lines ax2+2hxy+by2=0 at B and C. If AB=BC, then h2=4ab (b) 8h2=9ab 9h2=8ab (d) 4h2=ab
View SolutionIf the pair of straight lines x2−y2+2x+1=0 meet the y-axis in A and B, AB equals to
View Solution
Knowledge Check
If a,b,c are in H.P then the straight line xa+yb+1c=0 always passes through the fixed point whose coordinates are
A(-1,-2)B(-1,2)C(1,-2)D(1,−1/2)The curve (xa)n+(yb)n =2 touches the straight line xa+yb=2 at the point (a,b) then the value of n is
A2B3C4Dany real number
Find the equation of a straight line drawn perpendicular to the line xa+yb=1 through the point where it meets the y-axis.
View SolutionThe straight lines ax2+2hxy+by2=0 have the same pair of bisector as those of the lines given by a2x2+2h(a+b)xy+b2y2=0
View SolutionThe equation of the straight line joining the point (a,b) to the point of intersection of the lines xa+yb=1 and xb+ya=1 is a2y−b2x=ab(a−b)
View SolutionXY is drawn parallel to the base BC of a ABC cutting AB at X and AC at Y . If AB=4 BX and YC=2cm , then AY= 2cm (b) 4cm (c) 6cm (d) 8cm
View SolutionA straight line is drawn through the centre of the circle x2+y2−1ax=0 parallel to the straight line x+2y=0 and intersection the circle at AandB. Then the area of AOB is
View SolutionIf the straight line xa+yb=1 passes through the points (8,9) and (12,-15) find the values of a and b.
View Solution
