Find the number of solutions to 7|x|(|1−|x∣∣)=1.
Video Solution
Text Solution
We have 7|x|(|1−|x∣∣)=1
or |1−|x∣∣=7−|x|
To find the number of solutions to the equation, we draw the graph of y=|1−|x∣∣andy=7−|x| and find number of points of intersection. The graphs of both the functions are as intersection.
The graphs of both the functions are as shown in the following figure.
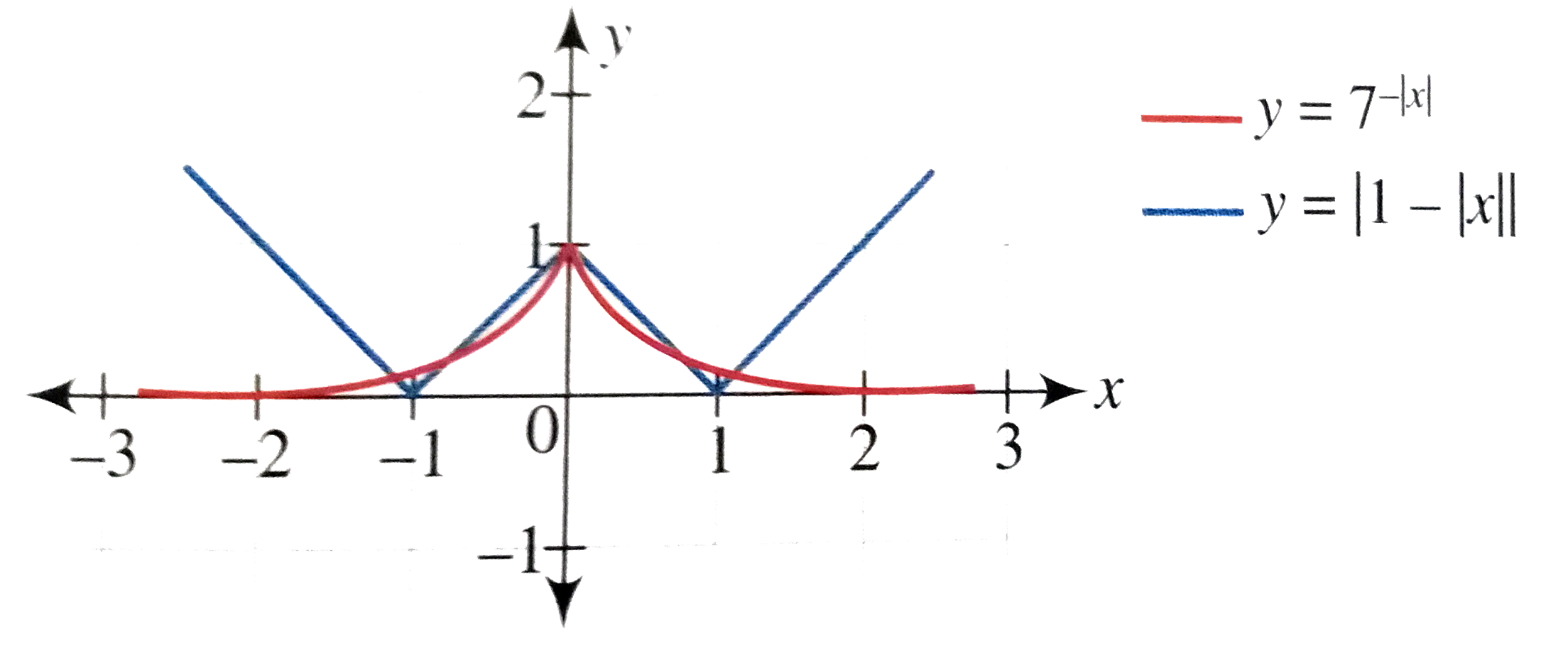
From the graph, the number of solutions is 5.
|
Topper's Solved these Questions
- GRAPHICAL TRANSFORMATIONSBOOK - CENGAGECHAPTER - GRAPHICAL TRANSFORMATIONSEXERCISE - Exercise
22 Videos
- GRAPH OF INVERSE TRIGONOMETRIC FUNCTIONSBOOK - CENGAGECHAPTER - GRAPH OF INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise
18 Videos
- GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSBOOK - CENGAGECHAPTER - GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSEXERCISE - Exercise
13 Videos
Similar Questions
Knowledge Check
Number of solutions of |x−1|cosx is
A2B3C4DNone of theseNumber of solutions of |x−1|=cosx is
A2B3C4DNone of these
The number of solutions of x2+|x−1|=1 is
View SolutionLet f(x)=x+sinx. Find the number of solutions of f(x)=f−1(x)
View SolutionFind the number of solutions of 4{x}=x+[x].
View SolutionThe graph of the function y=f(x) is shown.

Find the number of solutions to the equation ||f(x)|−1|=12.
View SolutionFind the number of solution of 2tan−1(tanx)=6−x
View SolutionFind the number of solution of 2tan−1(tanx)=6−x.
View Solution
