Find the sumber of solutions of equation (2x−3)2x=1.
We have (2x−3)2x=1
or2x−3=2−x
To find the number of roots of the above equation, we need to find the number of points of intersection of y=2x−3andy=2−x.
The graphs of functions are as shown in the following figure.
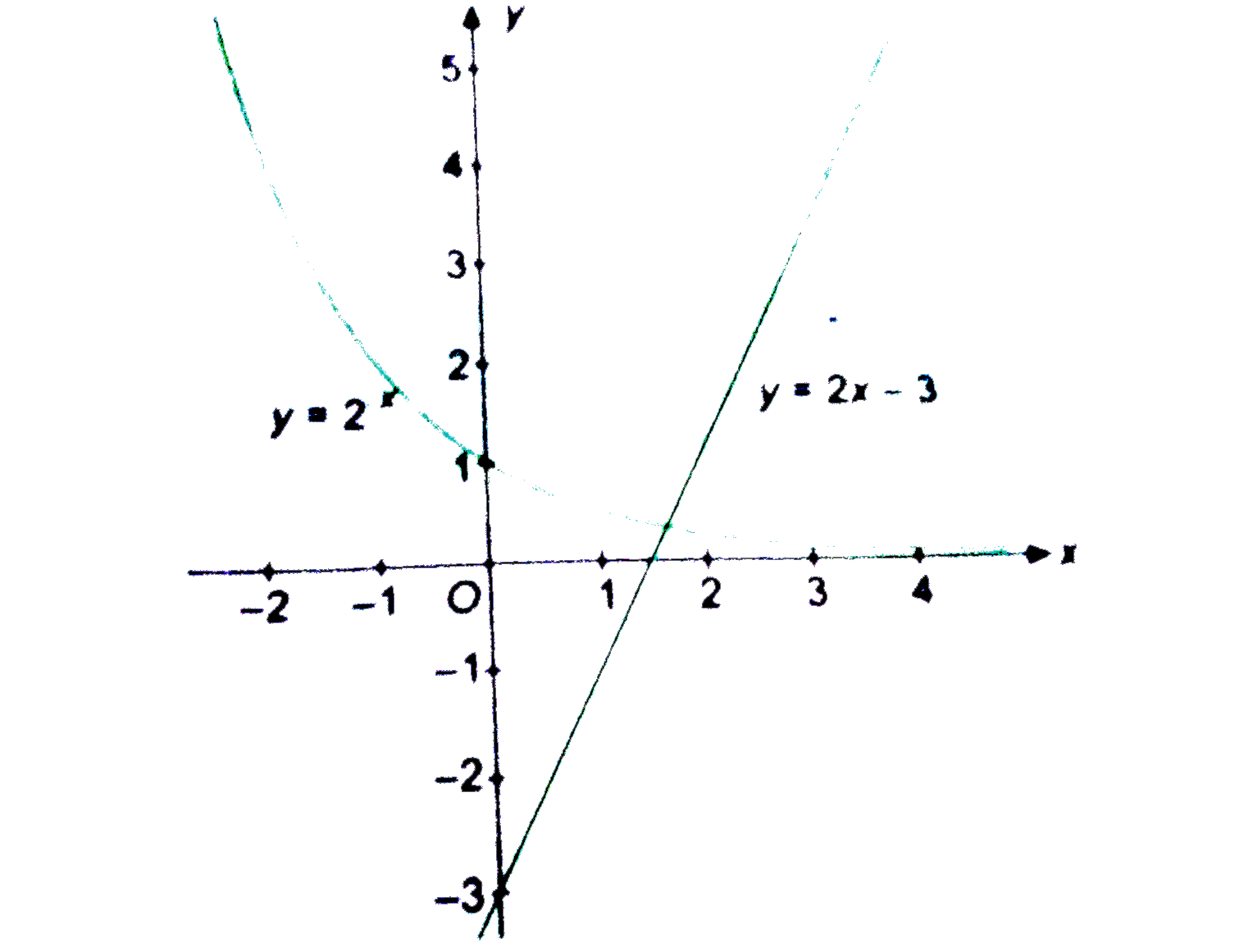
From the figure, graphs intersect at only one point.
Hence, there is only one solution of the given equation.
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - LOGARITHM AND ITS PROPERTIESEXERCISE - Exercise 1.16videos
LOGARITHM AND ITS PROPERTIES
- BOOK - CENGAGECHAPTER - LOGARITHM AND ITS PROPERTIESEXERCISE - Exercise 1.29videos
LOGARITHM AND ITS PROPERTIES
- BOOK - CENGAGECHAPTER - LOGARITHM AND ITS APPLICATIONS EXERCISE - Subjective Type9videos
LOGARITHM AND ITS APPLICATIONS
- BOOK - CENGAGECHAPTER - MATHMETICAL REASONINGEXERCISE - JEE Previous Year10videos
MATHMETICAL REASONING
Similar Questions
Find the number of solutions of equation (2x−3)2x=1
View SolutionFind the number of solutions of the equations
(sinx−1)3+(cosx−1)3+(sinx)3=(2sinx+cosx−2)3 in (0,2π).View SolutionFind the number of solutions of the equations
(sinx−1)3+(cosx−1)3+(sinx)3=(2sinx+cosx−2)3 in [0,2π].View SolutionFind the number of solutions of the equation x21−|x−2|=1, graphically.
View SolutionFind the number of real solutions of the equation (cosx)5+(sinx)3=1 in the interval [0,2π]
View SolutionFind the number of solutions of the equation sinx=x2+x+1.
View SolutionFind the number of solution of the equation 2x=3π(1−cosx).
View SolutionFind the number of solution of the equation sinx=x2+x+1.
View SolutionFind the number of solutions of the equation 2cos3x(3–4sin2x)=1 in [0,2π]
View SolutionFind the number of solution of the equation
x3+2x+cosx+tanx=0∈(−π2,π2)View Solution

