If the tangent to the curve $y = e^{x}$ at a point $(c, e^{c})$ and the normal to the parabola, $y^{2} = 4 x$ at the point (1,2) intersect at the same point on the x-axis then the value of c is ____________.

Video Solution

Answer

Step by step video & image solution for If the tangent to the curve y= e^x at a point (c, e^c) and the normal to the parabola, y^2 = 4x at the point (1,2) intersect at the same point on the x-axis then the value of c is ____________. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Topper's Solved these Questions

Knowledge Check

The tangent to the curve $y = e^{2 x}$ at the point (0, 1) meets the x-axis at
A $(0, 1)$
B $(- \frac{1}{2}, 0)$
C $(2, 0)$
D $(0, 2)$
If normals to parabola $y^{2} = 4 x$ at points A, B, C intersect at (11, 0), then

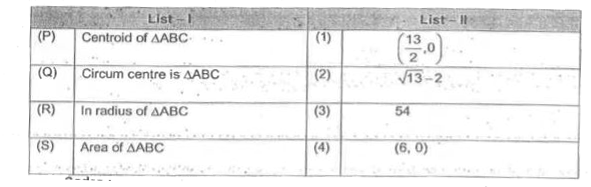A $\begin{matrix} P & Q & R & S 2 & 1 & 4 & 3 \end{matrix}$
B $\begin{matrix} P & Q & R & S 4 & 1 & 2 & 4 \end{matrix}$
C $\begin{matrix} P & Q & R & S 4 & 1 & 2 & 3 \end{matrix}$
D $\begin{matrix} P & Q & R & S 2 & 4 & 1 & 3 \end{matrix}$
The tangent to the curve $y = e^{2 x}$ at the point (0,1) meets X-axis at
A $(0, 1)$
B $(- \frac{1}{2}, 0)$
C $(2, 0)$
D $(0, 2)$