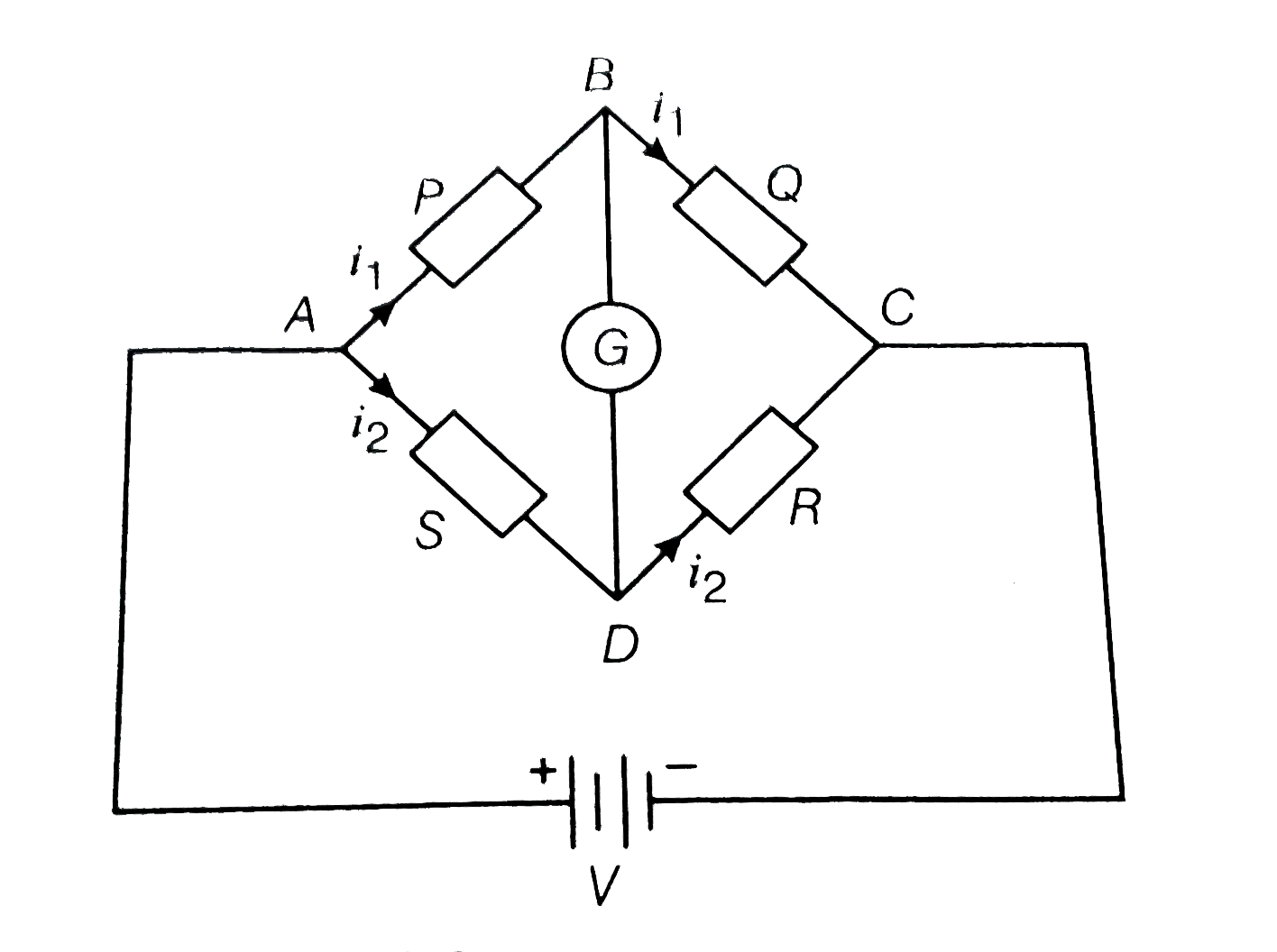
BALANCED WHEATSTONE BRIDGE
The correct Answer is:If deflection is galvenometer is zero then
potential difference across AB=potential difference across AD
I1P=I2R .........(i)
Potential diff. across BC=potential diff. across DC
I1Q=I2S........(ii)
From equation (i) and (ii) I1PI1Q=I2RI2S⇒PQ=RS
(##ALNPHYC05S01061_A01##)
Step by step video, text & image solution for BALANCED WHEATSTONE BRIDGE by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
KCL & KVL|| Combination OF Resistance||Wheatstone Bridge
View SolutionFigure shows a balanced Wheatstone's bridge

(1) If P is slightly increased, the current in the galvanometer flow from A to C.
(2) If P is slightly increased, the current in the galvanometer flows C to A.
(3) If Q is slightly increased, the current in the galvanometer flows from C to A.
(4) If Q is slightly increased, the current in the galvanometer flows from A to C.View Solution
Knowledge Check
- Question 1 - Select One
Four resistors A,B,C and D from a wheatstone's bridge. The bridge is balanced when C=100Ω, if A and b are inter changed, the bridge balances for C=121Ω. The value of D is
A10ΩB100ΩC110ΩD120Ω - Question 2 - Select One
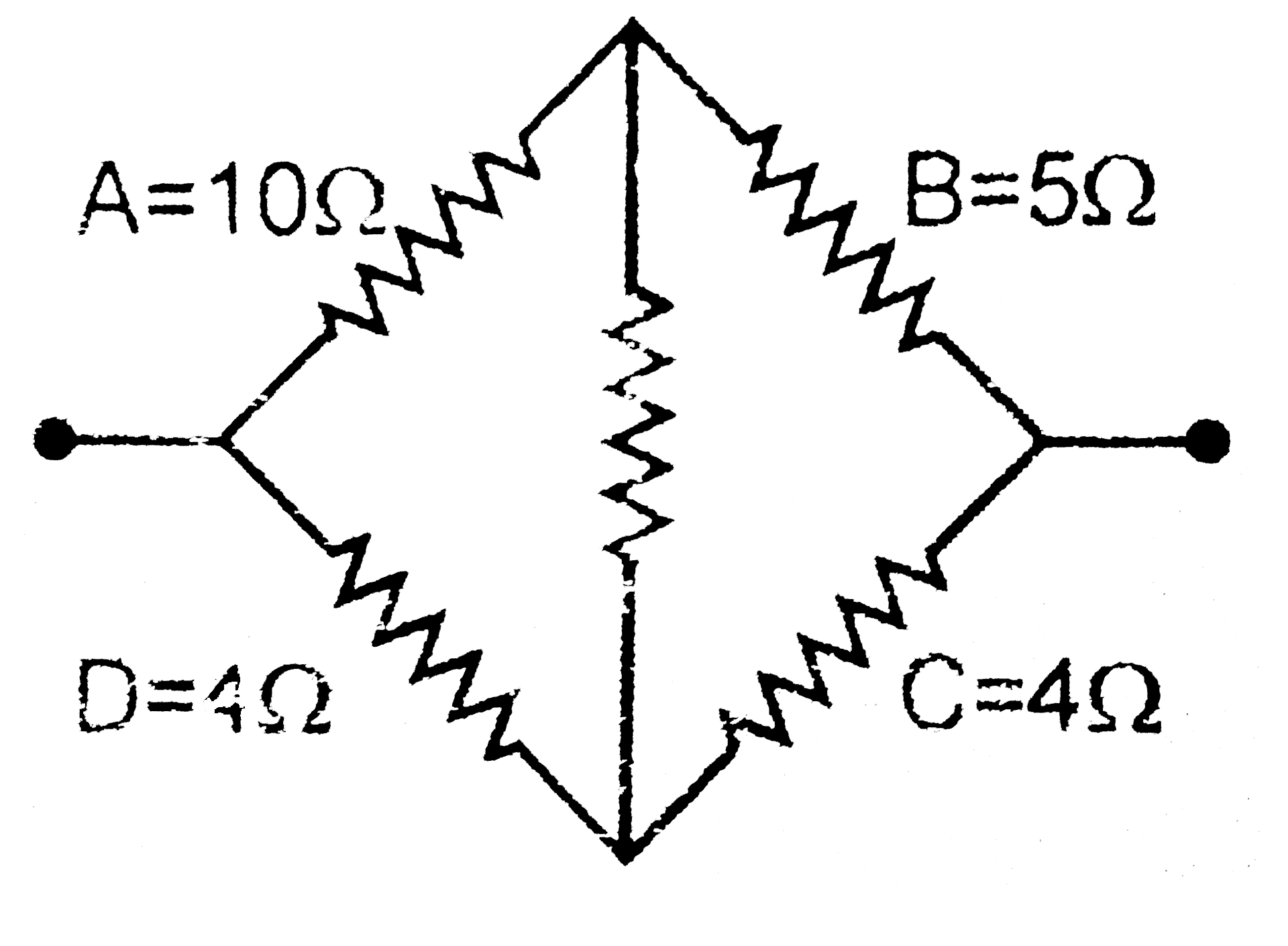
In a typical Wheatstone network, the resistances in cyclic order are A=10Ω,B=5Ω,C=4Ω D=4Ω for the bridge to be balanced
 A10Ω should be connected in parallel with AB10Ω should be connected in series with AC5Ω should be connected in series with BD5Ω should be connected in parallel with B
A10Ω should be connected in parallel with AB10Ω should be connected in series with AC5Ω should be connected in series with BD5Ω should be connected in parallel with B
Circuits Based On Wheatstone Bridge
View SolutionExtended Wheatstone Bridge
View SolutionStretching OF Wire and Change in Resistance||Wheatstone Bridge Example||Ladder Equivalent Resistance||Extended Wheatstone Bridge
View SolutionTricks for Combination Of Resistance,WheatStone Bridge
View SolutionIn the Wheatstone bridge network ABCD is balanced when P=500Ω,Q=250Ω and S=12Ω, what is the value of R ?
 View Solution
View SolutionIn a typical wheatstone network the resistance in cyclic order are A=10Ω,B=5O,ega,C=4Ω and D=4Ω for the bridge to be balanced.
 View Solution
View Solution

