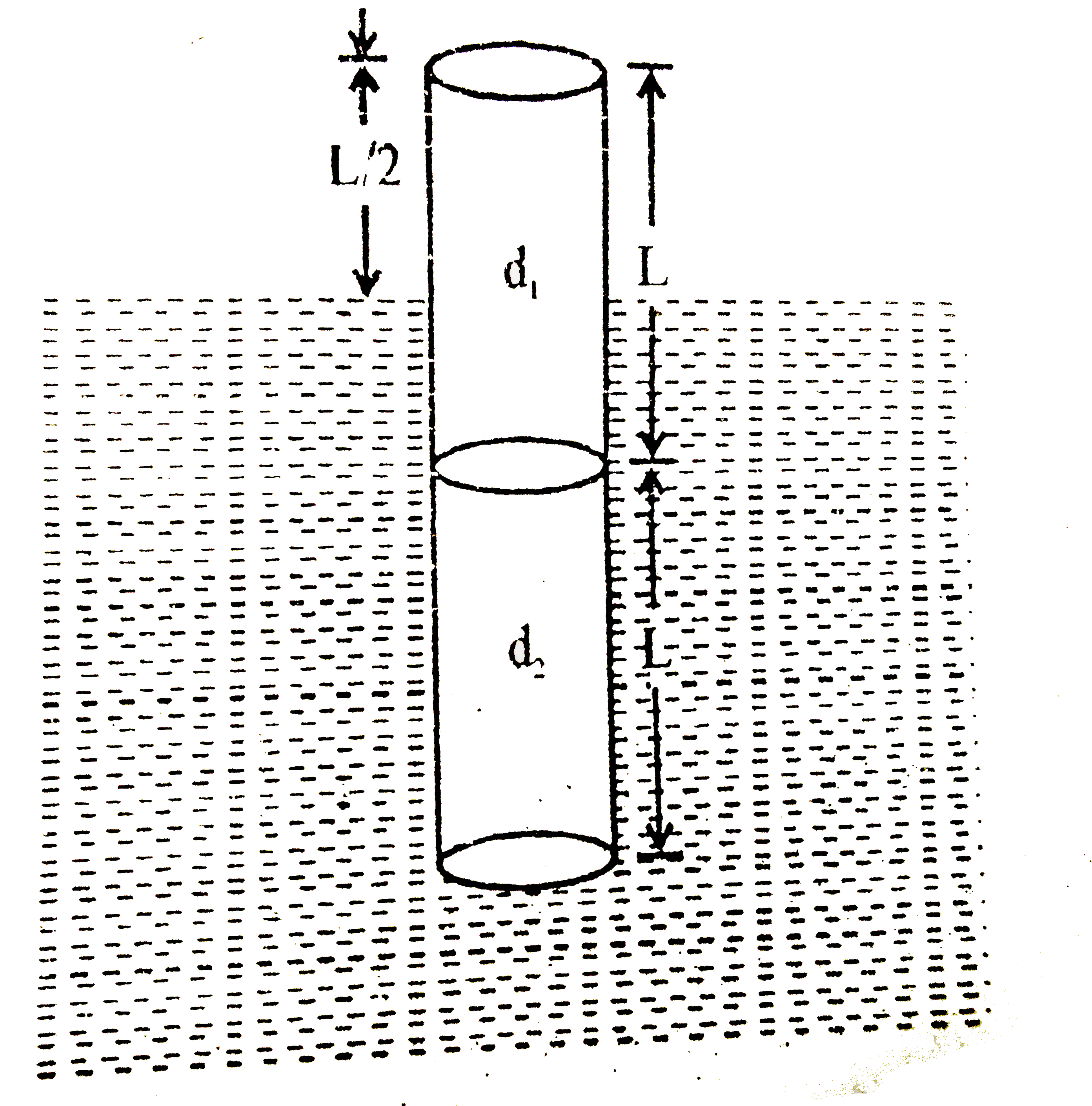
A cylindrical block of length 2l is made of two different materials. The upper half has density d1 and and lower half, which is heavier, has density d2 the block is floating in a liquid of unknown density d with l2 of its length outside the liquid.
(a) Find d
(b) Show that d>4d13
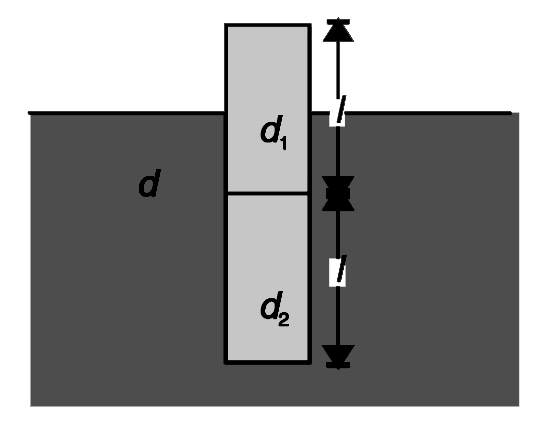
d1>34d
d2>d1
d4>d1
d<d1
The correct Answer is:A
m1g+m2g =Buyonany
ALd1g+ALd2g=34×LAdg
d1+d2=32d ⇒ d1>d2
2d1>d1+d2 ⇒ 2d1>32dimpliesd1>34d
Topper's Solved these Questions
Similar Questions
A block of material has a density ρ1 and floats three-fourth submerged in a liquid of unknown density. Show tht the density ρ2 of the unknown liquid is given by ρ2=43ρ1.
View Solutiontwo cylinders A and B are of the same dimensions but of different materials. A alone flots in a liquid with half of its length immersed. When B is placed over A , the combination just floats in the same liquid . Compare the densities of the two cylinders A and B.
View Solution
Knowledge Check
When a body of density d1 and volume V is floating in a liquid of density d2
Aits true weight is Vd2gBloss in its weight is Vd2gCits apparent weight is zeroDits density d1 is greater than that of liquid d2 .Two cylinders of same cross-section and length L but made of two different materials of densities d1 and d2 are cemented together to from a cylinder of length 2L. The combination floats in a liquid of density d d1<d2 then
Ad1<34dBd2>d1Cd4>d1Dd<d1An object weights m1 in a liquid of density d1 and that in liquid of density d2 is m2. The density of the object is
Am2d2−m1d1m2−m1Bm1d1−m2d2m2−m1Cm2d1−m1d2m1−m2Dm1d2−m2d1m1−m2
A composite rod whose upper half has a density ρ4 and lower half has a density of 3ρ2 is immersed vertically in a liquid of density ρ. To what length it shoul be immeresed so that centre of buoyancy coincides with centr of mass of the rod ? suppose the length is xl14 Find x .

View SolutionA drop of liquid of density ρ is floating half-immersed in a liquid of density d. If ρ is the surface tension the diameter of the drop of the liquid is
View SolutionA drop of liquid of density ρ is floating half-immersed in a liquid of density d. If ρ is the surface tension the diameter of the drop of the liquid is
View SolutionA drop of liquid of density p is floating half-immersed in a liquid of density d. If σ is the surface tension, then the diameter of the drop of the liquid is
View SolutionWeight of a body on the surfaces of two planets is the same. If their densities are d1andd2, then ratio of their radii is
View Solution
