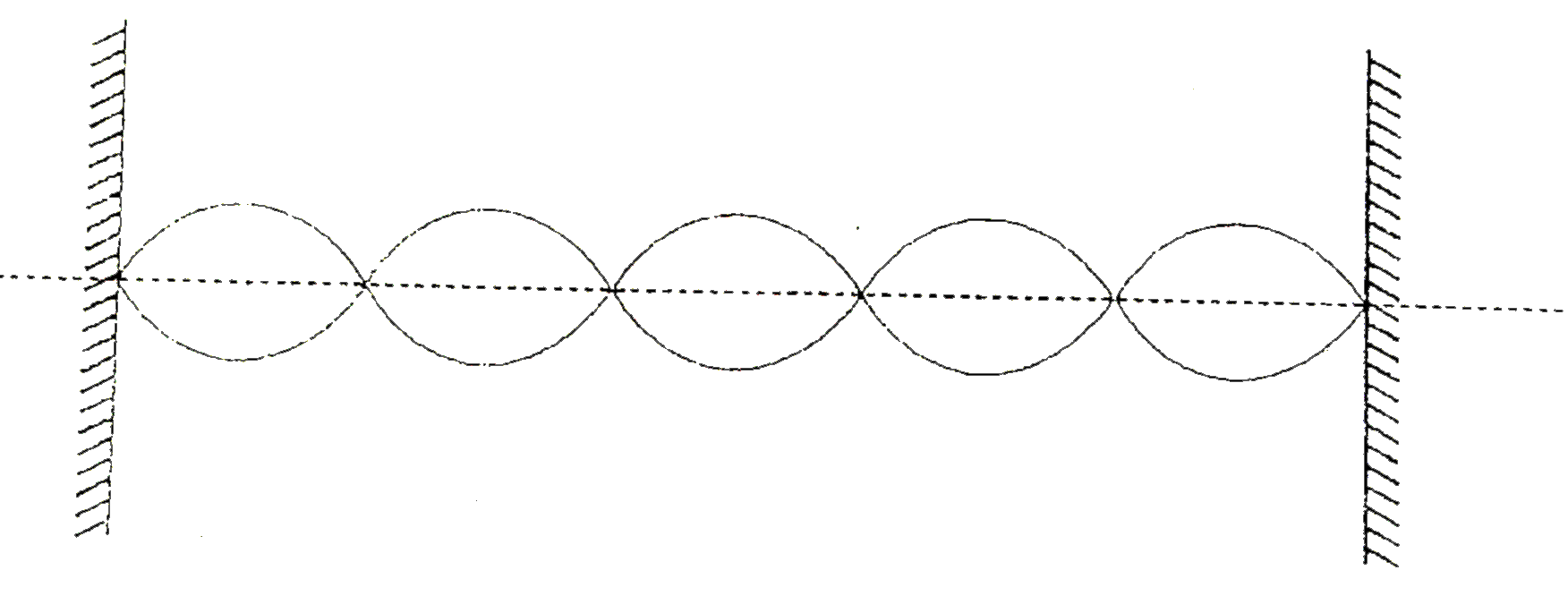
A horizontal stretched string, fixed at two ends, is vibrating in its fifth harmonic according to the equation, y(x,t)=(0.01m)sin[(62.8m−1)t]. Assuming π=3.14, the correct statement (s) is (are)
The number of nodes is 5.
The length of the string is 0.25m
The maximum displacement of the midpoint of the string its equilibrium position is 0.01m.
The fundamental frequency is 100Hz.
The correct Answer is:B,C
(A) There are 5 complete loops.
Total number of nodes =6
(B) ω=628sec−1
k=62.8m−1=2πλ⇒λ=110
vw=ωk=62862.8=10ms−1
L=5λ2=0.25
(C) 2A=0.01= maximum amplitude of antinode
(D) f=v2l=102×0.25=20Hz.
Topper's Solved these Questions
- TRAVELLING WAVESBOOK - RESONANCECHAPTER - TRAVELLING WAVESEXERCISE - Exercise- 3 PART II
7 Videos
- TRAVELLING WAVESBOOK - RESONANCECHAPTER - TRAVELLING WAVESEXERCISE - Exercise- 2 PART IV
9 Videos
- TEST SERIESBOOK - RESONANCECHAPTER - TEST SERIESEXERCISE - PHYSICS
127 Videos
- WAVE ON STRING BOOK - RESONANCECHAPTER - WAVE ON STRING EXERCISE - Exercise- 3 PART I
19 Videos
Similar Questions
The equation for the vibration of a string, fixed at both ends vibrating in its third harmonic, is given by y=(0.4cm)sin[(0.314cm−1)x]cos[f(600πs−1)t].(a) What is the frequency of vibration ? (b) What are the positions of the nodes ? (c) What is the length of the string ? (d) What is the wavelength and the speed of two travelling waves that can interfere to give this vibration ?
View SolutionA body oscillates with S.H.M. according to the equation
x(t)=(10m)cos[(2πt+π8)]
Calculate displacement and velocity at t = 1 s.
View Solution
Knowledge Check
A horizontal stretched string, fixed at two ends, is vibrating in its fifth harmonic according to the equation, t(x,t)=(0.01m)sin[(62.8m−1)t]. Assuming π=3.14, the correct statement (s) is (are)
A(a) The number of nodes is 5B(b) The length of the string is 0.25mC( c ) The maximum displacement of the midpoint of the string, from its equilibrium position is 0.01mD(d) The fundamental frequency is 100HzA horizontal stretched string, fixed at two ends, is vibrating in its fifth harmonic according to the equation, y(x,t)=(0.01m)sin[(628s−1)t]. Assuming π=3.14 , the correct statement(s) is (are) :
AThe number of nodes is 5.BThe length of the string is 0.25mCThe maximum displacement of the midpoint of the string its equilibrium position is 0.01m.DThe fundamental frequency is 100Hz.A horizontal stretched string, fixed at two ends, is vibrating in its fifth harmonic according to the equation, y(x,t)=(0.01m)sin[(62.8m−1x]cos[(628s−1)t]. Assuming p=3.14, the correct statement(s) is (are)
AThe number of node is 5BThe length of the string is 0.25 mCThe maximum displacement of the midpoint of the string. From its equilibrium position is 0.01 mDThe fundamental frequency is 100 Hz
the equation for the vibration of a string fixed both ends vibration in its third harmonic is given by
y = 2 cm sin [(0.6cm−1)×]cos[(500ps−1t]
View SolutionThe equation for the vibration of a string fixed at both ends vibrating in its third harmonic is given by y=2cmsin[(0.6cm−1)x]cos[(500πs−1)t]. The length of the string is
View SolutionA particle is subjected to two simple harmonic motion along x and y-directions according to equations x = 4sin100πt and y = 3sin100πt Choose the correct statement –
View SolutionA string is clamped at both the ends and it is vibrating in its 4th harmonic. The equation of the stationary wave is Y=0.3sin(0.157x)cos(200πt). The length of the string is: (All quantities are in SI units.)
View SolutionThe equation for the vibration of a string fixed at both ends vibrating in its second harmonic is given by y=2sin(0.3cm−1)xcos((500πs−1)t)cm. The length of the string is :
View Solution
