Doubtnut Promotions Sticky

The accompanying diagram represents a reversible cannot cycle for an ideal gas:
The accompanying diagram represents a reversible cannot cycle for an ideal gas: How many heat is rejected at the lower temperature, 200K, during the isothermal compression?
How many heat is rejected at the lower temperature, 200K, during the isothermal compression?

A

150 KJ

B

30KJ

C

120KJ

D

data are not sufficient to calculate exact value

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:B

−qc=qn(TcTh)=150×2001000
Heat rejected =30 KJ

|
Updated on:21/07/2023
Class 12CHEMISTRYTHERMODYNAMICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One

    The accompanying diagram represents a reversible cannot cycle for an ideal gas:
    ocr_image
    How many heat is rejected at the lower temperature, 200K, during the isothermal compression?

    A150 kJ
    B30 kJ
    C120 kJ
    Ddata are not sufficient to calculate exact value
  • Question 2 - Select One

    The accompanying diagram represents a reversible cannot cycle for an ideal gas:
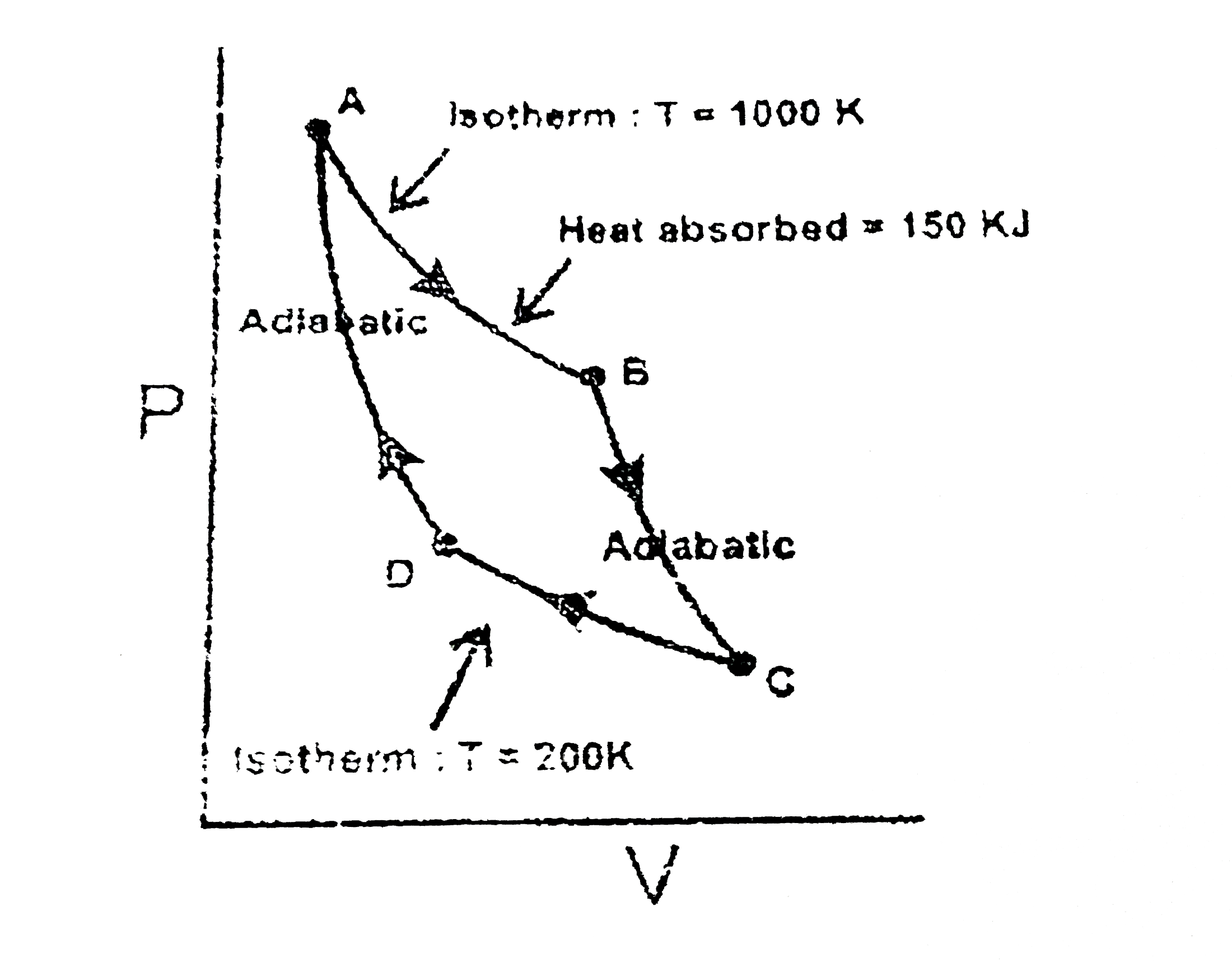
    ocr_image
    What is the entropy increase during isothermal at 1000K?

    A0.15KJ−1
    B150KJK−1
    C150JK−1
    D750JK−1
  • Question 3 - Select One

    The accompanying diagram represents a reversible cannot cycle for an ideal gas:
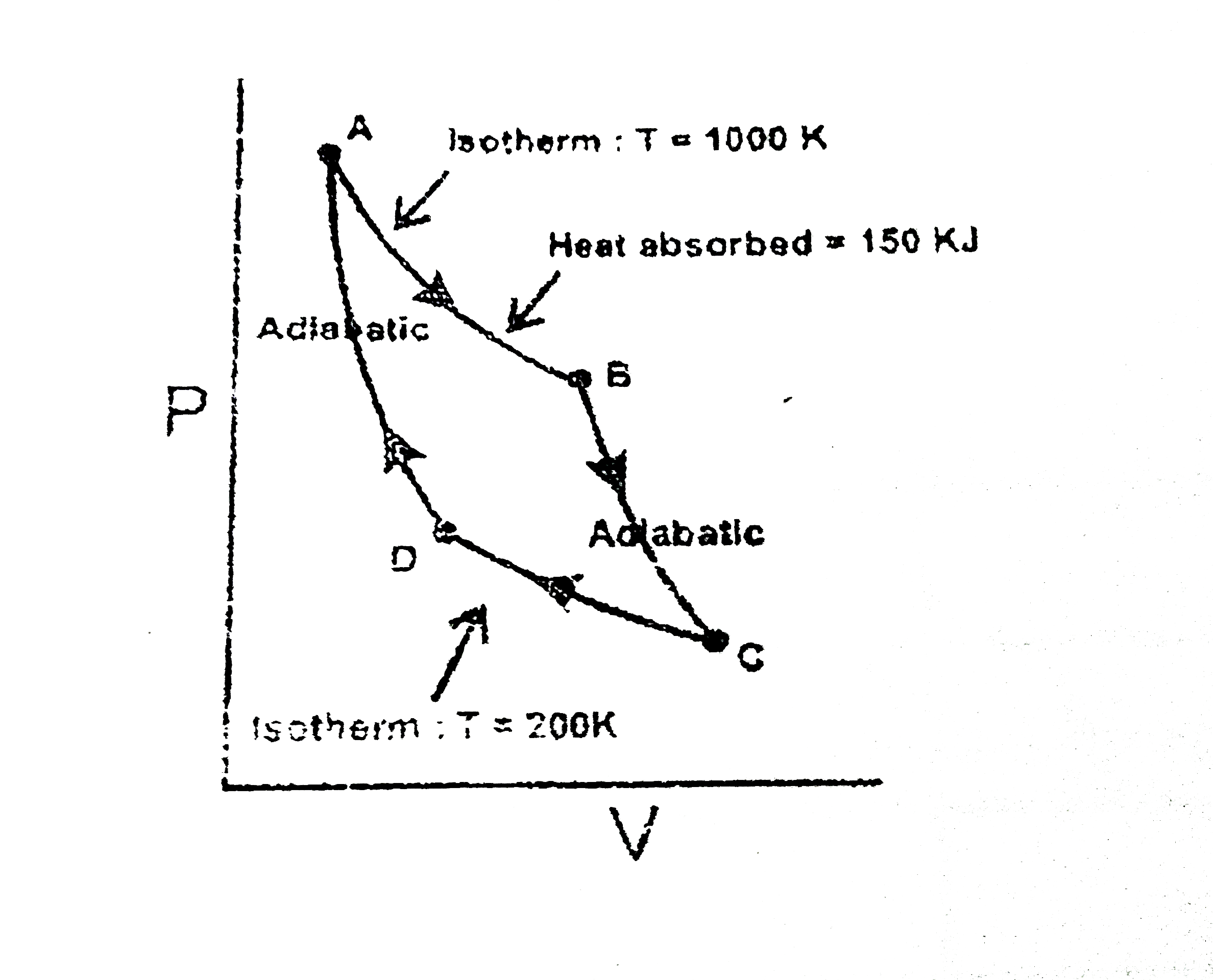
    ocr_image
    What is the Gibbs free energy change during the process A→B ?

    A150 KJ
    B−150KJ
    C30 KJ
    Ddata are not sufficient