An electrician has to repair an electric fault on a pole of height 5 m. She needs to reach a point 1.3m below the top of the pole to undertake the repair work. What should be the length of the ladder that she should use which, when inclined at
To solve the problem, we need to determine the length of the ladder required for the electrician to reach a point 1.3 meters below the top of a 5-meter pole while standing on the ladder inclined at an angle of 60 degrees to the horizontal.
1. Determine the Height to be Reached:
- The height of the pole (AD) is 5 meters.
- The electrician needs to reach a point 1.3 meters below the top of the pole.
- Therefore, the height (BD) to be reached is:
BD=5−1.3=3.7 meters
Hint: Always subtract the distance below the top from the total height to find the height to be reached.
2. Identify the Right Triangle:
- The ladder forms a right triangle (BCD) where:
- BD is the height (perpendicular) = 3.7 meters.
- BC is the length of the ladder (hypotenuse).
- CD is the base (horizontal distance from the pole).
3. Use the Sine Function:
- Since we know the angle of inclination (60 degrees), we can use the sine function:
sin(60∘)=BDBC
- Substituting the known values:
sin(60∘)=3.7BC
- The value of sin(60∘) is √32.
- Therefore, we have:
√32=3.7BC
- Rearranging gives:
BC=3.7×2√3=7.4√3
Hint: Use the sine function to relate the height and the hypotenuse in a right triangle.
4. Calculate the Length of the Ladder:
- To simplify BC:
√3≈1.73⇒BC≈7.41.73≈4.28 meters
Hint: Always approximate the square root values to simplify calculations.
5. Find the Base (Horizontal Distance):
- Now, we need to find the horizontal distance (CD) using the cosine function:
cos(60∘)=CDBC
- Since cos(60∘)=12, we have:
12=CDBC
- Rearranging gives:
CD=12×BC=12×4.28≈2.14 meters
Hint: Use the cosine function to find the base of the triangle, which represents the distance from the pole.
Final Answer:
- The length of the ladder required is approximately 4.28 meters.
- The distance from the foot of the pole to the foot of the ladder is approximately 2.14 meters.
- The height of the pole (AD) is 5 meters.
- The electrician needs to reach a point 1.3 meters below the top of the pole.
- Therefore, the height (BD) to be reached is:
BD=5−1.3=3.7 meters
- The ladder forms a right triangle (BCD) where:
- BD is the height (perpendicular) = 3.7 meters.
- BC is the length of the ladder (hypotenuse).
- CD is the base (horizontal distance from the pole).
- Since we know the angle of inclination (60 degrees), we can use the sine function:
sin(60∘)=BDBC
- Substituting the known values:
sin(60∘)=3.7BC
- The value of sin(60∘) is √32.
- Therefore, we have:
√32=3.7BC
- Rearranging gives:
BC=3.7×2√3=7.4√3
- To simplify BC:
√3≈1.73⇒BC≈7.41.73≈4.28 meters
- Now, we need to find the horizontal distance (CD) using the cosine function:
cos(60∘)=CDBC
- Since cos(60∘)=12, we have:
12=CDBC
- Rearranging gives:
CD=12×BC=12×4.28≈2.14 meters
- The length of the ladder required is approximately 4.28 meters.
- The distance from the foot of the pole to the foot of the ladder is approximately 2.14 meters.
Topper's Solved these Questions
Similar Questions
An electrician has to repair an electric fault on a pole of height 4m. He needs to reach a point 1.3m below the top of the pole to undertake the repair work. What should be the length of the ladder that he should use which when inclined at an angle of 60o to the horizontal would enable him to reach the required position?
View SolutionAn electrician has to repair an electric fault on a pole of height 4 metres.He needs to reach a point 1 m below the top of the pole to undertake the repair work.What should be the length of the ladder that he should use, which when inclined at an angle 60∘ to the horizontal would enable him to reach the required position? [use √3=1.73]
View Solution
Knowledge Check
- Question 1 - Select One
47 of a pole is in the mud. When 13 of it is pulled out, an 8 m long piece of the pole still remains in the mud. What is the total length of the pole?
A21 mB25 mC30 mD12 m - Question 2 - Select One
A ladder 9m long reaches a point 9 m below the top of a vertical flagstaff. From the foot of the ladder, the elevation of the ladder is 60∘. What is the height of the flag staff?
A10.5mB13.5mC13.5mD15m - Question 3 - Select One
A person of height 2m wants to get a fruit which is on a pole of height (103)m. If he stands at a distance (4√3)m from the foot of the pole, then the angle at which he should throw the stone, so that it hits the fruit is
A60∘B45∘C90∘D30∘
Anjali in an electrician and she has to repair an electric fault on a pole of height 5 m. She needs to reach to a point on the pole of 1.3 m below the top of the pole to undertake the repair work. What should be the length of the ladder that she should use which, when inclined at angle of 60° from the horizontal, would enable her to reach the required position? Further, how far from the foot of the pole should she place the foot of the ladder? What value is indicated from the question?
View SolutionA ladder 6m long reaches a point 6m below the top of a vertical flagstaff. From the foot of the ladder, the elevation of the top of the flagstaff is 75∘. What is the height of the flagstaff?
View SolutionA person of height 2m wants to get a fruit which is on a pole of height (103)m. If he stands at a distance (4√3)m from the foot of the pole, then the angle at which he should throw the stone, so that it hits the fruit is
View SolutionA stone is dropped from the top of tower. When it has fallen by 5m from the top, another stone is dropped from a point 25m below the top. If both stones reach the ground at the moment, then height of the tower from grounds is : (take g=10m/s2)
View SolutionRamesh places a mirror on level ground to determine the height of a pole (with traffic light fired on it). He stands at a certain distance so that he can see the top of the pole reflected from the mirror. Ramesh's eye level is 1.8 m above the ground. The distance of Ramesh and the pole from the mirror are 1.5 m and 2.5 m respectively.
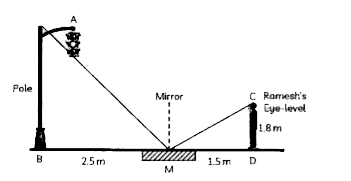
Which criterion of similarity is applicable to similar triangles?View Solution

