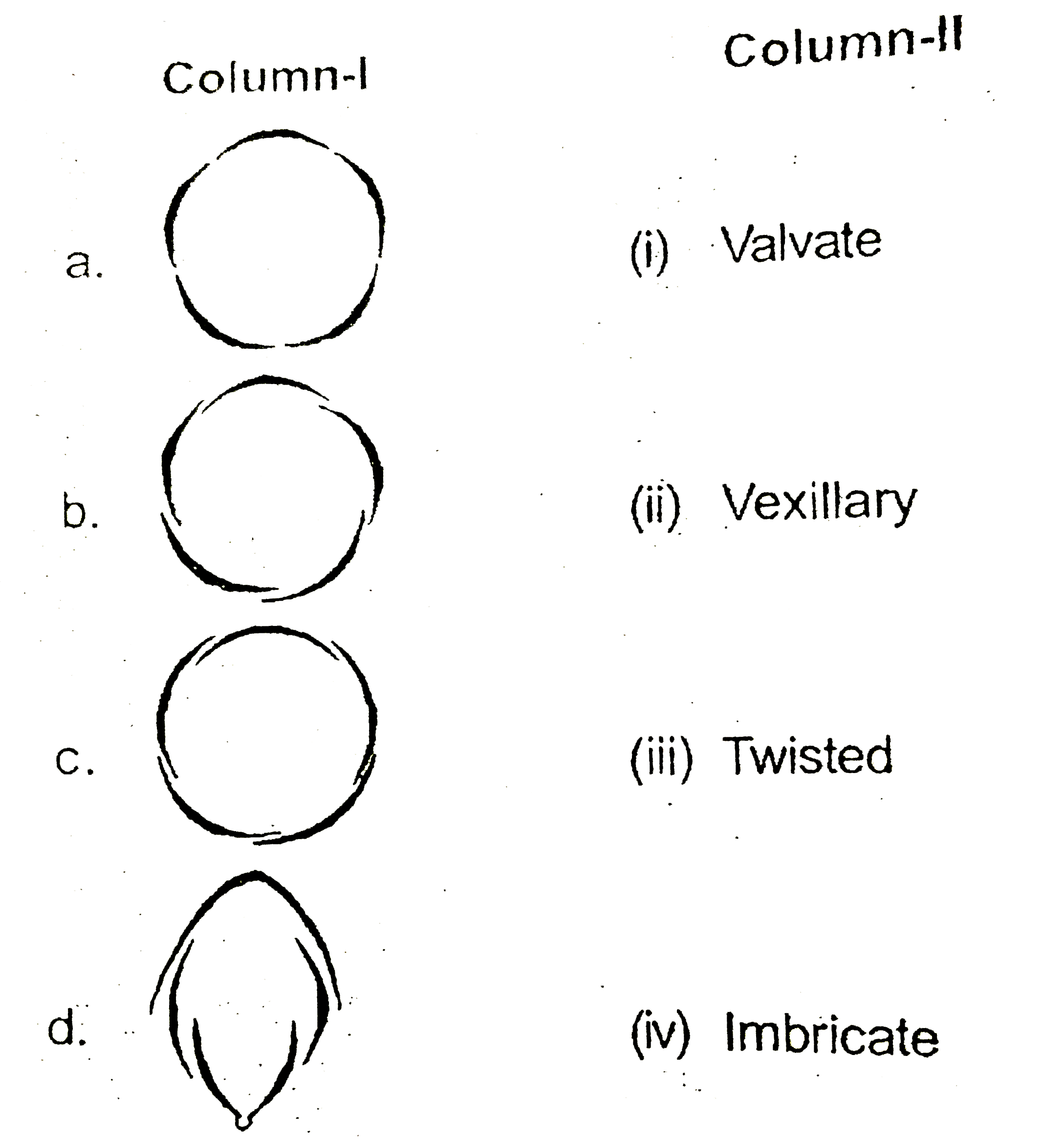
Match the type of interaction in column A with the distance dependence of their interaction energy in column B :
AB(I)ion-ion(a)1r(II)dipole-dipole(b)1r2(III)London dispersion(c)1r3(d)1r6
(I)-(a), (II)-(b), (III)-(d)
(I)-(a), (II)-(b), (III)-(c)
(I)-(a), (II)-(c), (III)-(d)
(I)-(a), (II)-(c), (III)-(b)
To solve the problem of matching the types of interactions in column A with their distance dependence of interaction energy in column B, we will analyze each interaction type and its corresponding distance dependence.
1. Identify Ion-Ion Interaction:
- Ion-ion interactions occur between charged ions (e.g., Na⁺ and Cl⁻ in NaCl).
- The interaction energy is inversely proportional to the distance between the ions.
- The relationship is given by:
E∝1r
- Thus, we match Ion-Ion (I) with (a) 1r.
2. Identify Dipole-Dipole Interaction:
- Dipole-dipole interactions occur between polar molecules that have permanent dipoles.
- The interaction energy decreases with the cube of the distance between the dipoles.
- The relationship is given by:
E∝1r3
- Thus, we match Dipole-Dipole (II) with (c) 1r3.
3. Identify London Dispersion Forces:
- London dispersion forces are weak interactions that occur due to temporary dipoles induced in atoms or molecules.
- The interaction energy decreases with the sixth power of the distance.
- The relationship is given by:
E∝1r6
- Thus, we match London Dispersion (III) with (d) 1r6.
Final Matching:
- I (Ion-Ion) → (a) 1r
- II (Dipole-Dipole) → (c) 1r3
- III (London Dispersion) → (d) 1r6
Summary of Matches:
- I → a
- II → c
- III → d
- Ion-ion interactions occur between charged ions (e.g., Na⁺ and Cl⁻ in NaCl).
- The interaction energy is inversely proportional to the distance between the ions.
- The relationship is given by:
E∝1r
- Thus, we match Ion-Ion (I) with (a) 1r.
- Dipole-dipole interactions occur between polar molecules that have permanent dipoles.
- The interaction energy decreases with the cube of the distance between the dipoles.
- The relationship is given by:
E∝1r3
- Thus, we match Dipole-Dipole (II) with (c) 1r3.
- London dispersion forces are weak interactions that occur due to temporary dipoles induced in atoms or molecules.
- The interaction energy decreases with the sixth power of the distance.
- The relationship is given by:
E∝1r6
- Thus, we match London Dispersion (III) with (d) 1r6.
- I (Ion-Ion) → (a) 1r
- II (Dipole-Dipole) → (c) 1r3
- III (London Dispersion) → (d) 1r6
- I → a
- II → c
- III → d
Topper's Solved these Questions
Similar Questions
Knowledge Check
Match the columns
Column-AColumn-B(a)Ribs(i)26(b)Vertebrae(ii)14(c)Phalanges(iii)1(d)Sternum(iv)14Aiv,I,iii,iiBiv,iii,ii,iCiv,I,ii,iiiDiv,iii,I,iiiMatch the following columns. (for a satellite in circular orbit)
Column-IColumn-II(A)Kinetic energy(p)−GMm2r(B)Potential energy(q)√GMr(C)Total energy(r)−GMmr(D)Orbital speed(s)GMm2rA(A→s,B→r,C→p,D→q)B(A→c,B→s,C→p,D→q)C(A→s,B→r,C→q,D→p)D(A→q,B→r,C→p,D→s)The correct match of the following is :
Column - IColumn - II(i)nCr(a)n!(ii)nC0(b)1(iii)nPr(c)r!.nCr(iv)nPn(d)nCn−rA(i)-(d), (ii)-(b), (iii)-(c ), (iv)-(a)B(i)-(a), (ii)-(b), (iii)-(c ), (iv)-(d)C(i)-(b), (ii)-(c ), (iii)-(d), (iv)-(a)D(i)-(d), (ii)-(b), (iii)-(a), (iv)-(c )
Match the statement (A,B,C,D) in column I with the statement (p,q,r,s) in column II. The answers are to be properly bobbled.
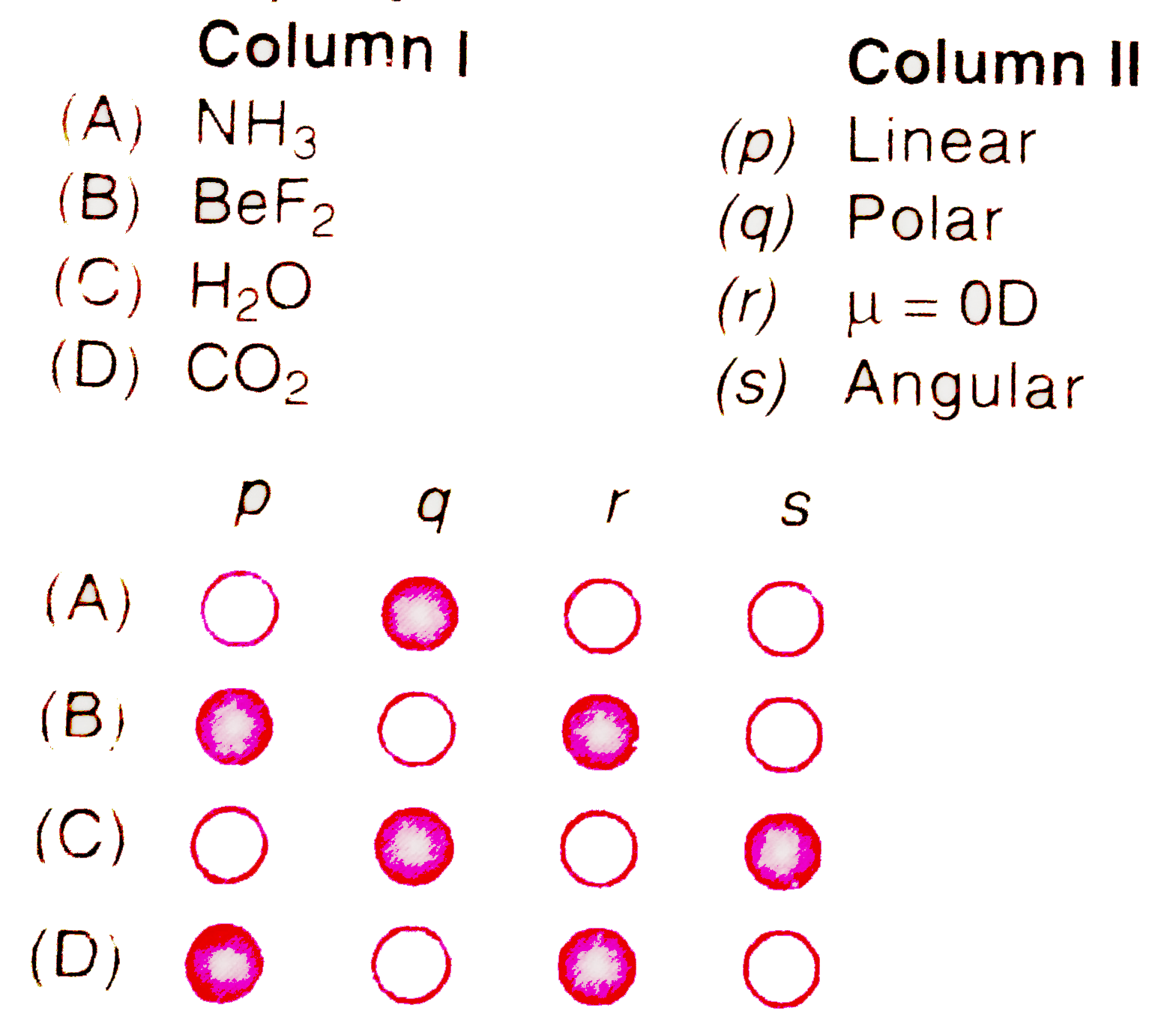
View SolutionMatch the species in Column I with the type of hybrid orbitals in Column II.
Column IColumn II(i)BF3(a)sp3d(ii)H2O(b)sp2(iii)PCl5(c)sp3d2(iv)SF6(d)sp3
View SolutionColumn IColumn II(a)Work(p)WF(b)Kinetic energy(q)mgh(c)Potential energy(r)12mv2(d)Displacement(s)F×S
View SolutionName the types of interaction or intermolecular forces of which potential energy-distance function are given below
(a) E∝1r (b) E∝1r2 (c ) E∝1r3
(d) E∝1r4 (e) E∝1r6 .
View SolutionA semicircular ring has mass m and radius R as shown in figure. Let I1,I2,I3andI4 be the moments of inertia of the four axes as shown. Axis 1 passes through centre and is perpendicular to plane of ring. Then, match the following columns.
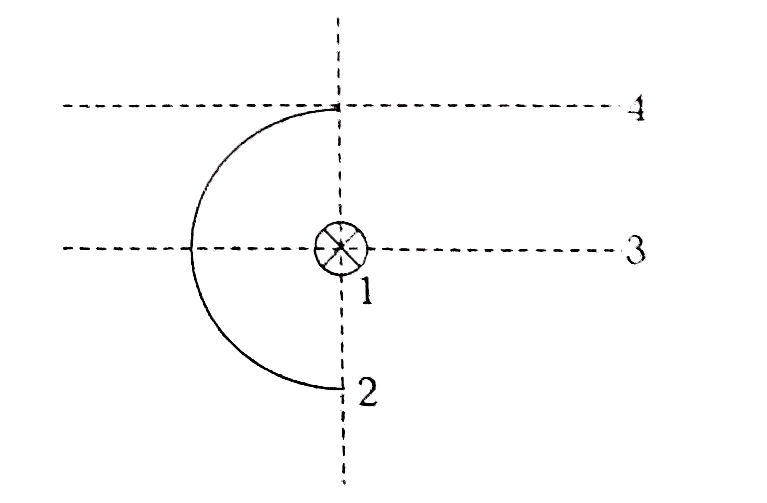
Column-IColumn-II(A)I1(p)mR22(B)I2(q)(3/2)mR2(C)I3(r)mR2(D)I4(s)Data is insufficient
View Solution