A conductor is at T kelvin. Find the thermal speed of free electrons of the conductor .
To find the thermal speed of free electrons in a conductor at temperature T Kelvin, we can follow these steps:
1. Understand the Concept of Thermal Speed:
The thermal speed (or thermal velocity) of free electrons in a conductor is related to the temperature of the conductor. It can be derived from the kinetic theory of gases, which applies to free electrons in a conductor.
2. Formula for Thermal Speed:
The thermal speed v of free electrons can be expressed as:
v=√3kTm
where:
- k is the Boltzmann constant (approximately 1.38×10−23J/K),
- T is the absolute temperature in Kelvin,
- m is the mass of an electron (approximately 9.11×10−31kg).
3. Kinetic Energy of Electrons:
The kinetic energy KE of an electron can be expressed as:
KE=12mv2
By substituting the expression for v into the kinetic energy formula, we can find the average kinetic energy of the electrons.
4. Substituting for v:
Substitute v=√3kTm into the kinetic energy formula:
KE=12m(√3kTm)2
5. Simplifying the Kinetic Energy Expression:
KE=12m⋅3kTm=32kT
This shows that the average kinetic energy of the free electrons in the conductor at temperature T is 32kT.
6. Conclusion:
The thermal speed of free electrons in a conductor at temperature T Kelvin is given by:
v=√3kTm
Final Answer:
The thermal speed of free electrons in a conductor at T Kelvin is:
v=√3kTm
The thermal speed (or thermal velocity) of free electrons in a conductor is related to the temperature of the conductor. It can be derived from the kinetic theory of gases, which applies to free electrons in a conductor.
The thermal speed v of free electrons can be expressed as:
v=√3kTm
where:
- k is the Boltzmann constant (approximately 1.38×10−23J/K),
- T is the absolute temperature in Kelvin,
- m is the mass of an electron (approximately 9.11×10−31kg).
The kinetic energy KE of an electron can be expressed as:
KE=12mv2
By substituting the expression for v into the kinetic energy formula, we can find the average kinetic energy of the electrons.
Substitute v=√3kTm into the kinetic energy formula:
KE=12m(√3kTm)2
KE=12m⋅3kTm=32kT
This shows that the average kinetic energy of the free electrons in the conductor at temperature T is 32kT.
The thermal speed of free electrons in a conductor at temperature T Kelvin is given by:
v=√3kTm
The thermal speed of free electrons in a conductor at T Kelvin is:
v=√3kTm
Similar Questions
Knowledge Check
Mobility of free electrons in a conductor is
ADirectly proportional to electron densityBDirectly proportional to relaxation timeCInversely proportional to electron densityDInversely proportional to relaxation timeif E denotes electric field in a uniform conductor and vd the corresponding drift velocity of free electrons in the conductor, then which of the following graph is correct
A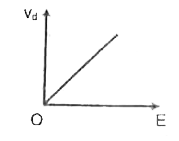 B
B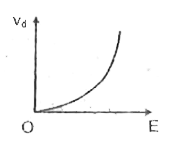 C
C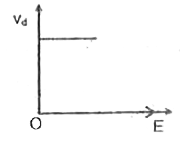 D
D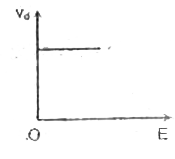
A straight conductor carries a current. Assume that all free electrons in the conductor move with the same drift velocity v. A and B are two observers on a striaght line XY parallel to the conductor. A is stationary B moves along XY with a velocity v in the direction of the free electrons.
AA and B observe the same magnetic field.BA observes a magnetic field B does not.CA and B observe magnetic fiels of the same magnitude but opposite directionsDA and B do not observe any electric field.
What do you mean by thermal motion of free electrons in conductors ?
View SolutionWhat are the possible paths of free electrons inside the conductor ?
View SolutionWhat are the possible paths of free electrons inside the conductor ?
View SolutionWhen in electric field is applied to a metallic conductor at room temperature the thermal speed of electrons is……….and drift velocity of electrons is …….
View SolutionA current of 10 A is maintained in a conductor of cross-section 1cm2 . If the free electron density in the conductor is 9×1028m−3 , then drift velocity of free electrons is
View Solution
