Tow cells of e.m.f E1 and E2 are joined in series and the balancing length of the potentiometer wire is 625 cm. If the terminals of E1 are reversed , the balancing length obtained is 125 cm. Given E2>E1,the ratio E1:E2 willbe
2:3
5:1
3:2
1:5
The correct Answer is:A
To solve the problem, we need to find the ratio E1:E2 given two scenarios involving a potentiometer. Let's break down the solution step by step.
Step 1: Understanding the potentiometer setup
When two cells E1 and E2 are connected in series, the total EMF is given by:
Etotal=E1+E2
The balancing length of the potentiometer wire is proportional to the total EMF.
Step 2: Setting up the equations
From the problem, we know:
1. When both cells are connected with the same polarity, the balancing length L1=625cm.
2. When the terminals of E1 are reversed, the balancing length L2=125cm.
Using the relationship of balancing lengths to EMF, we can write:
L1L2=E1+E2E2−E1
Substituting the values of L1 and L2:
625125=E1+E2E2−E1
This simplifies to:
5=E1+E2E2−E1
Step 3: Cross-multiplying to form an equation
Cross-multiplying gives:
5(E2−E1)=E1+E2
Expanding this, we have:
5E2−5E1=E1+E2
Rearranging terms leads to:
5E2−E2=5E1+E1
This simplifies to:
4E2=6E1
Step 4: Finding the ratio
Dividing both sides by 2E1 gives:
E1E2=46=23
Thus, the ratio E1:E2 is:
E1:E2=2:3
Final Answer
The ratio E1:E2 is 2:3.
---
When two cells E1 and E2 are connected in series, the total EMF is given by:
Etotal=E1+E2
The balancing length of the potentiometer wire is proportional to the total EMF.
From the problem, we know:
1. When both cells are connected with the same polarity, the balancing length L1=625cm.
2. When the terminals of E1 are reversed, the balancing length L2=125cm.
L1L2=E1+E2E2−E1
Substituting the values of L1 and L2:
625125=E1+E2E2−E1
This simplifies to:
5=E1+E2E2−E1
Cross-multiplying gives:
5(E2−E1)=E1+E2
Expanding this, we have:
5E2−5E1=E1+E2
Rearranging terms leads to:
5E2−E2=5E1+E1
This simplifies to:
4E2=6E1
Dividing both sides by 2E1 gives:
E1E2=46=23
Thus, the ratio E1:E2 is:
E1:E2=2:3
The ratio E1:E2 is 2:3.
Similar Questions
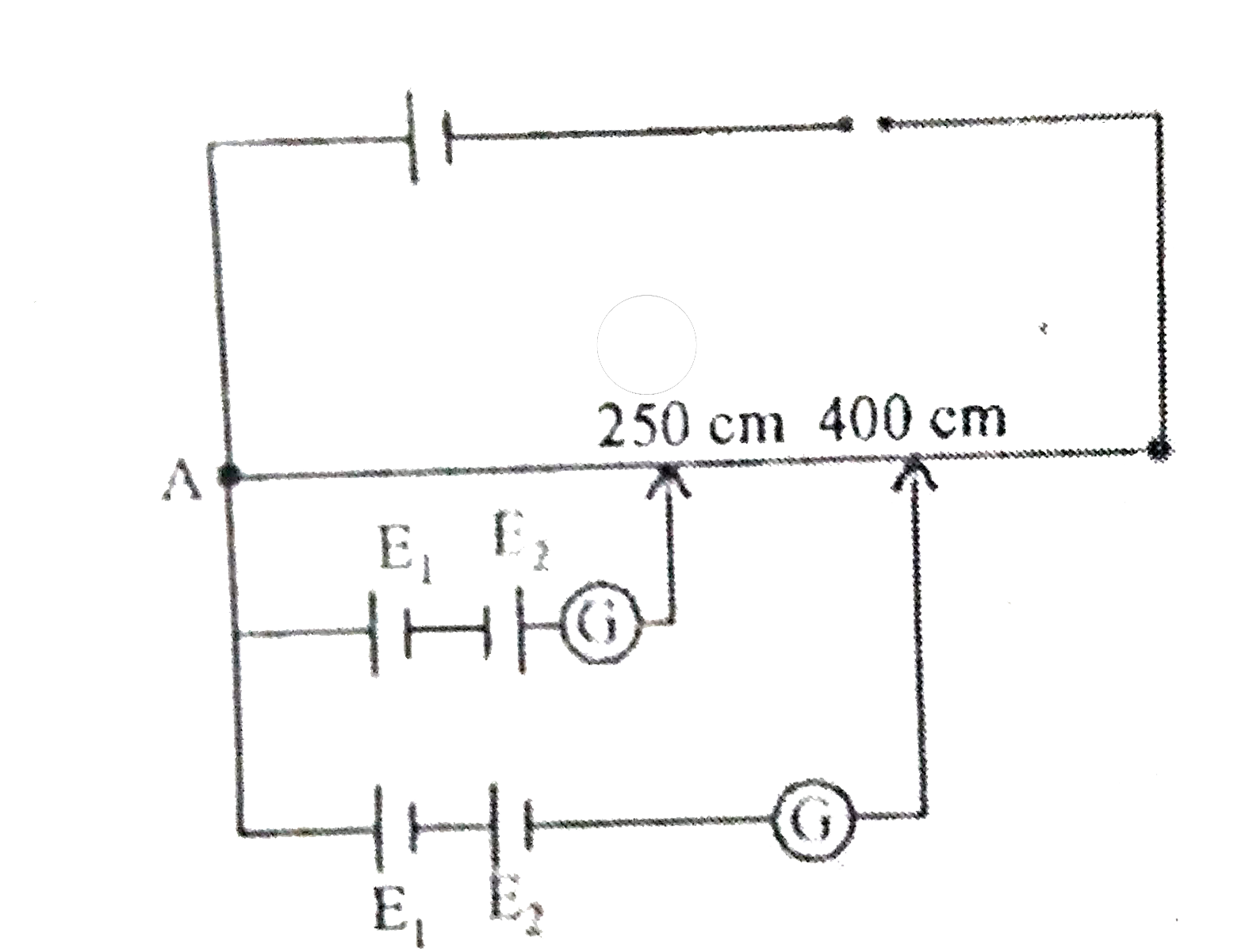
Two primary cells of emfs E1 and E2 are connected to the potentiometer wire AB as shown in the figure . If the balancing lengths for the two combinations of the cells are 250 cm and 400 cm , find the ratio of E1 and E2 .
View SolutionTwo cells of emf E1 and E2(E1>E2) are connected as shown in the figure below. When a potentiometer is used to measure potential difference between the points A and B, the balancing length of the potentiometer wire is 300 cm. But the same potentiometer for the potential difference between points A and C, gives the balancing length 100 cm. Find E1E2.

View Solution
Knowledge Check
Two cells of emf E1 and E2(E1>E2) are connected in series to potentiometer for balancing length 625 cm . When polarity of E2 is reversed then balancing length becomes 125 cm. Then the ratio E1E2 is
A3:2B3:1C5:1D2:1In a potentiometer experiment two cells of e.m.f. E and E are used in series and in conjunction and the balancing length is found to be 58 cm of the wire. If the olarity of E is reversed, then the balancing length becomes 29 cm . The ratio E1E2 of the e.m.f. of the two cells is
A1:1B2:1C3:1D4:1Two cells of emfs E1 and (E2(E1>E2) are connected as shows in Fig. 6.45.
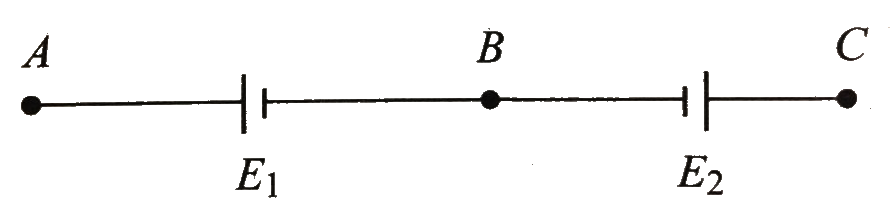
When a potentiometer is connected between A and B, the balancing length of the potentiometer wire is 300cm. On connecting the same potentiometer between A and C, the balancing length is 100cm. The ratio E1/E2 isA3:1B1:3C2:3D3:2
Two cells of E.M.F. E1 and E2(E2>E1) are connected in series in the secondary circuit of a potentiometer experiment for determination of E.M.F. The balancing length is found to be 825 cm. Now when the terminals to cell E1 are reversed, then the balancing length is found to be 225 cm. The ratio of E1 and E2 is
View SolutionTwo cells of e.m.f.'s E1andE2 where E1>E2, are connected in series so as to assist each other, the balancing length is 2.7m . When the cells are connected in series so as to oppose each other, the balancing length is found to be 0.3m . What is the ratio of their e.m.f.'s ?
View SolutionIn the above question, if the balancing length for a cell of emf E is 60cm, the value of E will be
View SolutionTwo cells when connected in series are balanced on 6 m on a potentiometer. If the polarity one of these cell is reversed, they balance on 2m. The ratio of e.m.f of the two cells.
View SolutionA cell of emf (E) and internal resistance (r) is balanced across (l) length of potentiometer wire. If another cell of emf 2E and internal resistance (2r) is connected in parallel to the first cell , then the balancing length will be
View Solution
