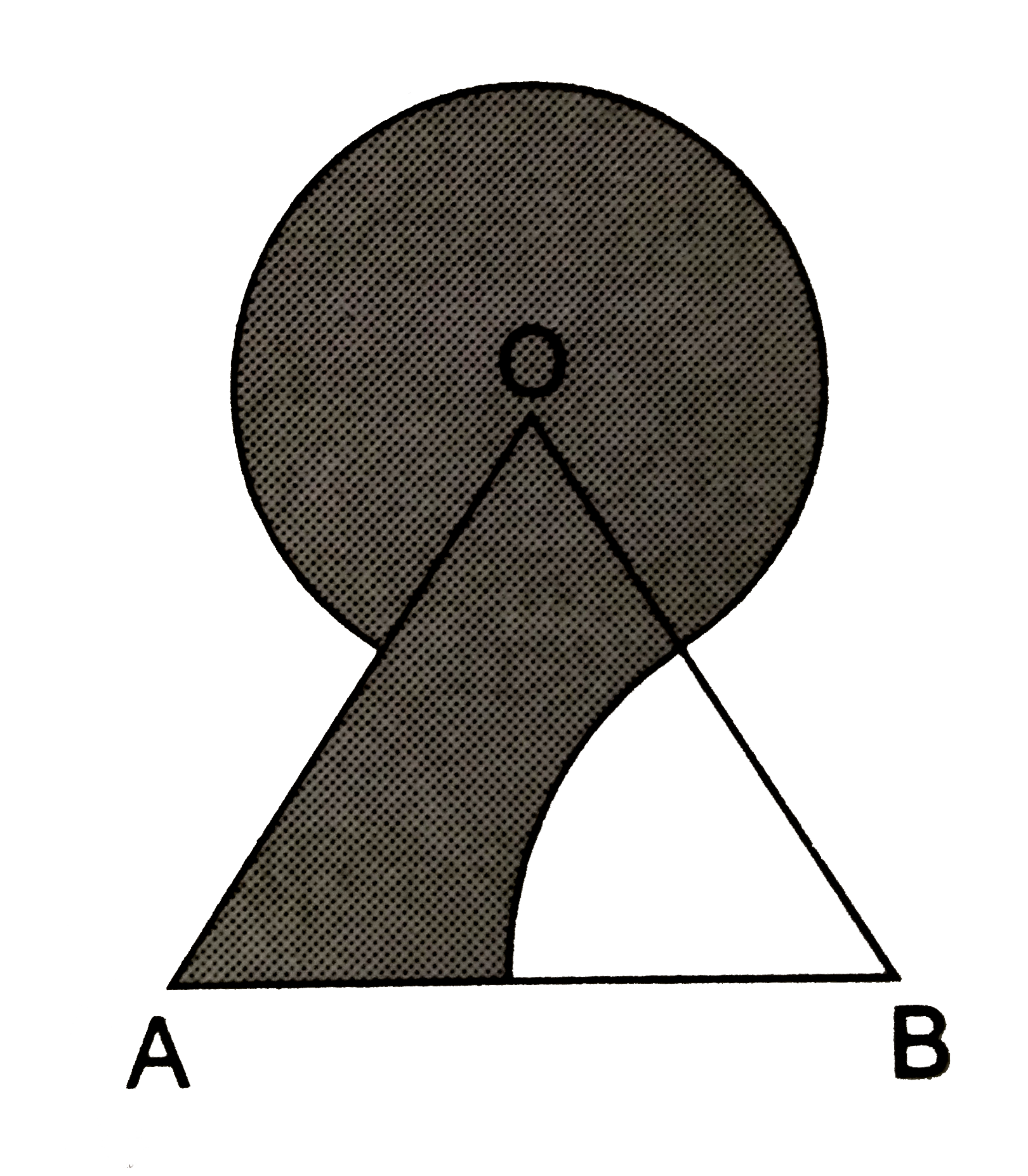
Find the area of the shaded region in Fig. 12.22, where a circular arc of radius 6 cm has been drawn with vertex O of an equilateral triangle OAB of side 12 cm as centre .
area(shaded)=area(OAB)+area(major sector)
=√34⋅r2+θ360πr2
=√34122+300360⋅227⋅62
=36√3+6607cm2
Similar Questions
Find the area of the shaded region in Fig. 12.22, where a circular arc of radius 6 cm has been drawn with vertex O of an equilateral triangle OAB of side 12 cm as centre .
View SolutionFind the areas of the shaded region in Fig. 15.52, where a circular arc of radius 6 cm has been drawn with vertex O of an equilateral triangle OAB of side 12 cm as centre. (FIGURE)
View SolutionFind the area of the shaded region in the given figure, where a circular arc of radius 6 cm has been drawn with vertex O of an equilateral triangle OAB of side 12 cm as centre.
[ Take √3=1.73 and π=3.14]
 View Solution
View SolutionFind the area of the shaded region in the given figure, where a circular of arc of radius 6 cm has been drawn with vertex of an equilateral triangle of side 12 cm as centre and a sector of circle of radius 6 cm with centre B is made. [ Use √3=1.73 and π=3.14]
 View Solution
View SolutionFind the area of a shaded region in the figure, where a circular arc of radius 7cm has been drawn with vertex A of an equilateral triangle ABC of side 14cm as centre.
View SolutionFind the area of the shaded region given in Fig. 12.72.
View SolutionFind the area of an equilateral triangle of side 12 cm.
View SolutionFind the height of an equilateral triangle of side 12 cm
View SolutionFind the area of an equilateral triangle of side 6 cm.
View SolutionFind the area of an equilateral triangle of side 6 cm.
View Solution

