Given the parabola C : y = x^(2). If the circle at y axis with radius

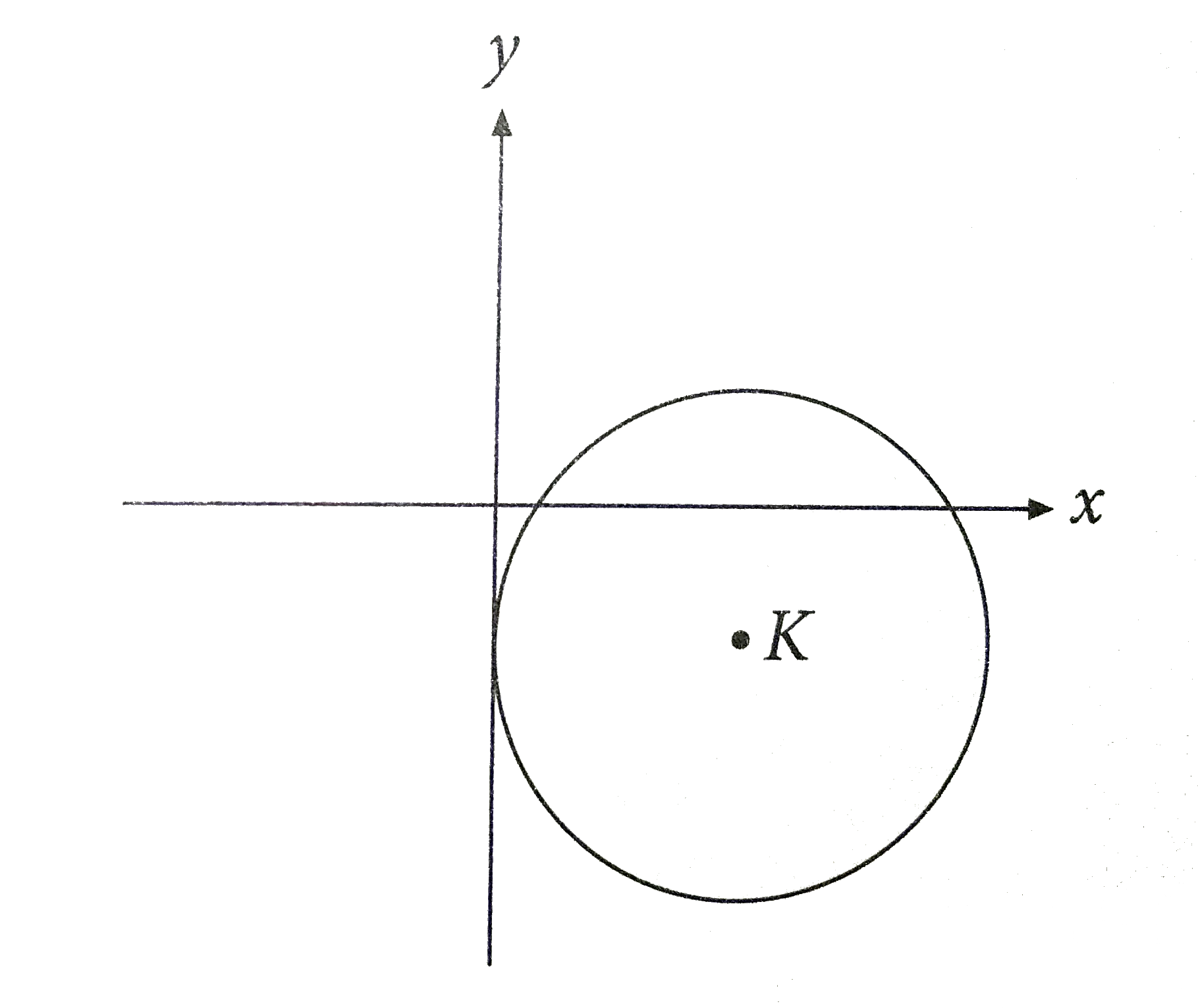
Point K is the center of the circle above, and the coordinates of Point K are (2, -1). What is the area of the circle?
A $π$
B $2 π$
C $4 π$
D $8 π$
The area (in sq. units) of the smaller of the two circles that touch the parabola, $y^{2} = 4 x$ at the point (1, 2) and the x-axis is:
A $4 π (2 - \sqrt{2})$
B $8 π (2 - \sqrt{2})$
C $8 π (3 - 2 \sqrt{2})$
D $4 π (3 + \sqrt{2})$

Given the parabola $C : y = x^{2}$ . If the circle at y axis with radius 1 touches parabola C at two distinct points, then find the coordinate of the center of the circle K and the area of the figure surrounded by C and K.