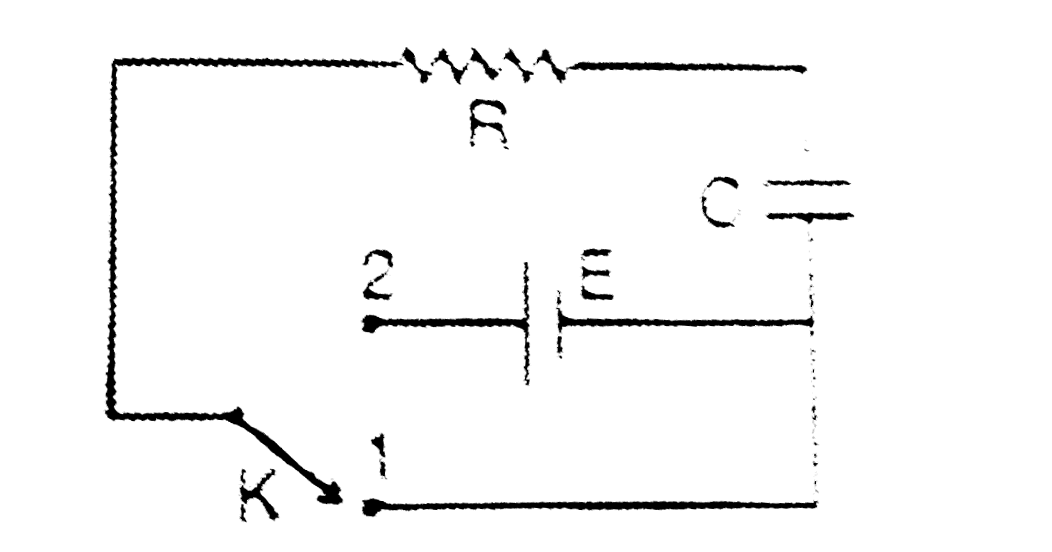
In the shown circuit involving a resistor of resistance RΩ, capacitor of capacitance C farad and an ideal cell of emf E volts, the capacitor is initially uncharged and the key is in position 1. At t = 0 second the key is pushed to position 2 for t0=RC seconds and then key is pushed back to position 1 for t0=RCseconds. This process is repeated again and again. Assume the time taken to push key from position 1 to 2 and vice verse to be negligible.
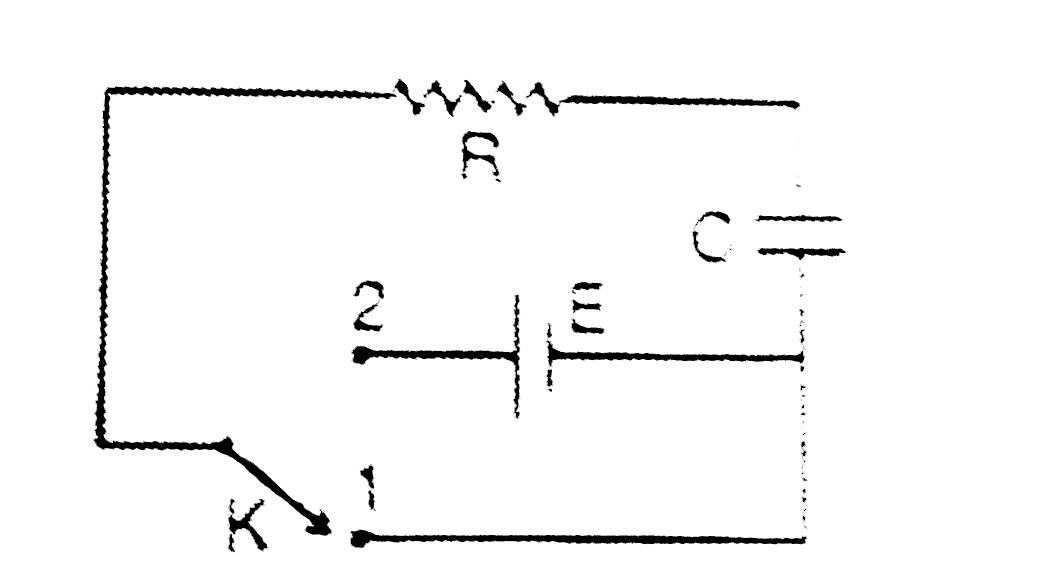
The current through the resistance at t = 1.5 RC seconds is
Ee2R(1−1e)
EeR(1−1e)
ER(1−1e)
E√eR(1−1e)
The correct Answer is:D
Topper's Solved these Questions
- CAPACITANCEBook:RESONANCEChapter:CAPACITANCEExercise:Exercise - 3
Explore 26 Videos
- CAPACITANCEBook:RESONANCEChapter:CAPACITANCEExercise:High Level Problems
Explore 16 Videos
- CAPACITANCEBook:RESONANCEChapter:CAPACITANCEExercise:Exercise - 1
Explore 79 Videos
- ATOMIC PHYSICSBook:RESONANCEChapter:ATOMIC PHYSICSExercise:Advanved level problems
Explore 17 Videos
- COMMUNICATION SYSTEMSBook:RESONANCEChapter:COMMUNICATION SYSTEMSExercise:Exercise 3
Explore 13 Videos
Similar Questions
In the shown circuit involving a resistance Rω, capacitor of capacitance C farad and an ideal cell of emf E volts, the capacitor is initially uncharged and the key is in position 1. At t = 0, second the key is pushed to position 2 for t0=RC second and then key is pushed back to position 1 for t0=RC seconds. This process is repeated again and again. Assume the time taken to push key from position 1 to 2 and vice versa to be negligible.
The current through the resistance at seconds is:
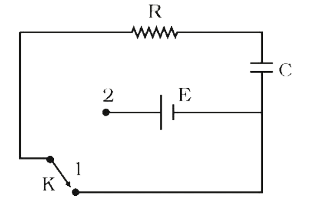
View SolutionIn the shown circuit involving a resistance Rω, capacitor of capacitance C farad and an ideal cell of emf E volts, the capacitor is initially uncharged and the key is in position 1. At t = 0, second the key is pushed to position 2 for t0=RC second and then key is pushed back to position 1 for t0=RC seconds. This process is repeated again and again. Assume the time taken to push key from position 1 to 2 and vice versa to be negligible.
Then the variation of charge on capacitor with time is best represented by
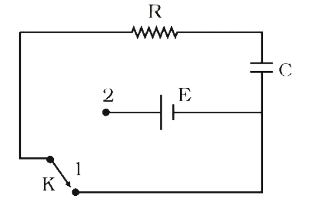
View Solution
Knowledge Check
In the shown circuit involving a resistor of resistance RΩ, capacitor of capacitance C farad and an ideal cell of emf E volts, the capacitor is initially uncharged and the key is in position 1. At t = 0 second the key is pushed to position 2 for t0=RC seconds and then key is pushed back to position 1 for t0=RCseconds. This process is repeated again and again. Assume the time taken to push key from position 1 to 2 and vice verse to be negligible.
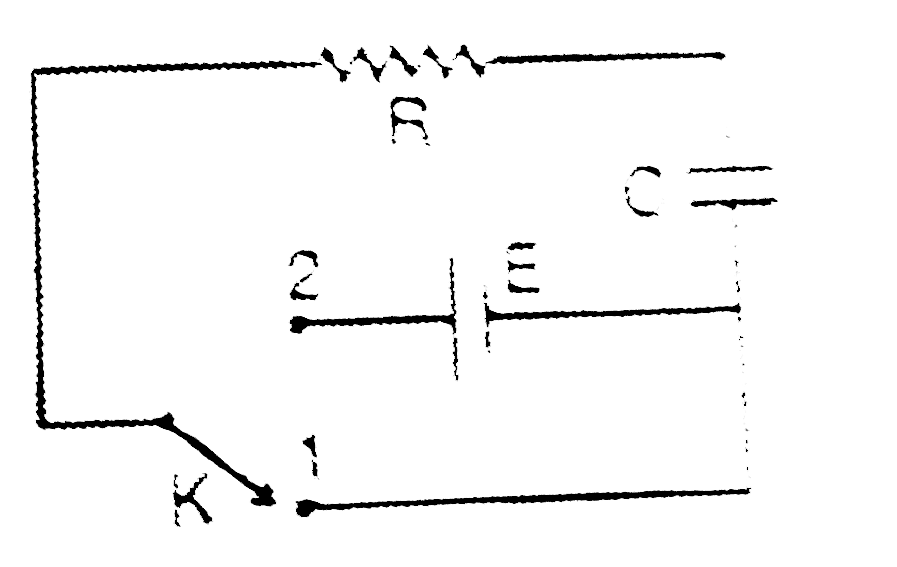
The charge on capacitor at t = 2RC second isACEBCE (1−1e)CCE (1e−1e2)DCE (1−1e+1e2)In the shown circuit involving a resistor of resistance RΩ, capacitor of capacitance C farad and an ideal cell of emf E volts, the capacitor is initially uncharged and the key is in position 1. At t = 0 second the key is pushed to position 2 for t0=RC seconds and then key is pushed back to position 1 for t0=RCseconds. This process is repeated again and again. Assume the time taken to push key from position 1 to 2 and vice verse to be negligible.
Then the variation of charge on capacitor with time is best represented byA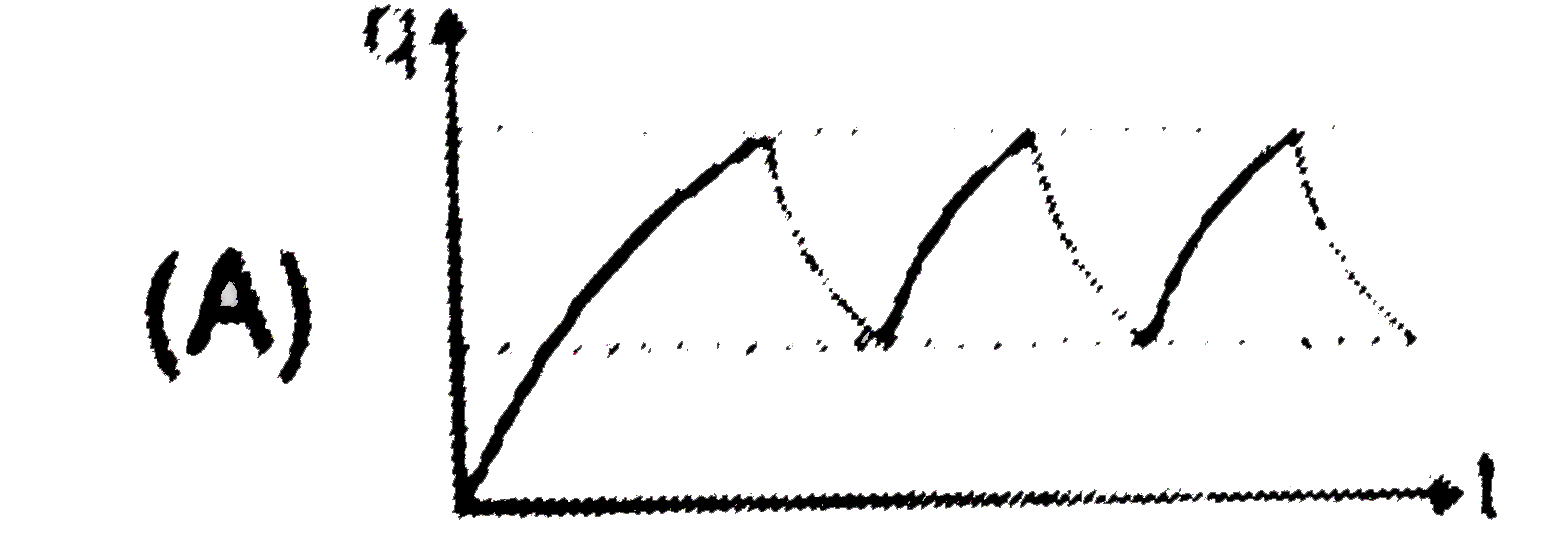 B
B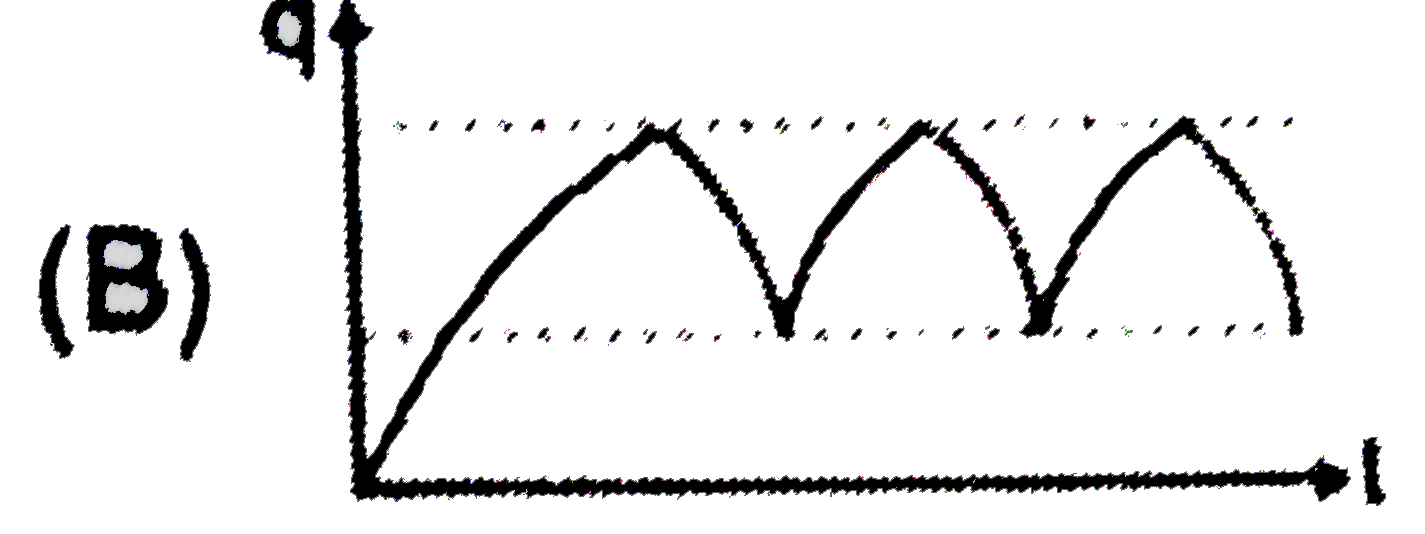 C
C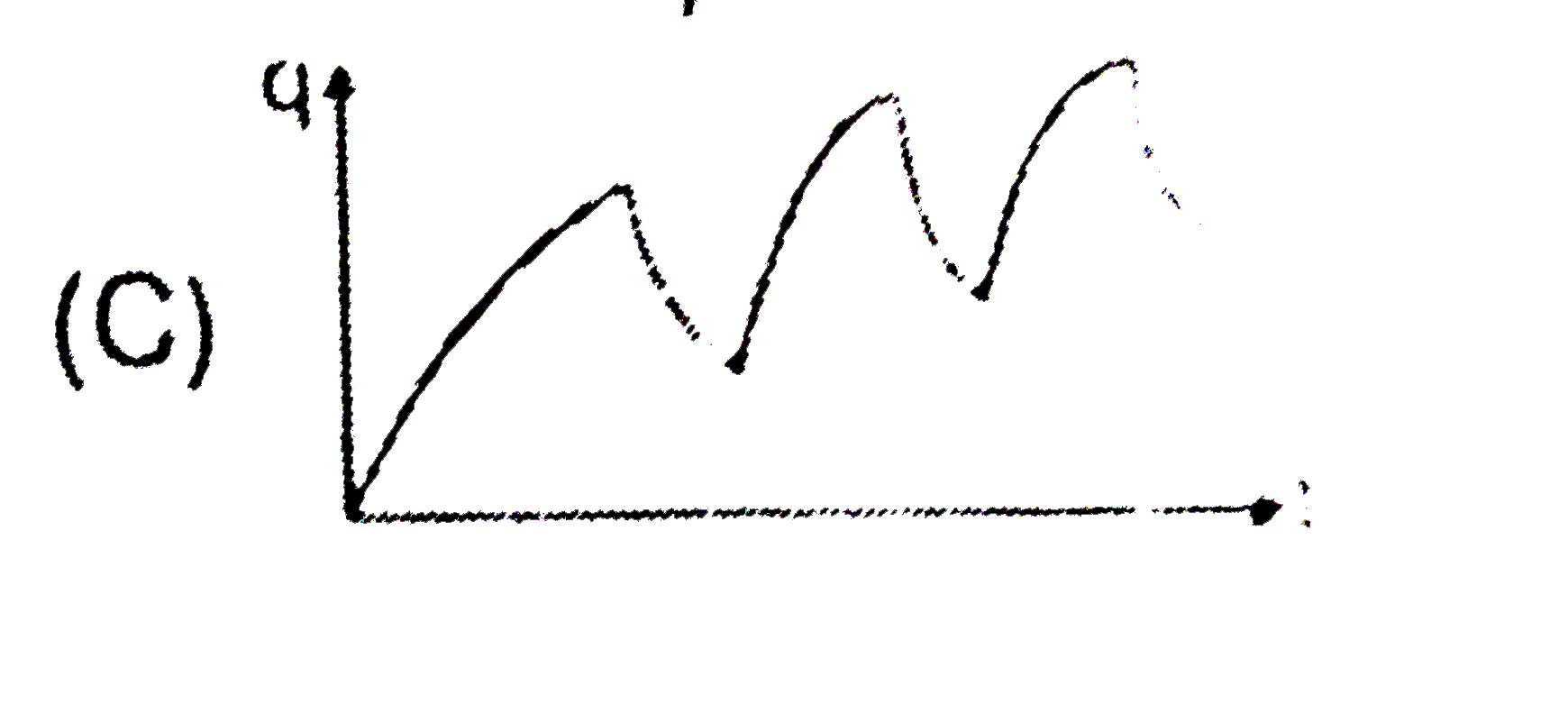 D
D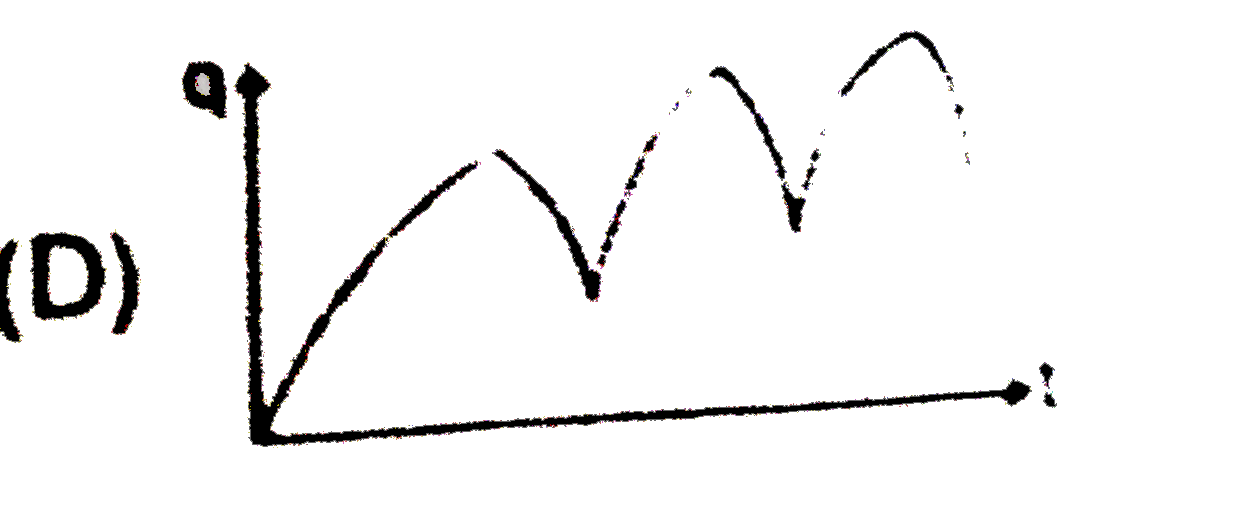
In the circuit shown key is closed at t=0 with capacitor initially uncharged.
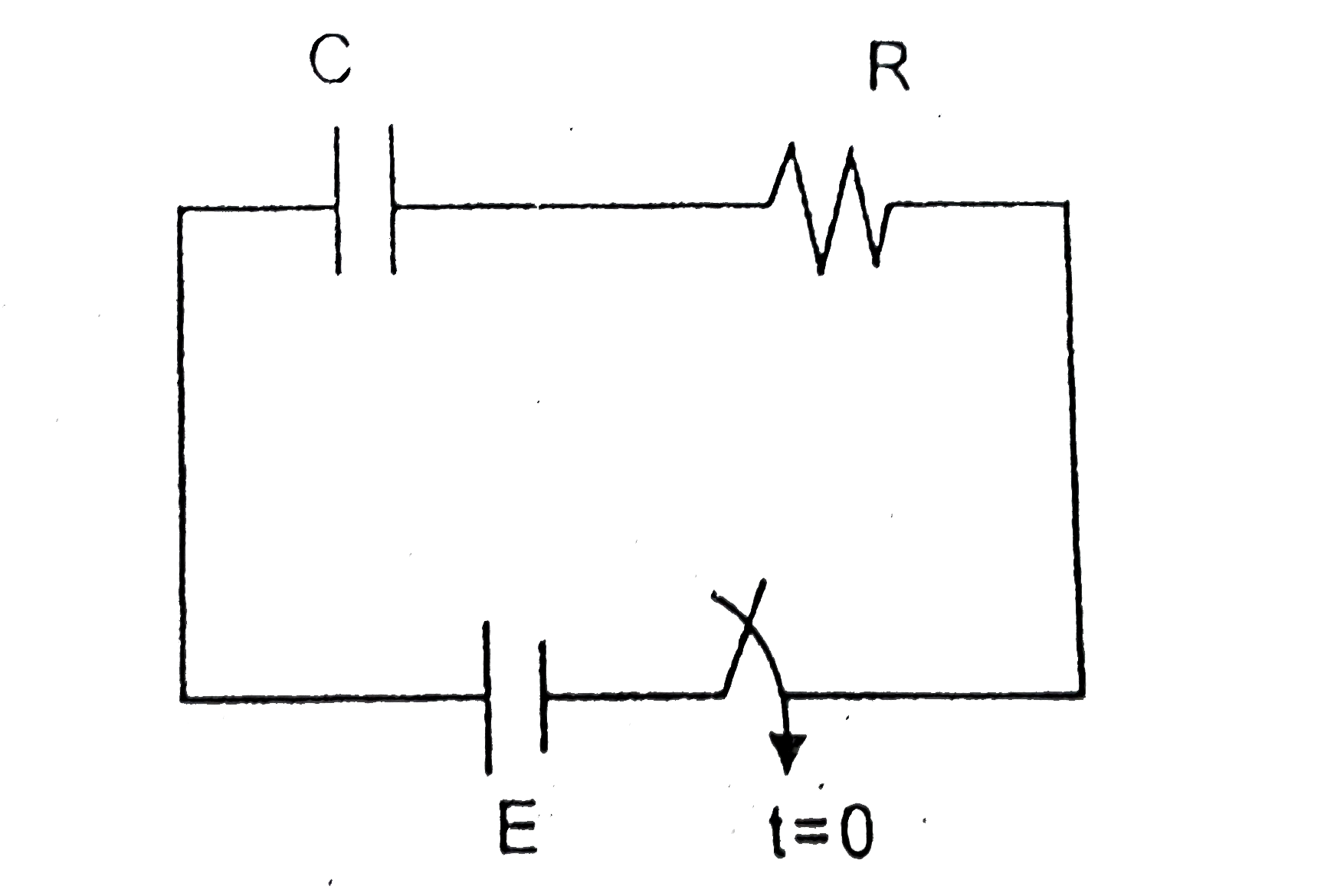 ARate of increase of capacitors energy, just after the switch is closed is E2RBMaximum power supplied by the cell is E2RCAt t=RCIn2, capacitor is charged up to half of the energy stored by it in steady stateDAt t=RCIn2, potential difference across capacitor is same that across resistor.
ARate of increase of capacitors energy, just after the switch is closed is E2RBMaximum power supplied by the cell is E2RCAt t=RCIn2, capacitor is charged up to half of the energy stored by it in steady stateDAt t=RCIn2, potential difference across capacitor is same that across resistor.
In the circuit shown key is closed at t=0 with capacitor initially uncharged.

View SolutionPassage-III
In the circuit shown, the battery is ideal with emf V. The capacitor is initially uncharged. The switch is closed at time t = 0
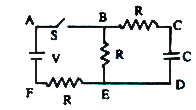
The current in the branch BE at t=∞ is
View SolutionIn the circuit shown the key (K) is closed at t=0, the current through the key at the instant t=10−3ln,2 is
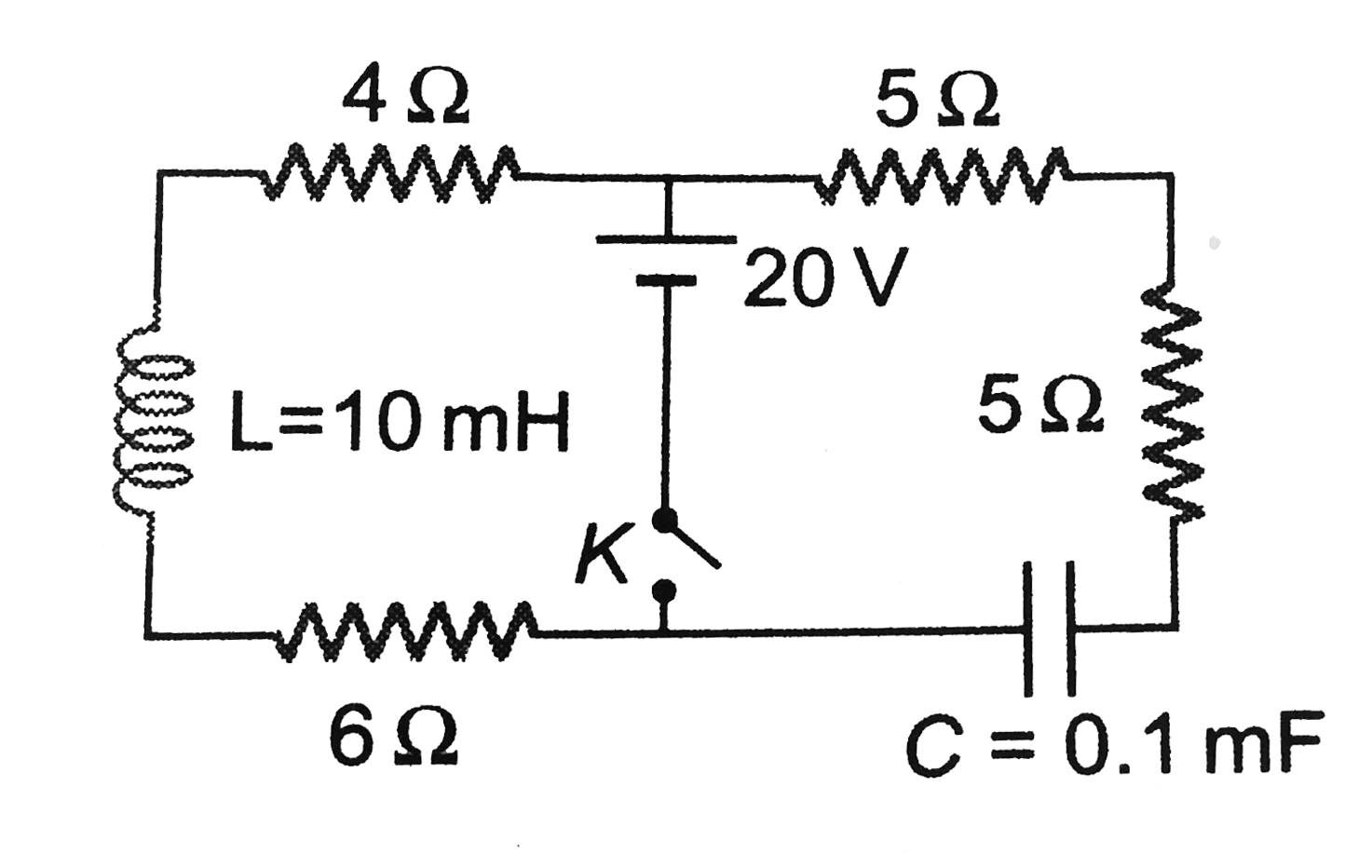
View SolutionIn the adjoining circuit, the capacitor is initially uncharged. The current through the resistor AB immediately after the key K is pressed is __________ mA.
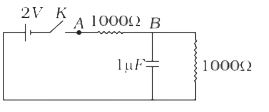
View SolutionIn the circuit shown the key (K) is closed at t=0, the current through the key at the instant t=10−3ln,2 is

View Solution
