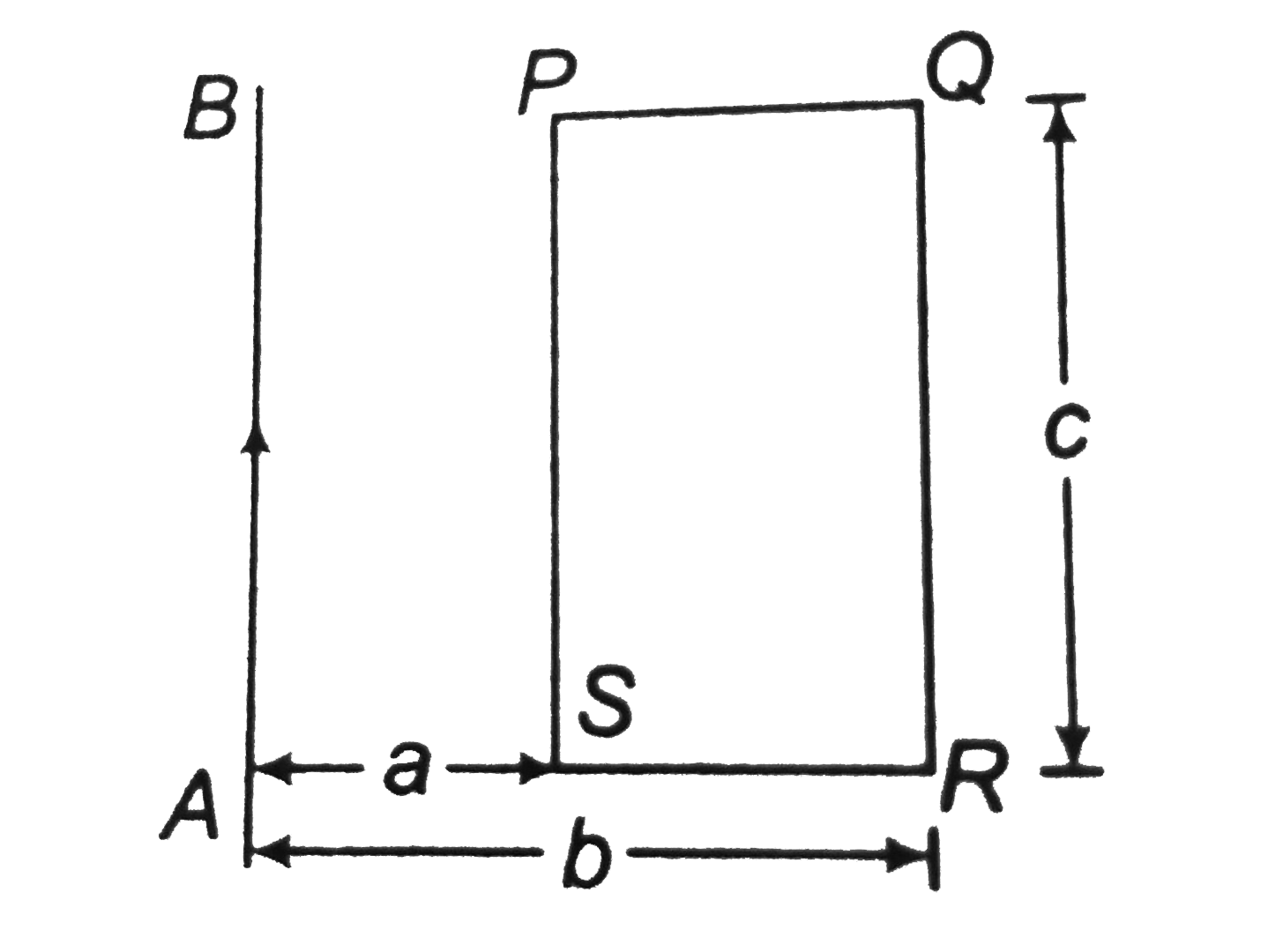
Find the mutual inductance between two rectangular loops, shown in the figure.
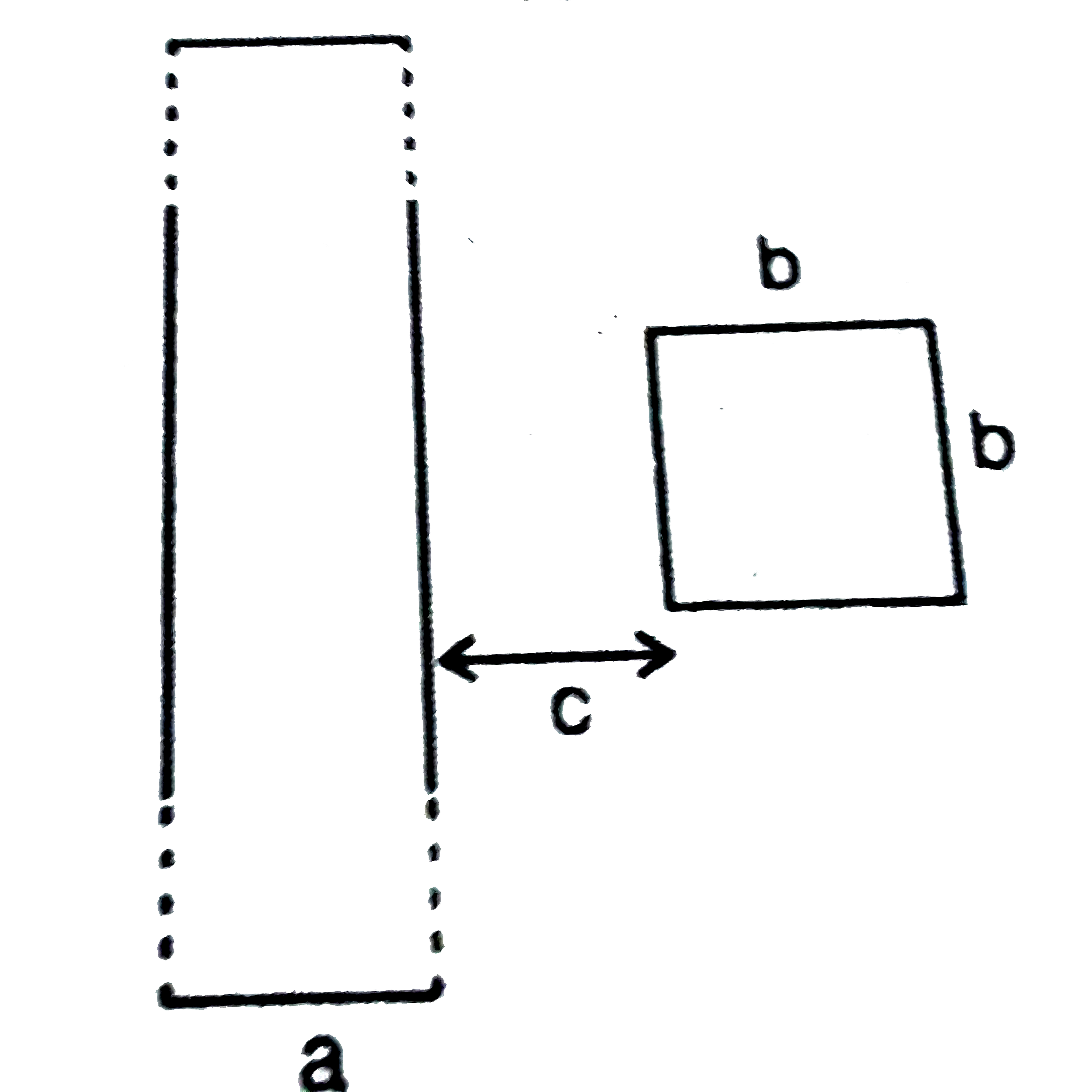
Video Solution
Text Solution
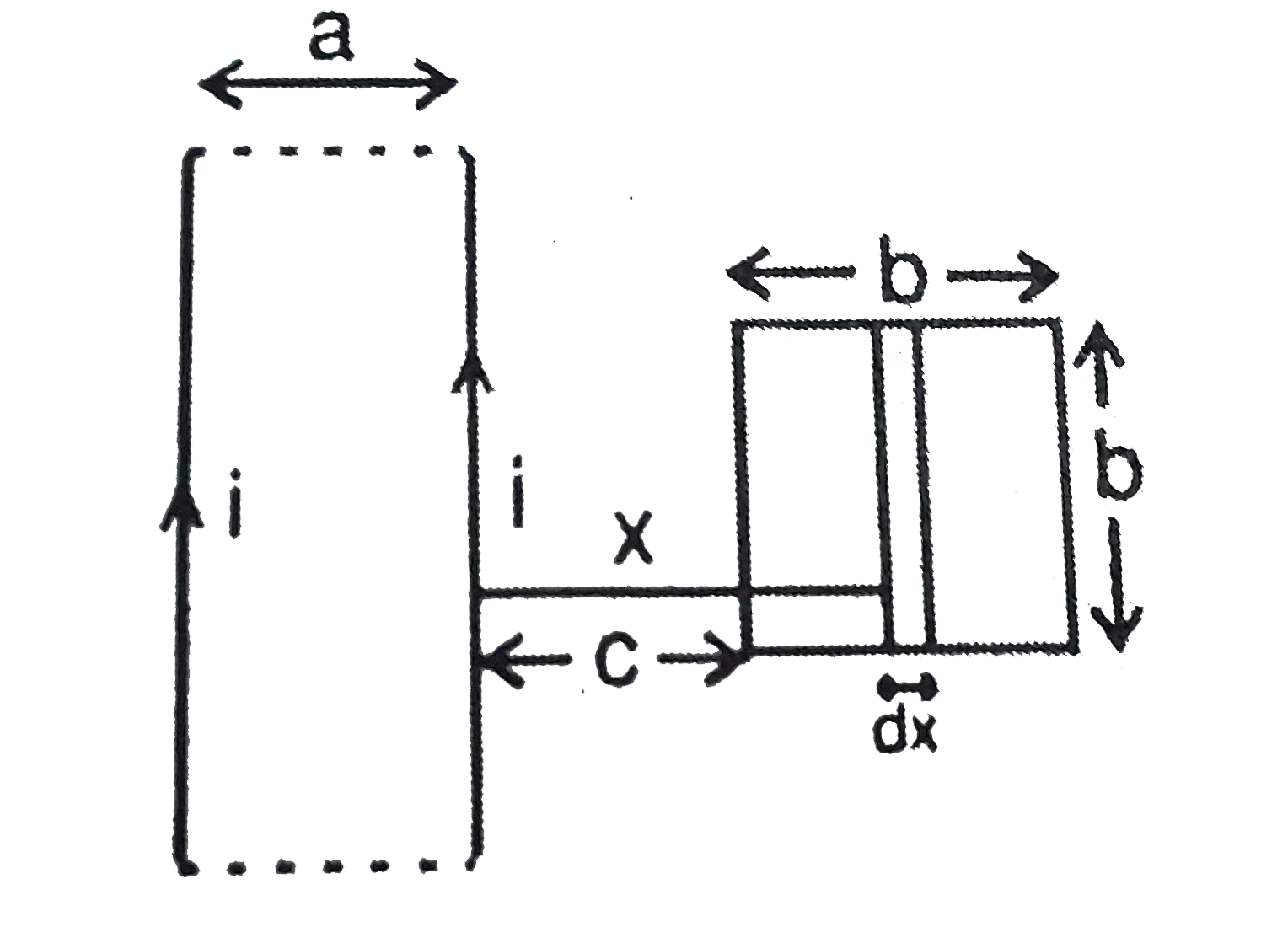
Let current i flow in the loop having ∞−by long sides. Consider a segment of width dx at a distance x as shown flux through the regent.
dϕ=[μ0i2πx−μ0i2π(x+a)]bdx⇒ϕ=∫c+bc[μ0i2πx−μ0i2π(x+a)]bdx=μ0ib2π[lnc+bc−lna+b+ca+c]
|
Updated on:21/07/2023
Similar Questions
Knowledge Check
- Question 1 - Select One
The mutual inductance between the rectangular loop and the long straight wire as shown in figure is M.
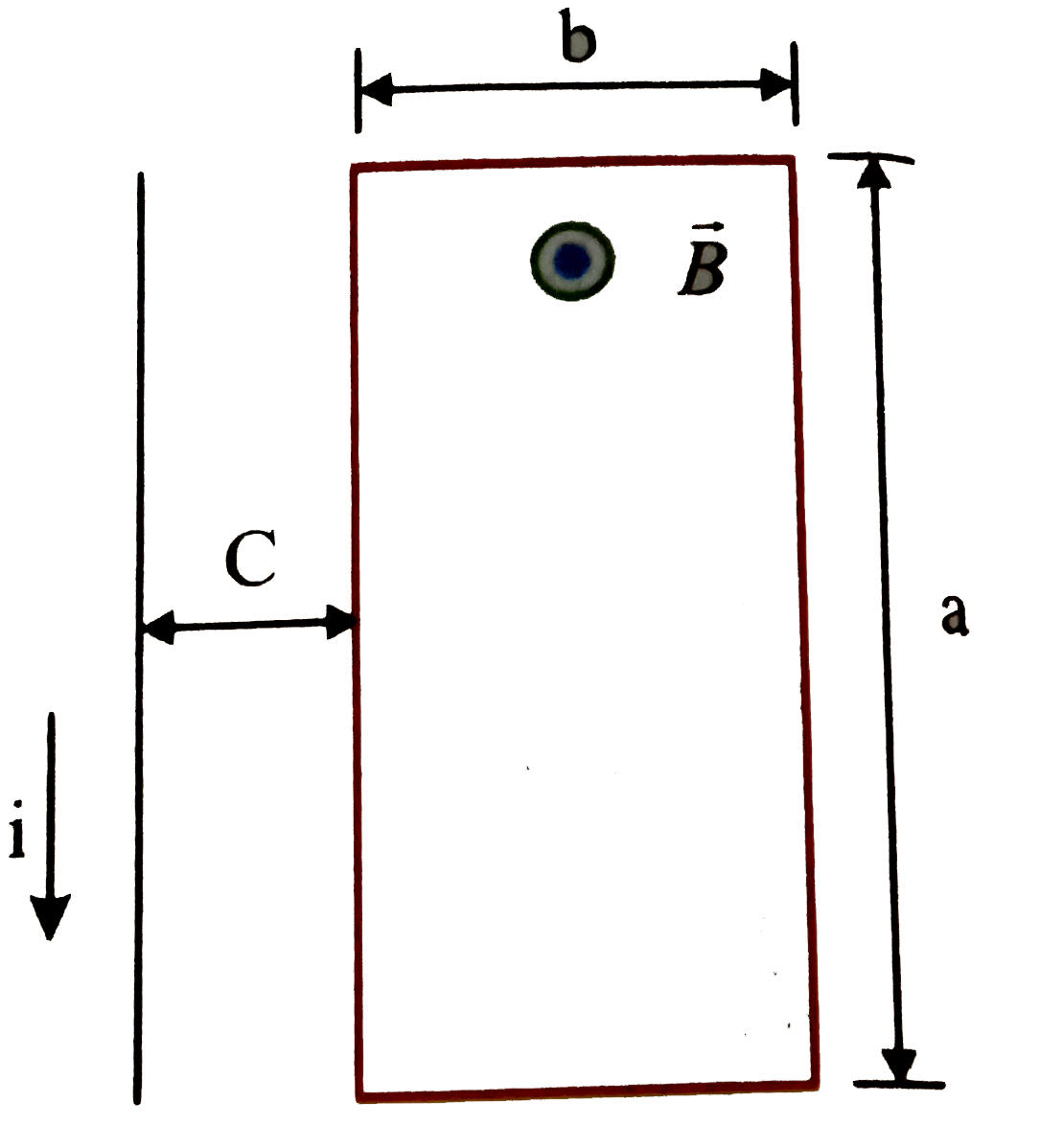 AM= zeroBM=μ0a2πln(1+cb)CM=μ0a2πln(a+bb)DM=μ0a2πln(1+bb)
AM= zeroBM=μ0a2πln(1+cb)CM=μ0a2πln(a+bb)DM=μ0a2πln(1+bb) - Question 2 - Select One
The mutual inductance between the rectangular loop and the long straight wire as shown in figure is M.
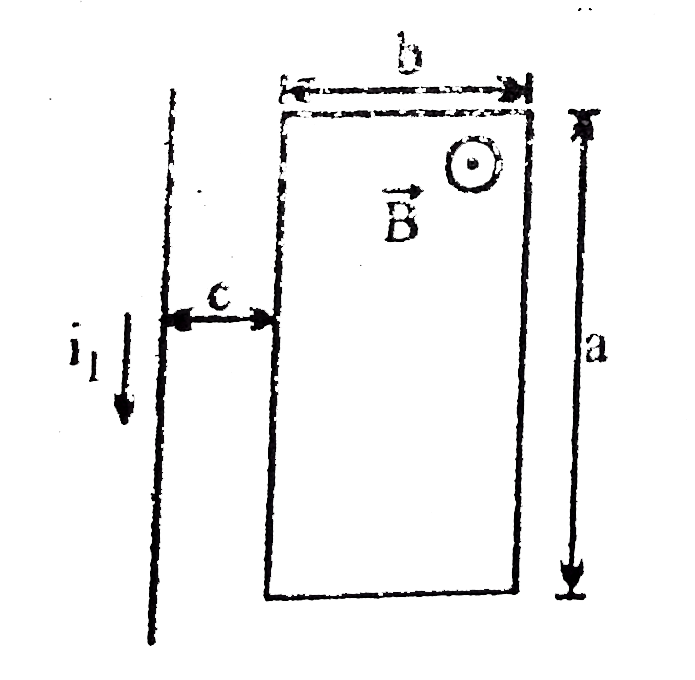 AM=ZeroBM=μ0a2πIn(1+cb)CM=μ0b2πIn(a+cb)DM=μ0a2πIn(1+bc)
AM=ZeroBM=μ0a2πIn(1+cb)CM=μ0b2πIn(a+cb)DM=μ0a2πIn(1+bc) - Question 3 - Select One
AB is an infinitely long wire placed in the plane of rectangular coil of dimension as shown in the figure. Calculate the mutual inductance of wire AB and coil PQRS
 Aμ0b2πlnabBμ0c2πlnbaCμ0abc2π(b−a)2DNone of these
Aμ0b2πlnabBμ0c2πlnbaCμ0abc2π(b−a)2DNone of these
Find the mutual inductance between the straight wire and the square loop of figure.
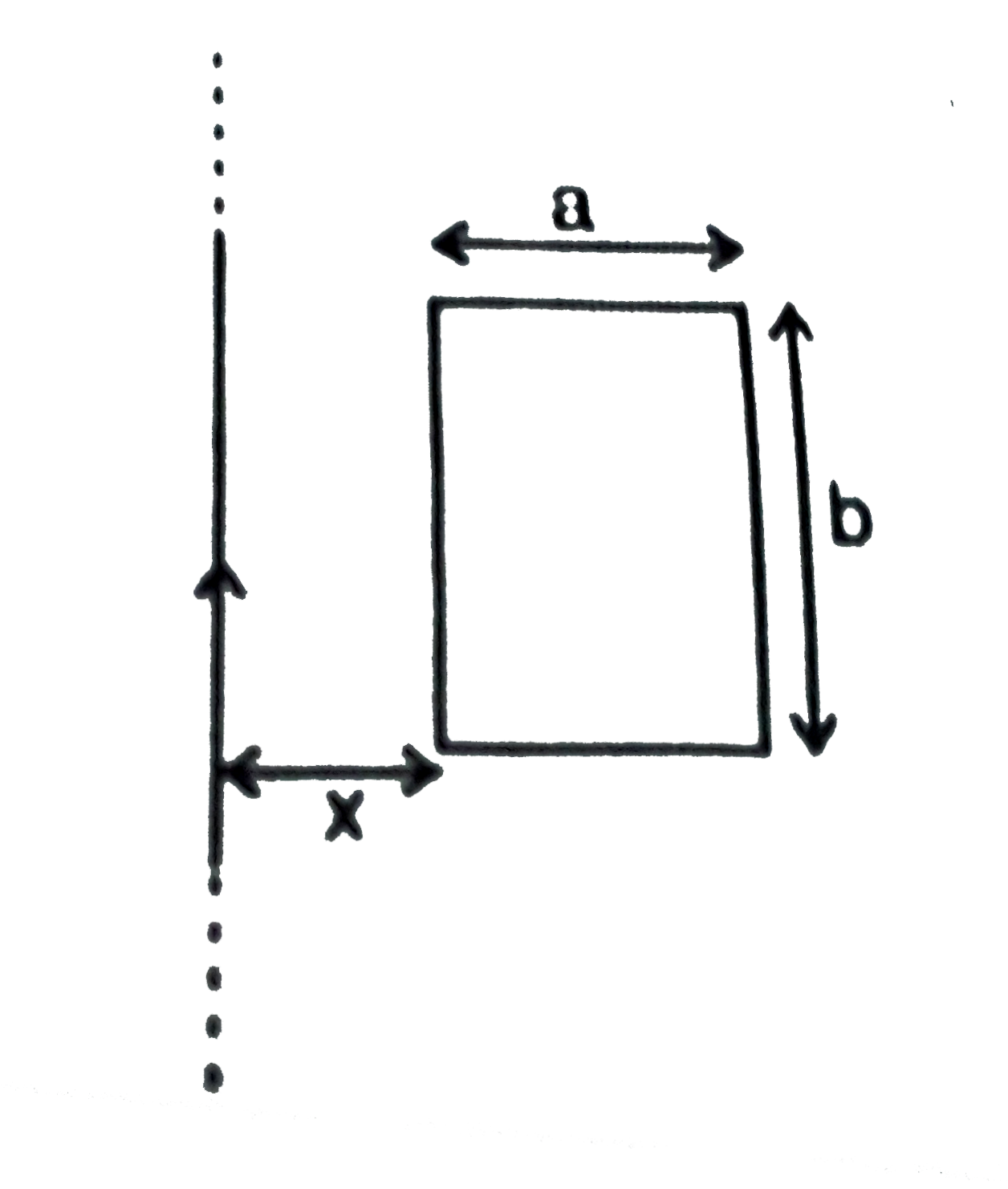
View SolutionFind the mutual inductance of a straight long wire and a rectangular loop, as shown in the figure.
 View Solution
View SolutionFigure. shows two concentric coplanar coils with radii a and b(a<<b). A current I=2t flows in the smaller loop. Neglecting self-inductance of the larger loop,
a. find the mutual inductance of the two coils,
b. find the emf induced in the larger coil,
c. if the resistance of the larger loop is R, find the current in it as a function of time.
 View Solution
View SolutionFind the magnetic field at the centre of the circular loop shown in figure.
 View Solution
View SolutionFind the magnetic field at the centre of the circular loop shown in figure.
 View Solution
View Solution

