The magnetic field in the cylinderical region shown in figure increases at a constant rate of 10.0mT/s Each side of the square loop abcd and defa has a length of 2.00 cm and resistance of 2.00Ω. Correctly match the current in the wire 'ad' in four different situations as listed in column-I with the values given in column-II
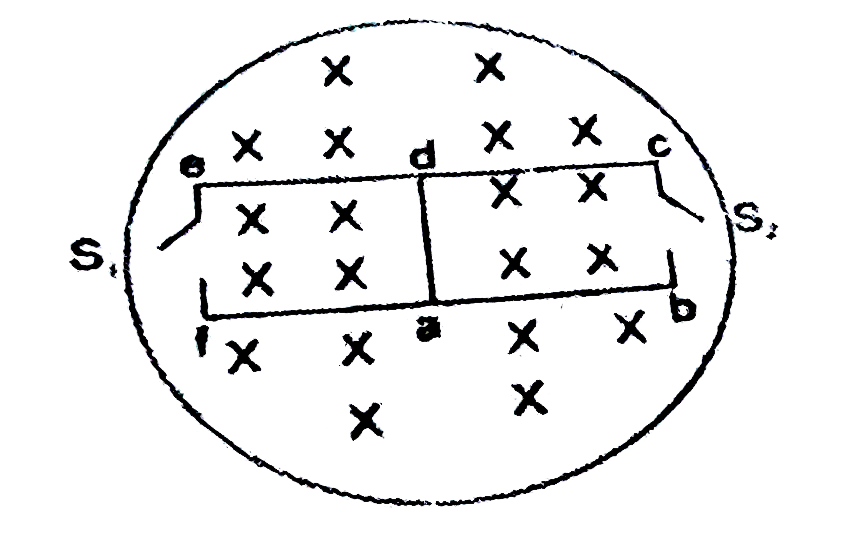
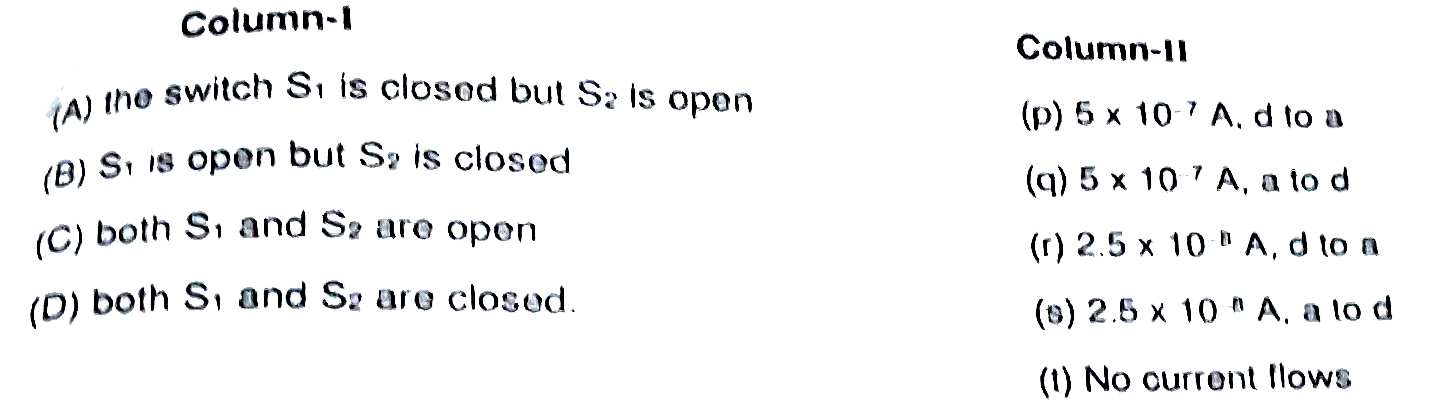
The correct Answer is:A, B, C, D
Similar Questions
The magnetic field in the cylindrical region shown in figure increase at a constant rate of 20mT/sec. Each side of the square loop ABCD has a length of 1cm and resistance of 4Ω. Find the current in the wire AB if the switch S is closed
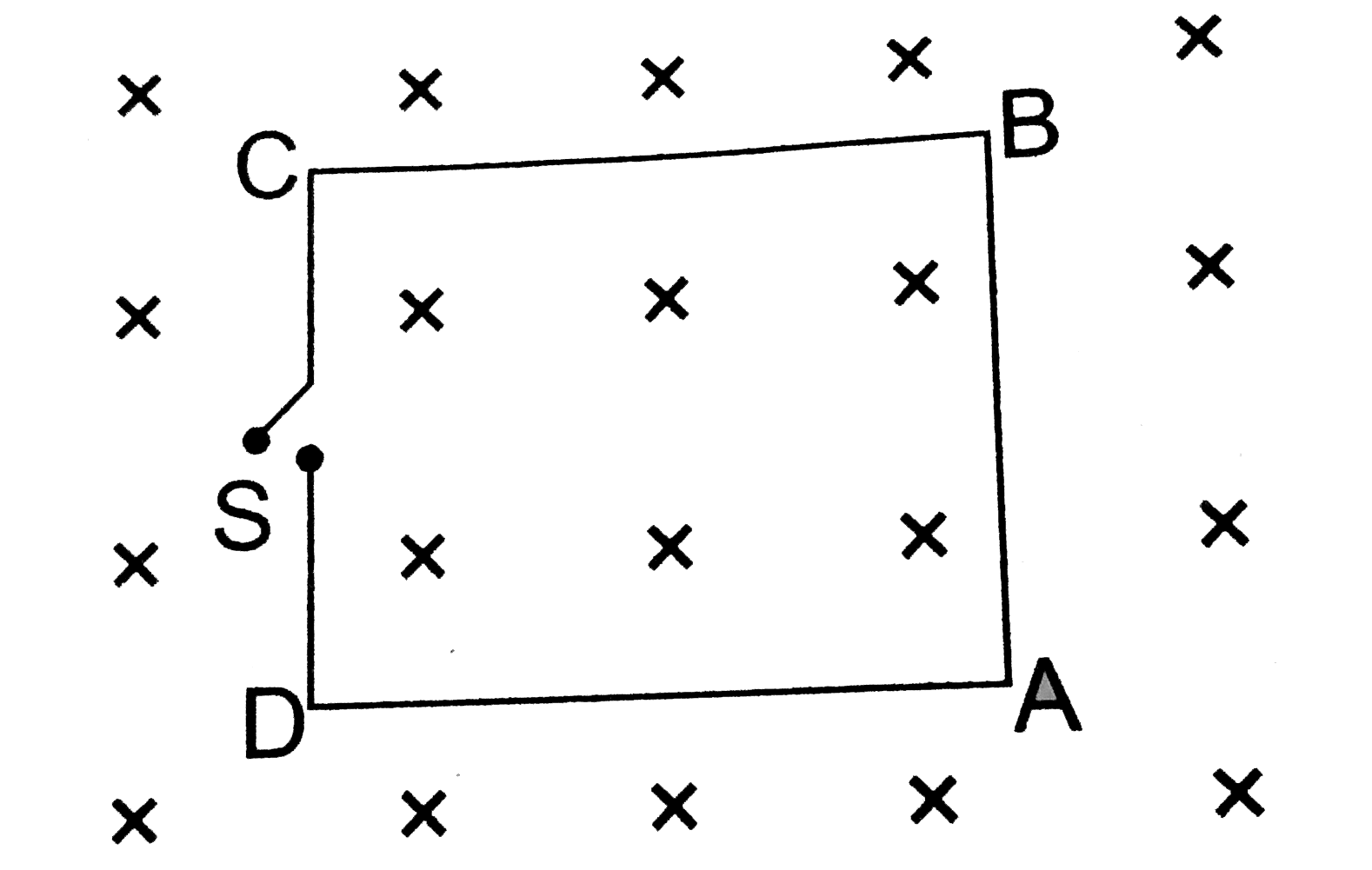
View SolutionThe magnetic field in the cylindrical region shown in figure increases at a constant rate of 20.0mT/sec . Each side of the square loop abcd and defa has a length of I.00cm and a resistance of 4.00Ω . Find the cuurent (magnitude and sense) in the wire ad if:
(a) the switch SI is closed but S2 is open
(b) SI is but S2 is closed
(c) both SI and S2 are open and
(d) both SI and S2 are closed

View Solution
Knowledge Check
The magnetic field in the cylindrical region shown in figure increase at a constant rate of 20mT/sec. Each side of the square loop ABCD has a length of 1cm and resistance of 4Ω. Find the current in the wire AB if the switch S is closed
 A1.25×10−7A,(anticlockwise)B1.25×10−7A (clockwise)C2.5×10−7A(anticlockwise)D2.5×10−7A(clockwise)
A1.25×10−7A,(anticlockwise)B1.25×10−7A (clockwise)C2.5×10−7A(anticlockwise)D2.5×10−7A(clockwise)A uniform magnetic field B exists in a cylindrical region of radius I0cm as shown in figure. A uniform wire or length 80cm and resistance 44.0Ω is bent into a square frame and is placed with one side along a diameter of the cylindrical region. if the magnetic field increases at a constant rate of 0.0I0T/sec , the current induced in the frame
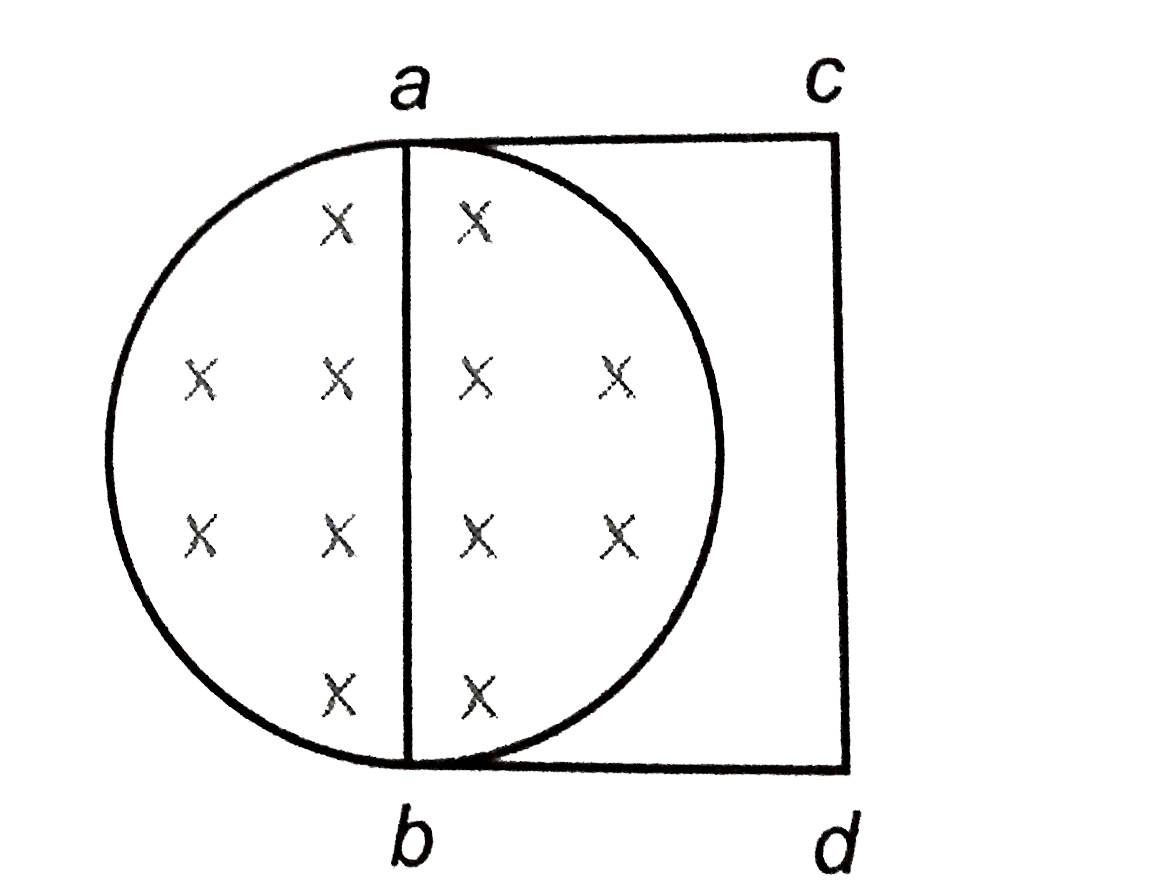 A3.9×10−5AB4.0×10−5AC4.1×10−5AD3.9×10−4A
A3.9×10−5AB4.0×10−5AC4.1×10−5AD3.9×10−4AA non - planar loop of conducting wire carrying a current I is placed as shown in the figure . Each of the straighrt sections of the loop is of the length 2a. The magnetic field due to this loop at the point P(a,0,a) points in the direction
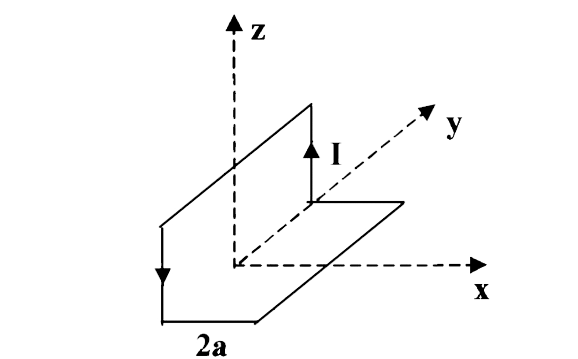 A1√2(−ˆj+ˆk)B1√3(−ˆj+ˆk+ˆi)C1√3(ˆi+ˆj+ˆk)D1√2(ˆi+ˆk)
A1√2(−ˆj+ˆk)B1√3(−ˆj+ˆk+ˆi)C1√3(ˆi+ˆj+ˆk)D1√2(ˆi+ˆk)
The magnetic field in the cylindrical region shown in increases at a constant rate of 20.0 mT/s. Each side of the square loop abcd and defa has a length of 1.00 cm and a resistance of 4.00Ω. Find the current (magnitude and sense) in the wire ad if (a) the switch S1 is closed but S2 is open, (b) S1 is open but S2 is closed, (c) both S1 and S2 are open and (d) both S1 and S2 are closed.

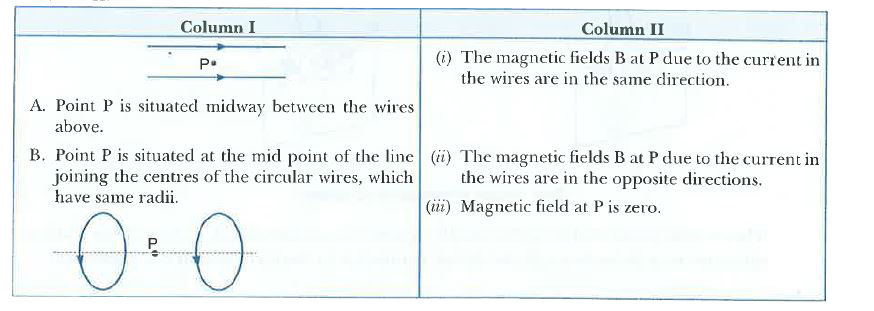
View SolutionTwo wires each carrying a steady current i are shown in two different configurations in column I. The magnetic field produced due to current in the wires is described in column II. Match the situations A and B in column I with all the correct statements in column II.
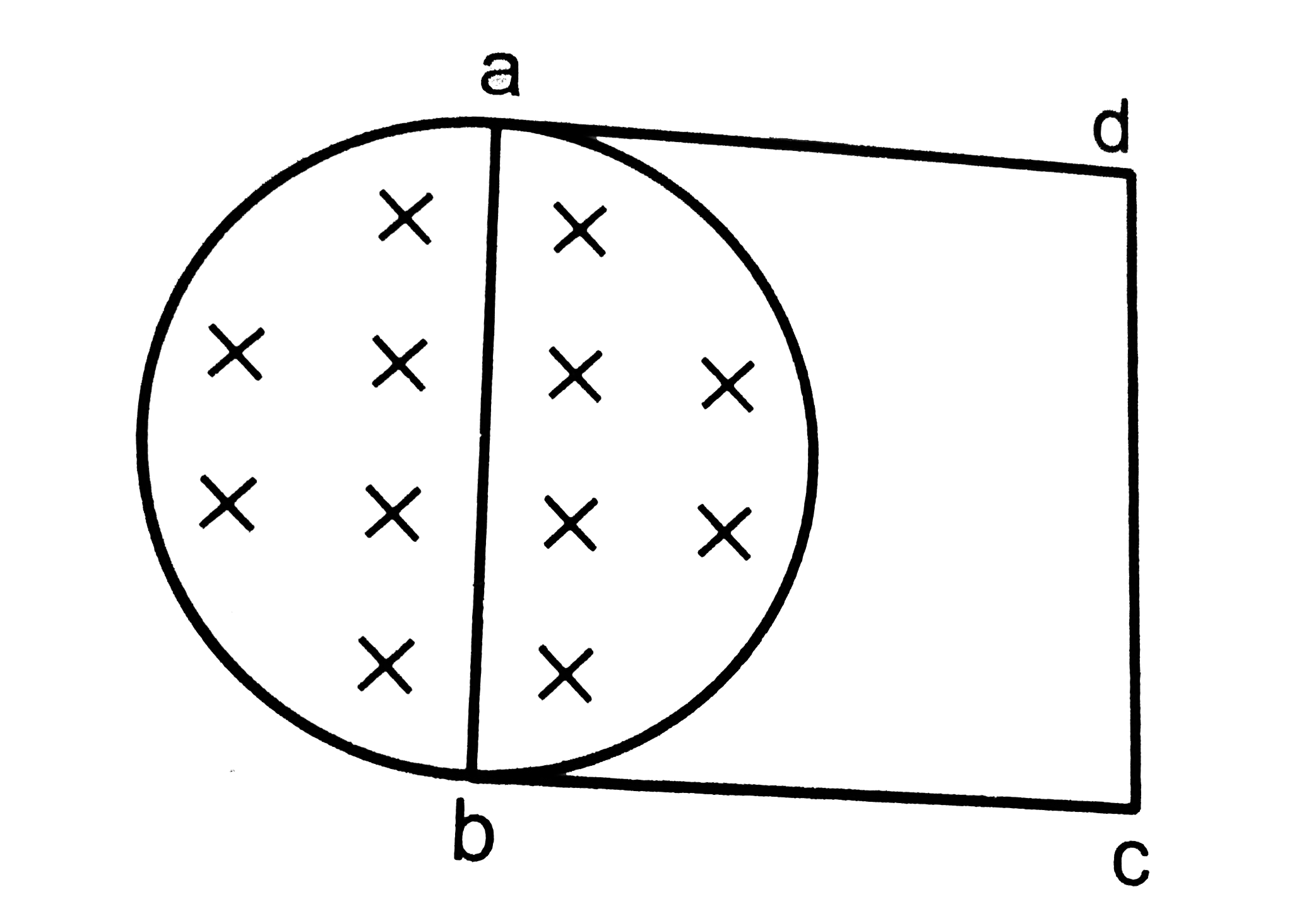
View SolutionA unifrom magnetic field B exists in a cylindrical region of radius 10 cm as shown in. A uniform wire of length 80 cm and resistance 4.0 Omega is bent into a square frame and is placed with one side along a diameter of the cylindrical region. If the magnetic field increases at a constant rate of 0.010 T/s, find the current induced in the frame.
View SolutionA unifrom magnetic field B exists in a cylindrical region of radius 1 cm as shown in figure. A uniform wire of length 16 cm and resistance 4.0Ω is bent into a square frame and is placed with one side along a diameters of the cylindrical region. If the magnetic field increaes at a constant rate of 1 T/s find the current induced in the frame.
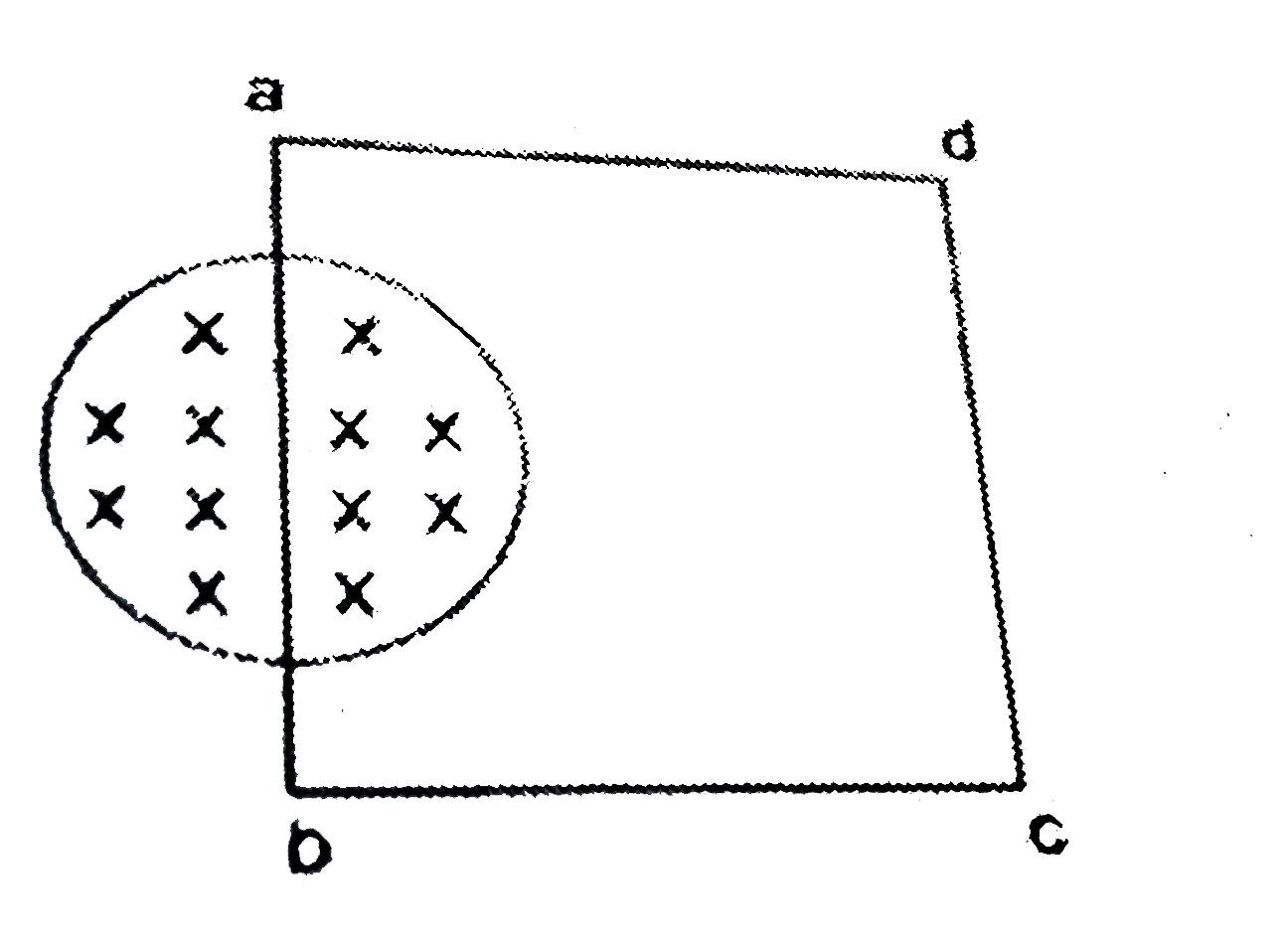
View SolutionA unifrom magnetic field B exists in a cylindrical region of radius 1 cm as shown in figure. A uniform wire of length 16 cm and resistance 4.0Ω is bent into a square frame and is placed with one side along a diameters of the cylindrical region. If the magnetic field increaes at a constant rate of 1 T/s find the current induced in the frame.

View Solution
