Two point charges and are separated by a distance cm. Find the magnitude and nature of the force between the two charges.
The correct Answer is:5400 N, attractive
To solve the problem of finding the magnitude and nature of the force between two point charges q1 and q2 separated by a distance x, we will use Coulomb's Law.
1. Identify the Charges and Distance:
- Given:
- q1=2×10−3C
- q2=−3×10−6C
- Distance x=10cm=0.1m
2. Coulomb's Law:
- The formula for the force F between two point charges is given by:
F=k|q1q2|r2
- Where k is Coulomb's constant, k=9×109N m2/C2 and r is the distance between the charges.
3. Substituting Values:
- Substitute the values of q1, q2, and r into the formula:
F=9×109|(2×10−3)(−3×10−6)|(0.1)2
4. Calculating the Product of Charges:
- Calculate |q1q2|:
|q1q2|=|(2×10−3)(−3×10−6)|=6×10−9C2
5. Calculating r2:
- Calculate r2:
r2=(0.1)2=0.01m2
6. Substituting Back into the Force Equation:
- Now substitute |q1q2| and r2 back into the force equation:
F=9×1096×10−90.01
7. Calculating the Force:
- Simplifying the equation:
F=9×109×6×10−9×100=54N
8. Determining the Nature of the Force:
- Since q1 is positive and q2 is negative, the force between them is attractive.
Final Answer:
- The magnitude of the force between the two charges is 54N and the nature of the force is attractive.
- Given:
- q1=2×10−3C
- q2=−3×10−6C
- Distance x=10cm=0.1m
- The formula for the force F between two point charges is given by:
F=k|q1q2|r2
- Where k is Coulomb's constant, k=9×109N m2/C2 and r is the distance between the charges.
- Substitute the values of q1, q2, and r into the formula:
F=9×109|(2×10−3)(−3×10−6)|(0.1)2
- Calculate |q1q2|:
|q1q2|=|(2×10−3)(−3×10−6)|=6×10−9C2
- Calculate r2:
r2=(0.1)2=0.01m2
- Now substitute |q1q2| and r2 back into the force equation:
F=9×1096×10−90.01
- Simplifying the equation:
F=9×109×6×10−9×100=54N
- Since q1 is positive and q2 is negative, the force between them is attractive.
- The magnitude of the force between the two charges is 54N and the nature of the force is attractive.
Topper's Solved these Questions
- BOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - Exercise-1 Section (B)12videos
ELECTROSTATICS
- BOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - Exercise-1 Section (C)14videos
ELECTROSTATICS
- BOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - Problems22videos
ELECTROSTATICS
- BOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - A.l.P19videos
ELECTROMAGNETIC INDUCTION
- BOOK - RESONANCECHAPTER - EXPERIMENTAL PHYSICSEXERCISE - PART -II10videos
EXPERIMENTAL PHYSICS
Similar Questions
Two point charges, q1=10×10−8Candq2=−2×10−8C, are separated by a distance of 60 cm in air.
Find at what distance from the 1st charge q1, would the electric potential be zero.View SolutionTwo point charges, q1=10×10−8C and q2=−2×10−8C are separated by a distance of 60 cm in air.
(i) Find at what distance from the 1st charge, q1, would the electric potential be zero.
(ii) Also calculate the electrostatic potential energy of the system.View Solution
Knowledge Check
- Question 1 - Select One
Two point charges q1=+2C and q2=−1C are separated by a distance d. The position on the line joining the two charges where a third charge q=+1C will be in equilibrium is at a distance
Ad√2 from q1 and q2Bd√2 from q1 away from q2Cd√2−1 from q2 between q1 and q2Dd√2−1 from q2 away from q1 - Question 2 - Select One
Two positive point charges q1=16μC and q2=4μC are separated in vacuum by a distance of 3.0m. Find the point on the line between the charges where the net electric field is zero.-
A2m from q1B1m from q1C2m from q2D0.5m from q1 - Question 3 - Select One
If point charges Q1=2×10−7C and Q2=3×10−7C are at 30 cm separation, then find electrostatic force between them
A2×10−3NB6×10−3NC5×10−3ND1×10−3N
Two point charges, q1=10×10−8Candq2=−2×10−8C, are separated by a distance of 60 cm in air.
Also calculate the electrostatic potential energy of the system.View SolutionTwo point charges 20×10−6Cand−4×10−6C are separated by a distance of 50cm in air, Find (i) the point on line joining the charges, where the electrostatic potential is zero (ii) Also, Calculate the electrostatic potential energy of the system.
View SolutionTwo point charged bodies q1=3μC, and q2=4μC are separated by 2 m in air. Find the magnitude of electrostatic force between them.
View SolutionTwo point charge q1=−10×10−6C and q2=15×10−6 are 40 cm apart as shown in figure. Find the potential difference between the point P and Q.
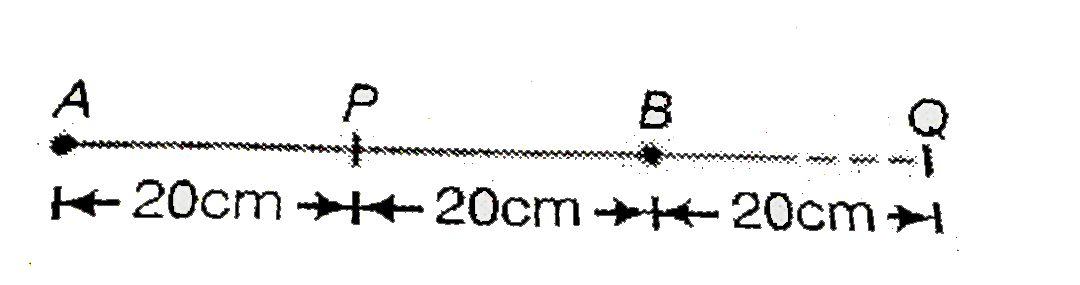 View Solution
View SolutionTwo point charges +5×10−19Cand+20×10−19C are separated by a distance of 2m. The electric field intensity will be zero at a distance d=………. From the charges of 5×1019C.
View Solution

