Two forces of mangitude F1 and F2 are acting on an object.The magnitude of the net force Fnet on the object will be in the range.
F1≤Fnet≤F2
|F1−F2|/2≤Fnet≤(F1+F2)/2
|F1−F2|≤Fnet≤F1+F2
|F1−F2|≥Fnet|F1+F2|
The correct Answer is:C
To determine the range of the net force Fnet acting on an object due to two forces F1 and F2, we can use the principles of vector addition. The net force can vary depending on the angle between the two forces. Here’s how we can find the minimum and maximum values of Fnet:
Step 1: Understand the Forces
We have two forces acting on an object:
- Force F1
- Force F2
Step 2: Use the Resultant Force Formula
The magnitude of the resultant force Fnet when two forces are acting at an angle θ can be calculated using the formula:
Fnet=√F21+F22+2F1F2cosθ
Step 3: Determine Maximum Value of Fnet
To find the maximum value of Fnet, we need to maximize the term cosθ. The maximum value of cosθ is 1 (which occurs when the forces are in the same direction). Thus:
Fnet, max=√F21+F22+2F1F2⋅1=√(F1+F2)2=F1+F2
Step 4: Determine Minimum Value of Fnet
To find the minimum value of Fnet, we need to minimize the term cosθ. The minimum value of cosθ is -1 (which occurs when the forces are in opposite directions). Thus:
Fnet, min=√F21+F22+2F1F2⋅(−1)=√F21+F22−2F1F2=√(F1−F2)2=|F1−F2|
Step 5: Conclusion
The magnitude of the net force Fnet will be in the range:
|F1−F2|≤Fnet≤F1+F2
We have two forces acting on an object:
- Force F1
- Force F2
The magnitude of the resultant force Fnet when two forces are acting at an angle θ can be calculated using the formula:
Fnet=√F21+F22+2F1F2cosθ
To find the maximum value of Fnet, we need to maximize the term cosθ. The maximum value of cosθ is 1 (which occurs when the forces are in the same direction). Thus:
Fnet, max=√F21+F22+2F1F2⋅1=√(F1+F2)2=F1+F2
To find the minimum value of Fnet, we need to minimize the term cosθ. The minimum value of cosθ is -1 (which occurs when the forces are in opposite directions). Thus:
Fnet, min=√F21+F22+2F1F2⋅(−1)=√F21+F22−2F1F2=√(F1−F2)2=|F1−F2|
The magnitude of the net force Fnet will be in the range:
|F1−F2|≤Fnet≤F1+F2
Topper's Solved these Questions
- KINEMATICSBOOK - FIITJEECHAPTER - KINEMATICSEXERCISE - MCQ (MULTIPLE CORRECT)
15 Videos
- KINEMATICSBOOK - FIITJEECHAPTER - KINEMATICSEXERCISE - ASSERTION-REASON MCQ ( SINGLE CORRECT)
8 Videos
- KINEMATICSBOOK - FIITJEECHAPTER - KINEMATICSEXERCISE - SECTION (II) : (SUBJECTIVE )
33 Videos
- HEAT AND TEMPERATUREBOOK - FIITJEECHAPTER - HEAT AND TEMPERATUREEXERCISE - NUMERICAL BASES QUESTIONS
1 Videos
- LAWS OF MOTIONBOOK - FIITJEECHAPTER - LAWS OF MOTIONEXERCISE - COMPREHENSION-III
2 Videos
Similar Questions
Two forces are acting together on an object. The magnitude of their resultant is minimum when the angle between the forces is
View SolutionTwo forces F1 and F2 are acting on a body. One force is double that of the other force and the resultant is equal to the greater force. Then the angle between the two forces is
View Solution
Knowledge Check
Two forces F1 and F2 are acting on a rod abc as shown in figure.
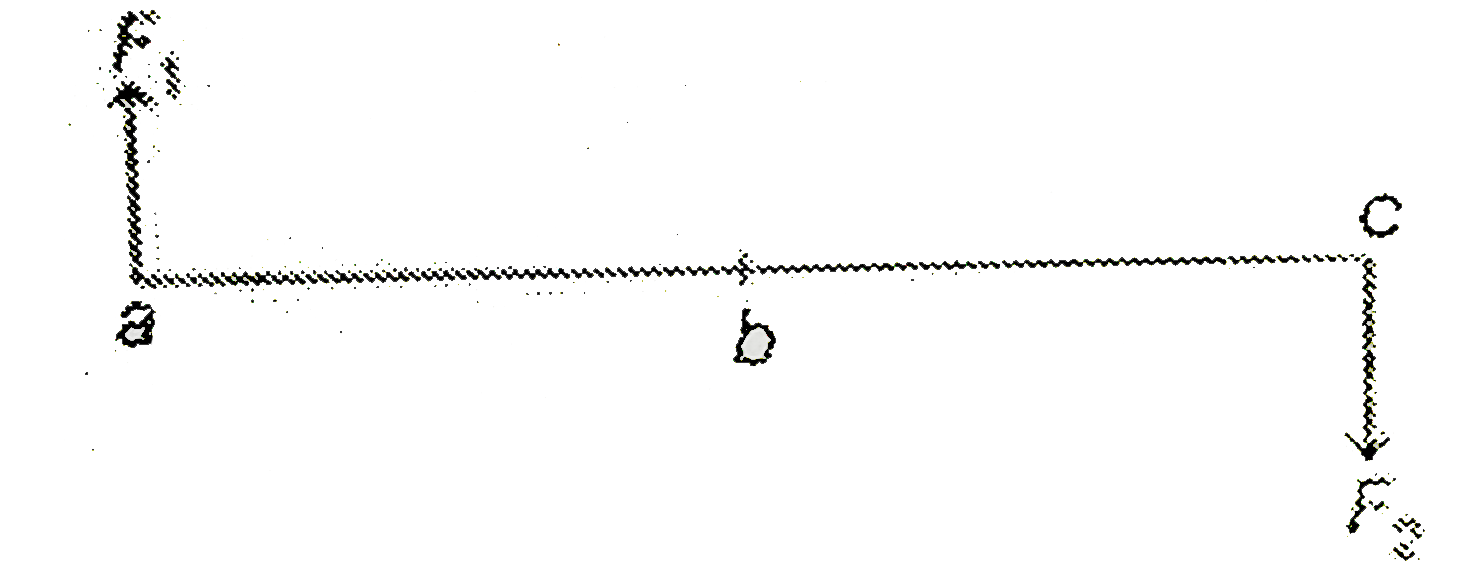 Aif F1=F2 then τa=τb=τc ( for both forces)Bif F1=F2 then τa=τc≠τb ( for both forces)Cif F1≠F2 then τa≠τb≠τc ( for both forces)Dif F1≠F2 then τa=τc≠τb ( for both forces)
Aif F1=F2 then τa=τb=τc ( for both forces)Bif F1=F2 then τa=τc≠τb ( for both forces)Cif F1≠F2 then τa≠τb≠τc ( for both forces)Dif F1≠F2 then τa=τc≠τb ( for both forces)A body is acted upon by two forces of manitudes F1=√2NandF2=3N which are inclined at 45∘ to each other. There magnitude of resultant force acting on the body is
A17 NB11 NC√17ND√11NA block is kept at rest on a rought ground as shown. Two force F1and F2 are acting on it. If we increased either of the two force F1and F2, force of friction acting on the block will increase.
By increasing F1, normal reaction from ground will increase.
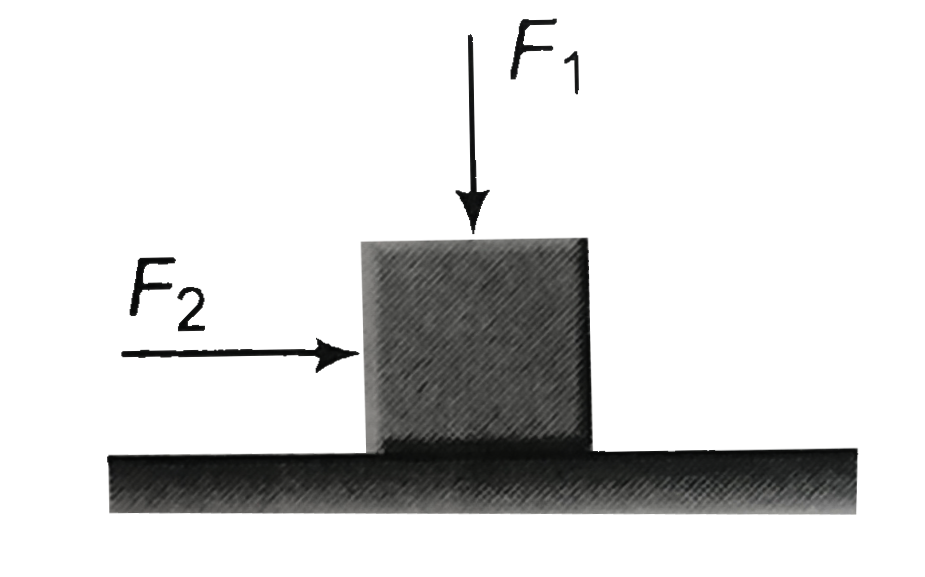 AIf both Accertion and Reason are true and the reason is correct explanation of the Assertion.BIf both Accertion and Reason are true but reason is not the correct explanation of Assertion.CIf Assertion is true, but the Reason is false.DIf Assertion is false but the Reason is true.
AIf both Accertion and Reason are true and the reason is correct explanation of the Assertion.BIf both Accertion and Reason are true but reason is not the correct explanation of Assertion.CIf Assertion is true, but the Reason is false.DIf Assertion is false but the Reason is true.
Two forces with equal magnitudes F act on a body and the magnitude of the resultant force is F /3. The angle between the two forces is
View SolutionAssertion:− when two non parallel forces F1 and F2 act on a body. The magnitude of the resultant force acting on the is less than the sum of F1 and F2
Reason:− In a triangle, any side is less then the sum of the other two sides.
View SolutionTwo force F1 and F2 are acting on a body. One force is double that of the other force and the resultant is equal to the greater force. Then the angle between the two forces is :-
View SolutionTwo forces, with equal magnitude F, act on a body and the magnitude of the resultant force is F3. The angle between the two forces is:
View SolutionTwo forces with equal magnitudes F act on a body and the magnitude of the resultant force is F /3. The angle between the two forces is
View Solution
