Considering the following general mechanism , which applies to many thermal decompositions and isomerisations.
Here represent the intermediate species formed during the process. if the first step is slow and rate determining, the rate law is
On the other hand, if is large, and thus is in rapid equilibrium with A, we have,
Using steady state approximation, the general rate law for the system can be found out as follows
Rate of production of rate of destruction of
or
Since the rate of reaction is
A practical example of the application of the steady state approximation is the decomposition of as follows
It follows the following mechanism
The overall order of the reaction , is
2
1
0
The correct Answer is:B
Topper's Solved these Questions
- ATOMIC STRUCTUREBOOK - FIITJEECHAPTER - ATOMIC STRUCTUREEXERCISE - COMPREHENSION -III
3 Videos
- ATOMIC STRUCTUREBOOK - FIITJEECHAPTER - ATOMIC STRUCTUREEXERCISE - COMPREHENSION -IV
3 Videos
- ATOMIC STRUCTUREBOOK - FIITJEECHAPTER - ATOMIC STRUCTUREEXERCISE - COMPREHENSION -I
3 Videos
- ALKYL AND ARYL HALIDESBOOK - FIITJEECHAPTER - ALKYL AND ARYL HALIDESEXERCISE - NUMERICAL BASED QUESTIONS
1 Videos
- CARBOHYDRATES, AMINO ACIDS, POLYMERS AND PRACTICAL ORGANIC CHEMISTRYBOOK - FIITJEECHAPTER - CARBOHYDRATES, AMINO ACIDS, POLYMERS AND PRACTICAL ORGANIC CHEMISTRYEXERCISE - EXERCISE
4 Videos
Similar Questions
The numerical value of a solubility product Ksp is measured by experiment.For example, we could determine Ksp for CaSO4 by adding on excess of solid CaSO4 to water, string the mixture to give a saturated solution of CaSO4 and then measuring the concentration of Ca2+ and SO−4 in the saturated solution. Value of Ksp are unaffected by the presence of other ions in solution. At 27∘C experiemntal value of Ksp is 2.4×10−5M2.
Will a precipitate for if equal volumes of 0.08 M CaCl2 and 0.02 M Na2SO4 are mixed ?
View SolutionThe molecular orbital with the lowest eenrgy is filled first. Thus σ(1s) is filled first where as 'σ⋅(2p) isfilled in thelast, also the maximum number of electron in bonding and antibonding molecular orbitals are according to Pauli and Hund's rule. As an electron in an antibonding molecular orbital cancels out the stability introduced by the electron in a bonding molecular orbital, it means that in order for bonding of atoms to occur there should be an excess of bonding electron sover antibonding electrons. In case where the number of bonding and antibonding electrons are equal, no bond will be formed between the atoms. With the help of above discussion, we can define easily bond order, relative bond length, relative stability and magnetic properties for a molecule.
In an antibonding molecular orbital, there is a line between the two probability contours of hydrogen atoms. This is called
View SolutionA laser ( light amplification of stimulated emission of radiation ) provides a coherent ( in phase ) nearly monochromatic source of high intensty light. Lasers are used in eye surgery, CD/ DVD players basic research, etc. some modern eye lasers can be "tuned" to emit a desired wavelength. The following table shows the λ ( nm ) , v(s−1) and E ( J) but some of the data is missing :
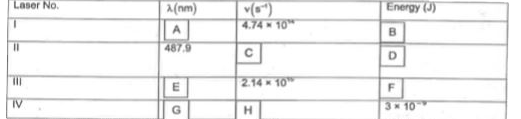
The value of A and H will be ......................... and ............................. respectively.
View SolutionCl−O∣∣C−OC2H5−(1)'X'RMgX−−−−−−−−−→(2)NH4Cl3∘alcohol
View SolutionTreatment of (+)− glucose with HIO4 gives results that confirm its aldohexose structure. What product should be formed, and how much HIO4 should be consumed.
View SolutionMechanism of the reaction is:
Ak1−−→B,2Ak2−−→C+D
What is −d[A]dt ?
View SolutionConisder the reaction mechanism: A_(2) overset(k_(eq))hArr 2A ("fast") (where A is the intermediate.) A+B underset(k_(1))rarr P (slow) The rate law for the reaction is
View SolutionA consecutive reaction, A overset(K_(1))(rarr)B overset(K_(2))(rarr)C is characterised by:
View SolutionAssertion: In Ak1−−→Bk2−−→C
If half-life of A is very less as compared to B, so the net reaction is A→C with rate constant (k1×k2)
Reason: Slowest step is the rate determining step so B→C is rate determining step.
View SolutionA hypothetical reaction : A_(2)+B_(2)rarr 2AB Follows mechanism as given below : A_(2) overset(k_(c))hArr A+A................( fast )" "(k_(C)- is equilibrium constant ) A+B_(2)overset(k_(1))rarrAB+B...............(" slow")" "(k_(1)- rate constant ) A+B underset(K_(2))overset(k_(2))hArrAB...............( fast )" "(k_(2),k_(3)- are rate constant ) Give the rate law.
View SolutionIn the sequence of reaction,
Lk1−−→Mk2−−→Nk3−−→O
k3>k2>k1
The rate determining step of the reaction is :
View SolutionThe mechanism of the reaction A+2B rarr C+D is: Step I: A+B underset(k_(2))overset(k_(1))hArr I Step II: B+I overset(k_(3))rarr C+D In the first step is a fast equilibruim , then the incorrect statement is :
View SolutionDecomposition of A to give B follows a two step mechansim as shown. From the mechanism identify the option which is incorrect. A rarr 1.5 B" " (overall reaction) Steps-1: A underset(k_(2))overset(k_(1))hArr B+C" " (fast) Step-2: A+C overset(k_(3))rarr 2B" " (slow)
View SolutionConsidering the following general mechanism , which applies to many thermal decompositions and isomerisations. A + M rarr underset( K_(-1))overset(K_(1))(rarr) A^(*) + M A^(*) overset( K_(2))(rarr) B + C Here A^(*) represent the intermediate species formed during the process. if the first step is slow and rate determining, the rate law is ( d [B])/(dt) = K_(1) [ A][M] On the other hand, if K_(-1) is large, and thus A^(*) is in rapid equilibrium with A, we have, ([A^(*)])/([A]) = (K_(1))/( K_(-1)) ( d[B])/( dt) = K_(2) [A^(*)] = ( K_(1) K_(2))/(K_(-1)) [A] Using steady state approximation, the general rate law for the system can be found out as follows : Rate of production of A^(*) = rate of destruction of A^(*) K_(1) [A][M] = K_(-1) [A^(*)][M]+K_(2) [ A^(*)] or [A^(*)] = ( K_(1) [A][M])/(K_(-1)[M]+K_(2)) Since the rate of reaction is ( d[B])/( dt) = K_(2) [A^(*)] :. ( d [B])/( dt) = ( K_(1) K_(2) [A][M])/(K_(-1) [ M ] + K_(2)) A practical example of the application of the steady state approximation is the decomposition of N_(2)O_(5) as follows : 2N_(2) O_(5) rarr 4NO_(2) + O_(2) It follows the following mechanism : N_(2)O_(5) underset( K_(-1))overset( K_(1))(rarr)NO_(2) + NO_(3) NO_(3) + NO_(2) overset( K_(3))(rarr) 2NO_(2) For the reaction : 2N_(2)O_(5) rarr 4NO_(2) + O_(2) ( d[O_(2) ] ) /( dt ) = K_("exp") [ N_(2) O_(5) ] The K_("exp.") is given by the expression :
View SolutionConsidering the following general mechanism , which applies to many thermal decompositions and isomerisations. A + M rarr underset( K_(-1))overset(K_(1))(rarr) A^(*) + M A^(*) overset( K_(2))(rarr) B + C Here A^(*) represent the intermediate species formed during the process. if the first step is slow and rate determining, the rate law is ( d [B])/(dt) = K_(1) [ A][M] On the other hand, if K_(-1) is large, and thus A^(*) is in rapid equilibrium with A, we have, ([A^(*)])/([A]) = (K_(1))/( K_(-1)) ( d[B])/( dt) = K_(2) [A^(*)] = ( K_(1) K_(2))/(K_(-1)) [A] Using steady state approximation, the general rate law for the system can be found out as follows : Rate of production of A^(*) = rate of destruction of A^(*) K_(1) [A][M] = K_(-1) [A^(*)][M]+K_(2) [ A^(*)] or [A^(*)] = ( K_(1) [A][M])/(K_(-1)[M]+K_(2)) Since the rate of reaction is ( d[B])/( dt) = K_(2) [A^(*)] :. ( d [B])/( dt) = ( K_(1) K_(2) [A][M])/(K_(-1) [ M ] + K_(2)) A practical example of the application of the steady state approximation is the decomposition of N_(2)O_(5) as follows : 2N_(2) O_(5) rarr 4NO_(2) + O_(2) It follows the following mechanism : N_(2)O_(5) underset( K_(-1))overset( K_(1))(rarr)NO_(2) + NO_(3) NO_(3) + NO_(2) overset( K_(3))(rarr) 2NO_(2) The steady state aproximateion is applied to a reaction intermediate but never to a reactant or product because
View Solution
