Prove that $[lim_{x \to 0} \frac{\tan^{- 1} x}{x}] = 0,$ where $[.]$ represents the greatest integer function.

Video Solution

Text Solution

Verified by Experts

See the graph of y=x and $\tan^{- 1} x$ in the figure:

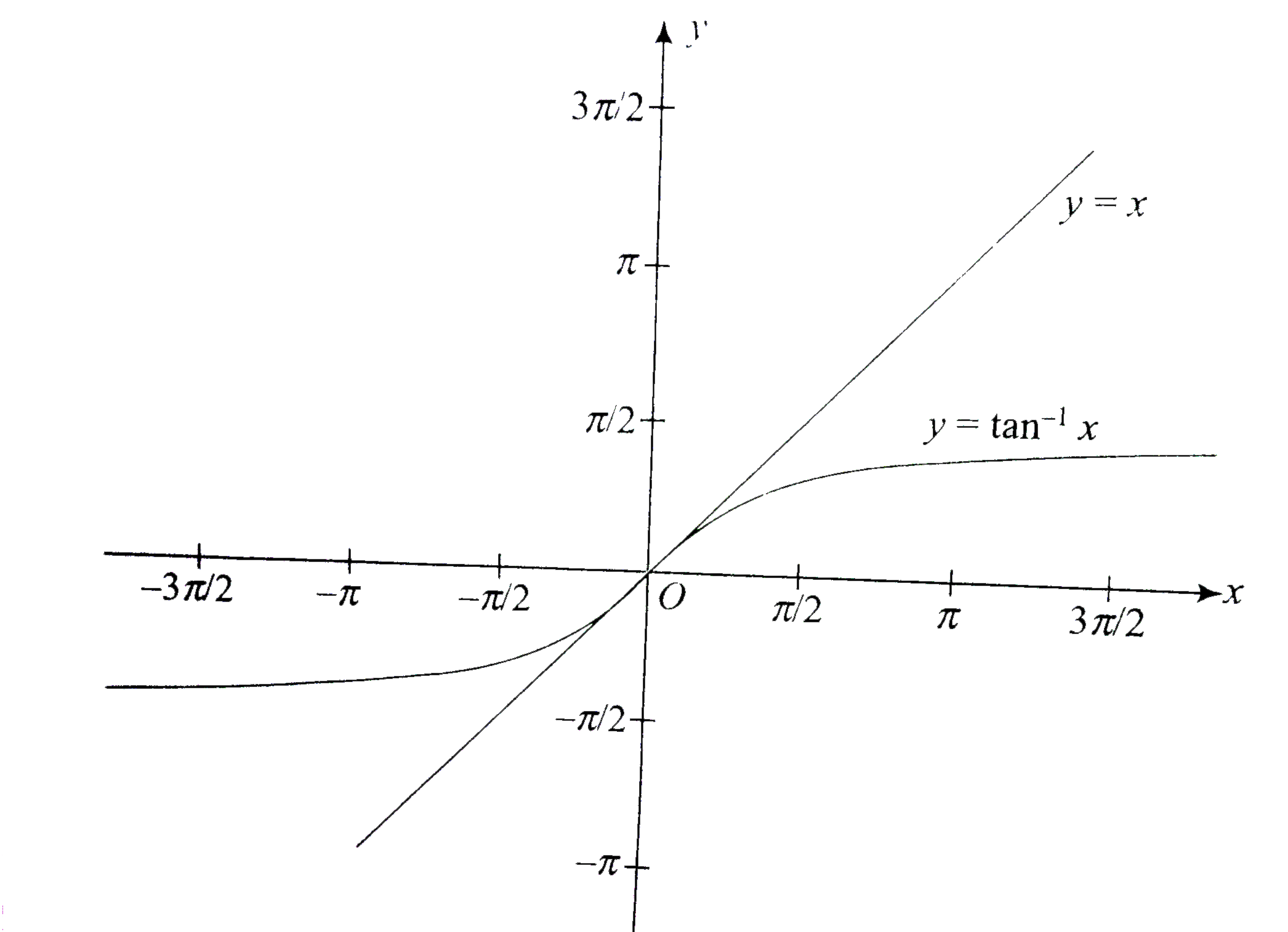
From the figure, when $x \to 0^{+}$ , graph of y=x is above the graph
So, $\tan^{- 1} x < x or \frac{\tan^{- 1} x}{x} < 1$
$\Rightarrow lim_{x \to 0^{+}} \frac{\tan^{- 1} x}{x} = 1^{-}$
$\Rightarrow [lim_{x \to 0^{+}} \frac{\tan x}{x}] = 0$
Thus $[lim_{x \to 0} \frac{\tan^{- 1} x}{x}] = 0$

|

Updated on:21/07/2023

Class 12 MATHS LIMITS AND DERIVATIVES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
$lim x \to 0 [(1 - e^{x}) \frac{sin x}{| x |}]$ is (where $[.]$ represents the greatest integer function )
A1
B2
C3
Dnone of these

CENGAGE-LIMITS-Question Bank

Home
Ask Doubt
Profile