The centroid of an equilateral triangle is (0,0). If two vertices of the triangle lie on x+y $= 2 \sqrt{2}$ , then find all the possible vertices fo triangle.

Text Solution

Verified by Experts

The correct Answer is: $(- 2 \sqrt{2}, - 2 \sqrt{2}), (\sqrt{2} - \sqrt{6}, \sqrt{2} + \sqrt{6}), (\sqrt{2} + \sqrt{6}, \sqrt{2} - \sqrt{6})$

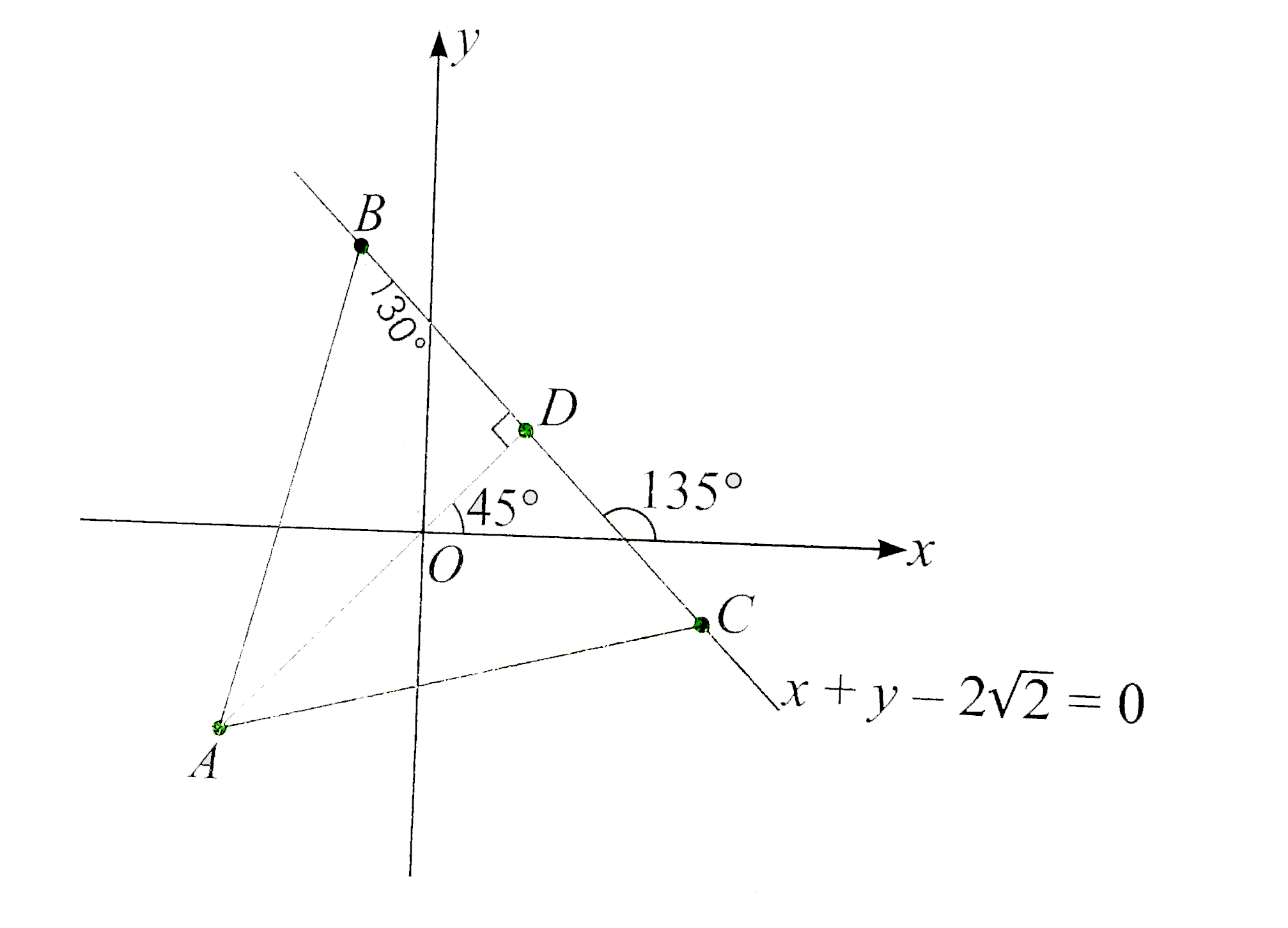
As shown in the figure, equilateral triangle ABC is such that B and C lie on the given line $x + y - 2 \sqrt{2} = 0$ .
AD is perpendicular to BC, which passes through centroid.
$O D = \frac{∣ ∣ 0 + 0 - 2 \sqrt{2} ∣ ∣}{\sqrt{2}} = 2$
$∴ OA=4 (As centroid divides median in the ratio 2 : 1)$
Slope of AD is 1, i.e., tan $45^{\circ}, where 45^{\circ}$ is the inclination of AD with x-axis.
$∴ A \equiv (0 - 4 cos 45^{\circ}, 0 - sin 45^{\circ}) \equiv (- 2 \sqrt{2}, - 2 \sqrt{2})$
$and D \equiv (0 + 2 cos 45^{\circ}, 0 + 2 sin 45^{\circ}) \equiv (\sqrt{2}, \sqrt{2})$
$Now, D C = D B = 2 cot 30^{\circ} = 2 \sqrt{3}$
Therefore, B $\equiv (\sqrt{2} + 2 \sqrt{3} cos 135^{\circ}, \sqrt{2} + 2 \sqrt{3} cos 135^{\circ})$
$\equiv (\sqrt{2} - \sqrt{6}, \sqrt{2} + \sqrt{6})$
$And C \equiv (\sqrt{2} - 2 \sqrt{3} cos 135^{\circ}, \sqrt{2} - 2 \sqrt{3} cos 135^{\circ})$
$\equiv (\sqrt{2} + \sqrt{6}, \sqrt{2} - \sqrt{6})$

Updated on:21/07/2023

|

Class 12 MATHS STRAIGHT LINES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Two vertices of an equilateral triangle are origin and (4, 0). What is the area of the triangle ?
AA sq units
BV3 sq units
C $4 \sqrt{3}$ sq units
D2V3 sq units

CENGAGE-STRAIGHT LINES-Exercise 2.2

Home
Ask Doubt
Profile