Suppose α,β,γandδ are the interior angles of regular pentagon, hexagon, decoagon and dedecogon respectively, find the values of |cosα+secβ+cosγ+cosesδ|. Assume that all polygons are regular.
The correct Answer is:√52
Step by step video, text & image solution for Suppose alpha, beta, gammaand delta are the interior angles of regular pentagon, hexagon, decoagon and dedecogon respectively, find the values of |cosalpha +sec beta+cos gamma+"coses"delta|. Assume that all polygons are regular. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Suppose α,β,γandδ are the interior angles of regular pentagon, hexagon, decagon, and dodecagon, respectively, then the value of |cosαsecβcosγcosecδ| is _________
View SolutionFind the measure of each interior angle of a regular
(i) pentagon (ii) hexagon (iii) octagon (iv) polygon of 12 sides
View Solution
Knowledge Check
If α,β,γ,δ be four angles of a cyclic quadrilateral taken in clockwise direction then the value of (2+Σcosαcosβ) will be:
Asin2α+sin2βBcos2γ+cos2δCsin2α+sin2δDcos2β+cos2γIf the normals at α,β,γ and δ on an ellipse are concurrent then the value of (σcosα)(σsecα) I
A2B4C6Dnone of theseIf cos(α+β)sin(γ+δ)=cos(α−β)sin(γ−δ) then the value of cotαcotβcotγ, is
AcotαBcotβCcot deltaDcot(α+β+γ+δ)
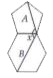
Polygon A is a regular pentagon and polygon B is a regular hexagon. Find the value of x.
View SolutionWhat is the sum of all interior angles of a regular
(i) pentagon (ii) hexagon (iii) nonagon (iv) polygon of 12 sides ?
View SolutionIf α,β,γ,δ be four angles of a cyclic quadrilateral taken in clockwise direction then the value of (2+∑cosαcosβ) will be:
View SolutionIf a line makes angles α,β,γ,δ with the diafonals of a cubes then the value of 9(cos 2alpha + cos 2beta + cos2gamma<br>+ cos 2delta)^(2) equals ….......
View SolutionIf a line makes angle alpha,beta\ and\ gamma with the coordinate axes, find the value of cos2alpha+cos2beta+cos2gamma.
View Solution
