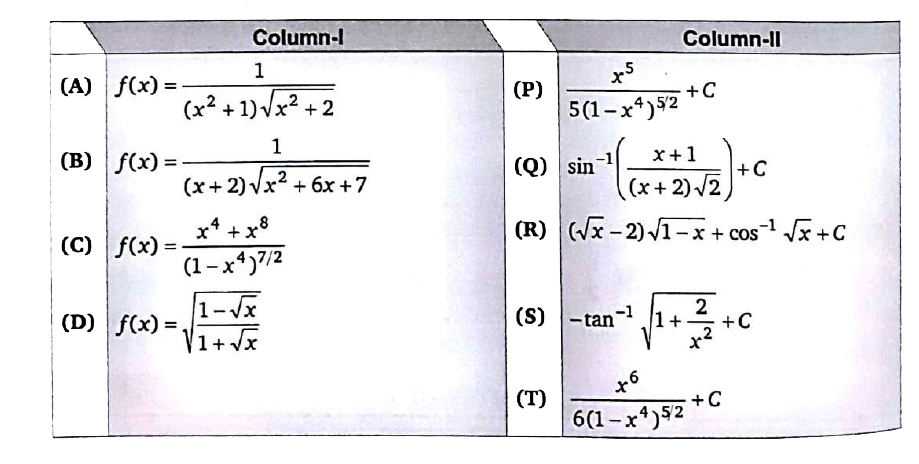
Mathc the following $\int f (x) d x$ is equal to, if

Text Solution

Verified by Experts

The correct Answer is: $A \to S; B \to Q; C \to P; D \to R$

|

Updated on:21/07/2023

Class 12 MATHS INTEGRALS

Topper's Solved these Questions

BOOK - VK JAISWALCHAPTER - INDEFINITE AND DEFINITE INTEGRATIONEXERCISE - EXERCISE (SUBJECTIVE TYPE PROBLEMS)
29videos
INDEFINITE AND DEFINITE INTEGRATION
BOOK - VK JAISWALCHAPTER - INDEFINITE AND DEFINITE INTEGRATIONEXERCISE - EXERCISE (COMPREHENSION TYPE PROBLEMS)
17videos
INDEFINITE AND DEFINITE INTEGRATION
BOOK - VK JAISWALCHAPTER - HYPERBOLAEXERCISE - Exercise-4 : Subjective Type Problems
3videos
HYPERBOLA
BOOK - VK JAISWALCHAPTER - INVERSE TRIGONOMETRIC FUNTIONS EXERCISE - Exercise-5 : Subjective Type Problems
6videos
INVERSE TRIGONOMETRIC FUNTIONS

Similar Questions

Knowledge Check

Question 1 - Select One
$\int_{- a}^{a} f (x) d x$ is equal to
A $\int_{0}^{a} [f (x) + f (- x)] d x$
B $\int_{0}^{a} [f (x) - f (- x)] d x$
C $2 \int_{0}^{a} f (x) d x$
D0
Question 2 - Select One
$\int e^{x} [f (x) + f' (x)] d x =$
A $\int e^{x} f' (x) d x$
B $e^{x} f (x)$
C $e^{x} f' (x)$
DNone of these
Question 3 - Select One
If $\int g (x) d x = g (x)$ , then $\int (f (x) + f' (x)) g (x) d x$ is equal to
A $f (x) g (x)$
B $f' (x) g (x)$
C $(f (x) - f' (x)) g (x)$
D $f (x) g' (x)$

VK JAISWAL-INDEFINITE AND DEFINITE INTEGRATION-EXERCISE (MATCHING TYPE PROBLEMS)

Home
Ask Doubt
Profile