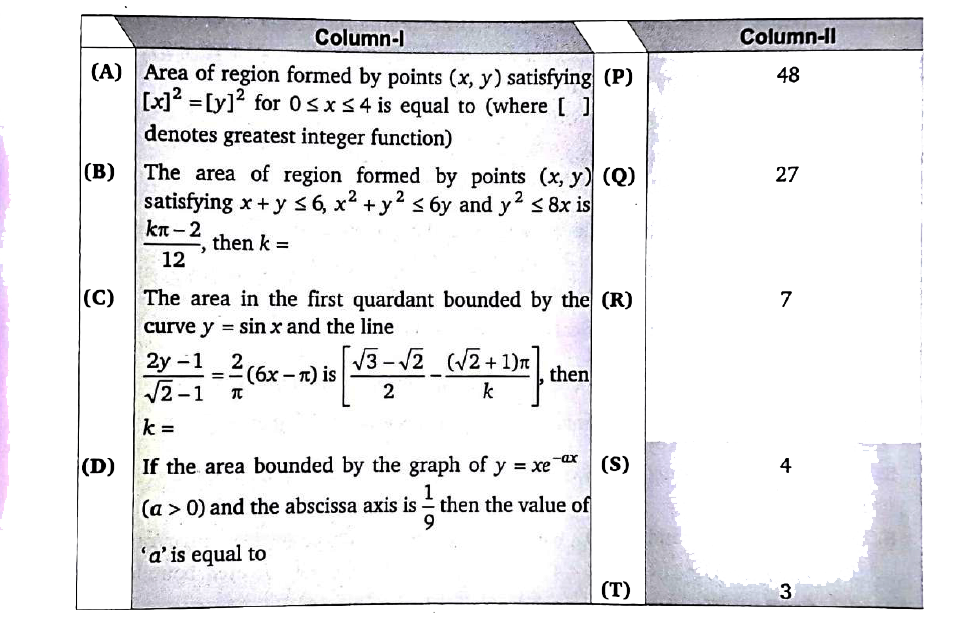
For j=0,1,2…n let Sj be the area of region bounded by the x-axis and the curve yex=sinx for fπ≤x≤(j+1)π
The value of ∞∑j=0Sj equals to :
12(1+ex)
12(1+e−π)
12(1−e−π)
12(eπ−1)
The correct Answer is:B
Step by step video, text & image solution for For j =0,1,2…n let S _(j) be the area of region bounded by the x-axis and the curve ye ^(x)=sin x for f pi le x le (j +1) pi The value of sum _(j =0)^(oo) S_(j) equals to : by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - VK JAISWALCHAPTER - AREA UNDER CURVESEXERCISE - EXERCISE (MATCHING TYPE PROBLEMS)1videos
AREA UNDER CURVES
- BOOK - VK JAISWALCHAPTER - AREA UNDER CURVESEXERCISE - EXERCISE (SUBJECTIVE TYPE PROBLEMS)8videos
AREA UNDER CURVES
- BOOK - VK JAISWALCHAPTER - AREA UNDER CURVESEXERCISE - EXERCISE (ONE OR MORE THAN ONE ANSWER IS/ARE CORRECT)4videos
AREA UNDER CURVES
- BOOK - VK JAISWALCHAPTER - APPLICATION OF DERIVATIVES EXERCISE - EXERCISE (SUBJECTIVE TYPE PROBLEMS)22videos
APPLICATION OF DERIVATIVES
- BOOK - VK JAISWALCHAPTER - BIONMIAL THEOREMEXERCISE - Exercise-4 : Subjective Type Problems15videos
BIONMIAL THEOREM
Similar Questions
View SolutionLet f be a differentiable function satisfying the condition f(xy)=(fx))f(y)(y≠0,f(y)≠0)∀x,y∈Randf'(1)=2. If the smaller area enclosed by y=f(x),x2+y2=2 is A, then findal [A], where [.] represents the greatest integer function.
View SolutionLet f (x) be a polynomial function of degree 3 where a<b<candf(a)=f(b)=f(c). If the graph of f (x) is as shown, which of the following statements are INCORRECT ? (Where c>|a|)
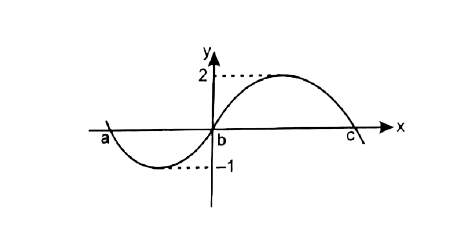15:05View SolutionA conical vessel is to be prepared out of a circular sheet of metal of unit radius in order that the vessel has maximum value, the sectorial area that must be removed from the sheet is A1 and the area of the given sheet is A2, then A2A1 is equal to
View SolutionThe sum of series 3⋅2007C0−8⋅2007C1+13⋅2007C2−18⋅2007C3+…… upto 2008 terms is K, then K is :
05:35View SolutionLet S1=∑∑0≤i<j≤100CiCj, S2=∑∑0≤j<i≤100CiCj and S3=∑∑0≤i=j≤100CiCj where Cr represents cofficient of xr in the binomial expansion of (1+x)100
If S1+S2+S3=ab where a, b∈N, then the least value of (a+b) is08:09View SolutionThe value of the expansion (∑∑)0≤i<j≤n(−1)i+j−1nCi⋅nCj=
03:12View SolutionLet b≠0 and for j=0,1,2,....,n. Let Sj be the area of the region bounded by Y_axis and the curve x⋅eay=sinby,jπb≤y≤(j+1)πb. Show that S0,S1,S2,...Sn are in geometric progression. Also, find their sum for a=-1 and b=π.
View SolutionFor j=0,1,2…n let Sj be the area of region bounded by the x-axis and the curve yex=sinx for fπ≤x≤(j+1)π
The value of So is :02:36View SolutionFor j=0,1,2…n let Sj be the area of region bounded by the x-axis and the curve yex=sinx for jπ≤x≤(j+1)π
The ratio S2009S2010 equals :01:45View SolutionFor j=0,1,2…n let Sj be the area of region bounded by the x-axis and the curve yex=sinx for jπ≤x≤(j+1)π
The value of ∞∑j=0Sj equals to :02:48View Solutionवक्र y=cosx,(0≤x≤π) तथा x -अक्ष से घिरे क्षेत्र का क्षेत्रफल ज्ञात कीजिए ।
03:20View Solutionमाना b≠0,j=0,1,2,.....,n के लिए, यदि Sj,y-अक्ष और वक्र xeay=sinby,jπb≤y≤(j+1)πb द्वारा घिरे क्षेत्र का क्षेत्रफल है। तब दिखाइए कि S0,S1,.....,Sn गुणोत्तर श्रेढ़ी में हैं। a=−1 और b=π के लिए उनका योग भी ज्ञात कीजिए।
09:45View SolutionLet S1=∑∑0≤i<j≤100CiCj, S2=∑∑0≤j<i≤100CiCj and S3=∑∑0≤i=j≤100CiCj where Cr represents cofficient of xr in the binomial expansion of (1+x)100
If S1+S2+S3=ab where a, b∈N, then the least value of (a+b) is05:38View SolutionThe value of the expansion (∑∑)0≤i<j≤n(−1)i+j−1nCi⋅nCj=
11:55View SolutionArea of the region bounded by the curve y=sinxandx -axis −π2≤x≤π2 ………
View Solution