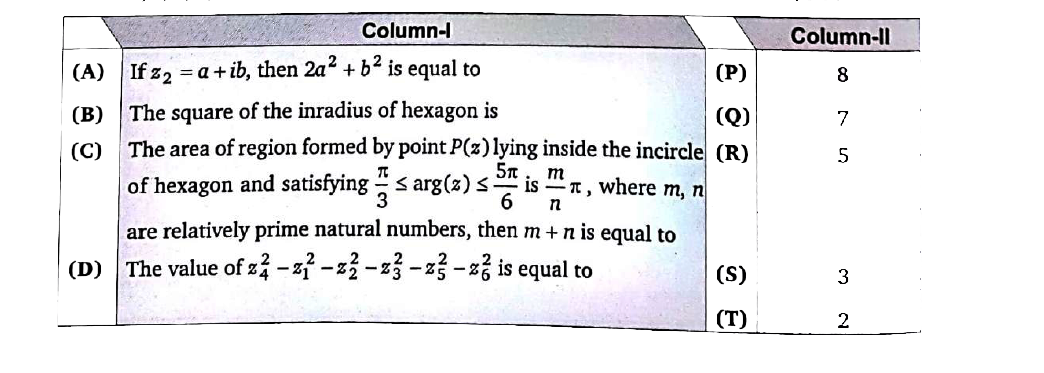
Let ABCDEF is a regular hexagon A(z1),B(z2),C(z3),D(z4),E(z5),F(z6) in argand plane where A,B,C,D,E and F are taken in anticlockwise manner. If z1=−2,z3=1−√3i.
Text Solution
The correct Answer is:A-R, B-S, C-Q,D-P
|
Topper's Solved these Questions
- COMPLEX NUMBERSBOOK - VK JAISWALCHAPTER - COMPLEX NUMBERSEXERCISE - EXERCISE-5 : SUBJECTIVE TYPE PROBLEMS
8 Videos
- COMPLEX NUMBERSBOOK - VK JAISWALCHAPTER - COMPLEX NUMBERSEXERCISE - EXERCISE-3:COMPREHENSION TYPE PROBLEMS
9 Videos
- CIRCLEBOOK - VK JAISWALCHAPTER - CIRCLEEXERCISE - Exercise - 5 : Subjective Type Problems
13 Videos
- COMPOUND ANGLESBOOK - VK JAISWALCHAPTER - COMPOUND ANGLESEXERCISE - Exercise-5 : Subjective Type Problems
31 Videos
Similar Questions
Knowledge Check
if A(z1),B(z2),C(z3),D(z4) lies on |z|=4 (taken in order) , where z1+z2+z3+z4=0 then :
AMax. area of quadrilateral ABCD=32BMax. area of quadrilateral ABCD=16CThe triangle ΔABC is right angledDThe quadrilateral ABCD is rectangleIf z1,z2,z3,z4 are the affixes of the vertices of a parallelogram taken in order in Argand plane, then
Az1+z3=z2+z4Bz1+z2=z3+z4Cz1−z3=z2−z4DNone of theseLet A(z1),B(z2),C(z3) be the vertices of an equilateral triangle ABC in the Argand plane, then the number z2−z32z1−z2−z3, is
Apurely realBpurely imaginaryCa complex number with non-zero and imaginary partsDnone of these
The vertices of a square are z1,z2,z3andz4 taken in the anticlockwise order, then z3=
View SolutionLet z1,z2,z3,z4 be the vertices A,B,C,D respectively of a square on the Argand diagramtaken in anticlockwise direction then prove that: 2z2=(1+i)z1+(1−i)z3
View SolutionA(z1),B(z2),C(z3) are the vertices of he triangle ABC (in anticlockwise). If ∠ABC=π/4 and AB=√2(BC) , then prove that z2=z3+i(z1−z3).
View SolutionA(z1),B(z2),C(z3) are the vertices of the triangle ABC (in anticlockwise). If ∠ABC=π/4 and AB=√2(BC) , then prove that z2=z3+i(z1−z3).
View SolutionLet A(z1),B(z2),C(z3) be the vertices of an equilateral triangle ABC in the Argand plane, then the number z2−z32z1−z2−z3, is
View Solution
