W-{0}=………….Solution in Telugu
R
N
Z
Q
The correct Answer is:B
Step by step video, text & image solution for W-{0}=…………. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SETS BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - SETS EXERCISE - OBSERVATION BITS TO SOLVE VARIOUS QUESTION GIVEN IN THE PUBLIC EXAMINATION
21 Videos
- REAL NUMBERS (MULTIPLE CHOICE QUESTION)BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - REAL NUMBERS (MULTIPLE CHOICE QUESTION)EXERCISE - REAL NUMBERS (MULTIPLE CHOICE QUESTION)
22 Videos
- SIMILAR TRIANGLES BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - SIMILAR TRIANGLES EXERCISE - CREATIVE BITS FOR CCE MODEL EXAMINATION
90 Videos
Similar Questions
Set of human being that reside on moon is ………………..
View Solution915=…………..
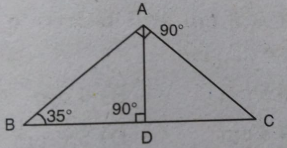
View SolutionFrom the figure ,∠DAC……….
View SolutionIf w is a complex cube root of unity, show that ([[1,w,w^2],[w,w^2,1],[w^2,1,w]]+[[w,w^2,1],[w^2,1,w],[w,w^2,1]])*[[1],[w],[w^2]]=[[0],[0],[0]]
View SolutionShow that ⎛⎜⎝⎡⎢⎣1ωω2ωω21omwga21ω⎤⎥⎦+⎡⎢⎣ωω21ω21ωωω21⎤⎥⎦⎞⎟⎠⎡⎢⎣1ωω2⎤⎥⎦=⎡⎢⎣000⎤⎥⎦
View SolutionIf omega is cube roots of unity, prove that {[(1,omega,omega^2),(omega,omega^2,1),(omega^2,1,omega)]+[(omega,omega^2,1),(omega^2,1,omega),(omega,omega^2,1)]} [(1),(omega),(omega^2)]=[(0),(0),(0)]
View SolutionIf ω=1 is the complex cube root of unity and matrix H=∣∣∣ω00ω∣∣∣, then H70 is equal to:
View SolutionIf A=[ω00ω], where ω is cube root of unity, then what is A100 equal to ?
View Solutionयदि ω, इकाई के एक घनमूल है तो सिद्ध कीजिए की-
⎧⎪⎨⎪⎩⎡⎢⎣1ωω2ωω21ω21ω⎤⎥⎦+⎡⎢⎣ωω21ω21ωωω21⎤⎥⎦⎫⎪⎬⎪⎭⎡⎢⎣1ωω2⎤⎥⎦=⎡⎢⎣000⎤⎥⎦
View Solutionदिखाएँ कि ⎛⎜⎝⎡⎢⎣1ωω2ωω21ω21ω⎤⎥⎦+⎡⎢⎣ωω21ω21ωωω21⎤⎥⎦⎞⎟⎠⎡⎢⎣1ωω2⎤⎥⎦=⎡⎢⎣000⎤⎥⎦
View SolutionIf ω≠1 is the complex root of unity and matrix H=[ω00ω] then H70 is equal to :
View SolutionIf ω is a complex cube root of unity, show that ⎛⎜⎝⎡⎢⎣1ωω2ωω21ω21ω⎤⎥⎦+⎡⎢⎣ωω21ω21ωωω21⎤⎥⎦⎞⎟⎠[1ωω2]=[000]
View SolutionIf omega The complex of the unit is cube root and determinant. H=|(omega,0),(0,omega)| Then H^(70) Is equal to
View SolutionIf ω is a complex cube root of unity and A=[ω00ω] then A100=
View SolutionProve that , {[{:(1,omega,omega^(2)),(omega,omega^(2),1),(omega^(2),1,omega):}]+[{:(omega,omega^(2),1),(omega^(2),1,omega),(omega,omega^(2),1):}]}[{:(1),(omega),(omega^(2)):}]=[{:(0),(0),(0):}] where omega is the cube root of unit.
View Solution
