If a and b are two numbers such that ab = 0 , then (a)a = 0 and b = 0 (b) a = 0 or b = 0 (c) a = 0 and b≠0 (d) b = 0 and a≠0
To solve the problem, we need to analyze the statement that the product of two numbers a and b equals zero, i.e., ab=0.
1. Understanding the Product Zero Condition:
- The equation ab=0 implies that the product of a and b is zero. In mathematics, this condition leads us to conclude that at least one of the multiplicands must be zero.
2. Exploring Cases:
- We can break down the possibilities:
- Case 1: a=0 and b can be any number (including zero).
- Case 2: b=0 and a can be any number (including zero).
- Case 3: Both a=0 and b=0.
3. Analyzing the Options:
- (a) a=0 and b=0: This is one possibility, but not the only one.
- (b) a=0 or b=0: This is true because at least one of them must be zero for their product to be zero.
- (c) a=0 and b≠0: This is a possible scenario but not the only one.
- (d) b=0 and a≠0: This is also a possible scenario but not the only one.
4. Conclusion:
- The only statement that must always be true given ab=0 is that at least one of a or b must be zero. Therefore, the correct answer is:
- (b) a=0 or b=0.
- The equation ab=0 implies that the product of a and b is zero. In mathematics, this condition leads us to conclude that at least one of the multiplicands must be zero.
- We can break down the possibilities:
- Case 1: a=0 and b can be any number (including zero).
- Case 2: b=0 and a can be any number (including zero).
- Case 3: Both a=0 and b=0.
- (a) a=0 and b=0: This is one possibility, but not the only one.
- (b) a=0 or b=0: This is true because at least one of them must be zero for their product to be zero.
- (c) a=0 and b≠0: This is a possible scenario but not the only one.
- (d) b=0 and a≠0: This is also a possible scenario but not the only one.
- The only statement that must always be true given ab=0 is that at least one of a or b must be zero. Therefore, the correct answer is:
- (b) a=0 or b=0.
Topper's Solved these Questions
Similar Questions
If A and B are two events such that P(A∩B)=0.32 and P(B)=.5,thenP(A/B)
View SolutionIf A and B are two events such that P(A)=0.3, P(B)=0.6 and P(B/A) =0.5 find P(A/B) .
View SolutionGiven that A and B are two events such that P:(a)=0.6, P(B)=0.3 and P(A∩B)=0.2, find P(AB)and P(B/A).
View SolutionIf A and B are two independent events such that P(A)=0.3 and P(A∪B')=0.8
Find P(B)
View Solutionif A and B are events such that P(A)=0.6, P(B)=0.3 and P(A∩B)=0.2,find P(A/B)and P(B/A).
View SolutionIf P(A)=0.4, P(B)=0.3 and P(B/A)=0.5, find P(A∩B) and P(A/B).
View SolutionGiven two independent events A and B such that P(A)=0.3 and P(B)=0.6 . Find: P(A∩B)
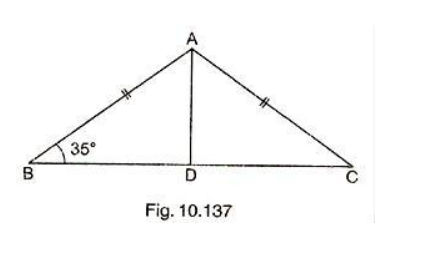
View SolutionABC is an isosceles triangle such that AB=ACandAD is the median to base BC . Then, ∠BAD=
(a) 55∘
(b) 70∘
(c) 35∘
(d) 110∘
View SolutionIf P(A∪B)=0.8 and P(A∩B)=0.3 ,then P(A)+P(B)=
View SolutionIf the points (a, 0), (0, b)and (1, 1) are collinear, prove that a+b=ab.
View Solution
